filmov
tv
Integral battlel#11, the theta world!
Показать описание
Integral battlel#11, the theta world!
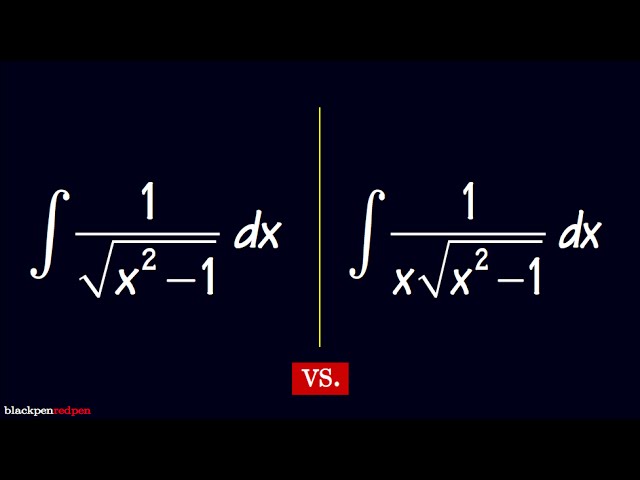
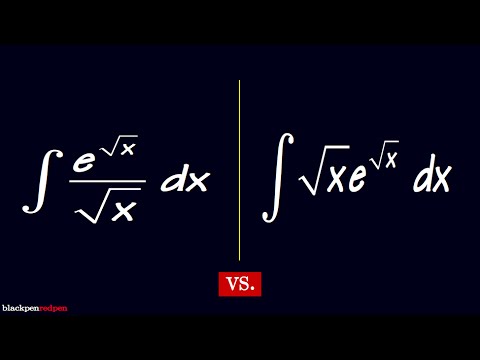
integral of 1/sqrt(x^2-1) vs. integral of 1/(x*sqrt(x^2-1)),
integral of 1/sqrt(x^2-1), integral of 1/(x*sqrt(x^2-1)),
Be sure to subscribe for more,
blackpenredpen
integral of 1/sqrt(x^2-1) vs. integral of 1/(x*sqrt(x^2-1)),
integral of 1/sqrt(x^2-1), integral of 1/(x*sqrt(x^2-1)),
Be sure to subscribe for more,
blackpenredpen
Integral battlel#11, the theta world!
Maths vs Physics
IQ TEST
Integral of 1/(sinh(theta) + cosh(theta))
integral battle#10: what? twins?
fun integral battle#6A: looks different, just off by a constant!
11 years later ❤️ @shrads
Casio 991EX vs. 991ES, which is fast and better? #scientificcalculator #casiocalculator
fun integral battle#7: you might as well do them this way!
integral battle#8: division or multiplication?
integral battle#12, with a pokeball
7th CLASS Student Solving IIT Problems😱🔥#jeewallah #shorts #pw #physicswallah
'So close 🤏🏼,yet so far ♾️' Explanation and code in description #maths #satisfying #adhd #...
integral battle#13, the old, the new!
Integrals | Integral of Sec (theta)| My first Video ♥☺️
Pakistan education system what a beautiful environment WOW🤣🤣
Integral battle#20, hidden u-sub
Steven Hawcking on God
EoTech EXPS3 + G33 #eotech #optic #guns #reddotsight
Very nice integral with hyperbolic trig functions
integral battle#2: a small sign makes a BIG difference!
sec theta = 3
How Psychedelics Change the Brain
The Battle of Reach – Complete Timeline
Комментарии
 0:04:44
0:04:44
 0:00:25
0:00:25
 0:00:29
0:00:29
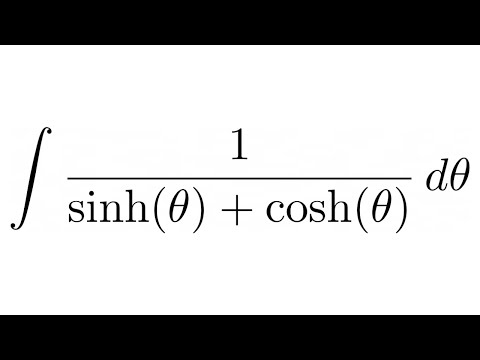 0:02:40
0:02:40
 0:06:38
0:06:38
 0:05:28
0:05:28
 0:00:11
0:00:11
 0:00:22
0:00:22
 0:09:24
0:09:24
 0:08:40
0:08:40
 0:08:23
0:08:23
 0:00:17
0:00:17
 0:01:00
0:01:00
 0:05:49
0:05:49
 0:00:31
0:00:31
 0:00:16
0:00:16
 0:08:15
0:08:15
 0:01:01
0:01:01
 0:00:15
0:00:15
 0:04:41
0:04:41
 0:08:49
0:08:49
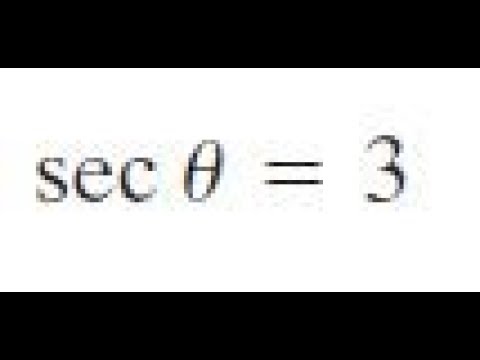 0:00:35
0:00:35
 0:04:12
0:04:12
 0:25:53
0:25:53