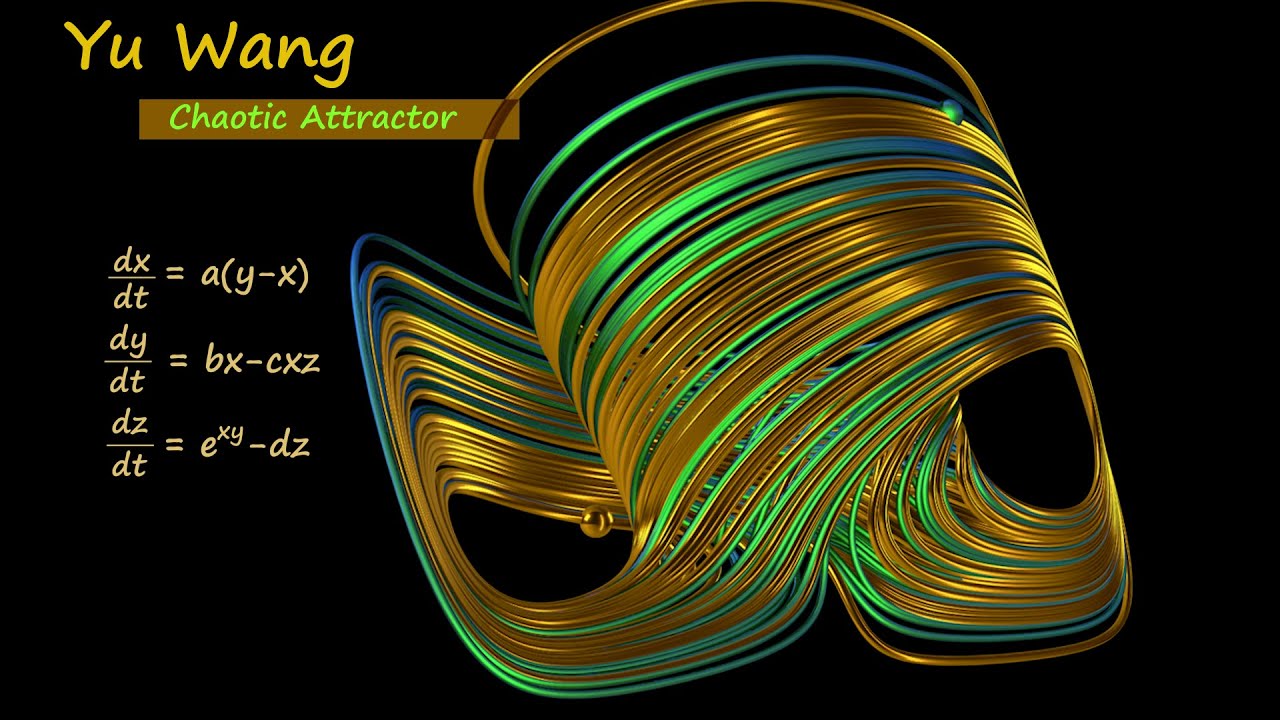
Yu-Wang Attractor| Chaotic attractor | Chaos Theory

This nonlinear system with specific initial conditions is solved
numerically and the resulting trajectory is shown through a 3 dimensional animation.

Initial condition 1: (2.5,2.2,28)
Initial condition 2: (2.2,2.2,28)
Time step: 0.002

"In a chaotic system, the trajectory moves around on the attractor as time goes on, but two
nearby points separate exponentially so that eventually they are very far apart. Although their
future is determined uniquely and precisely by the governing equations, very small differences
in the starting point can make large differences in the future conditions. Although tomorrow’s
weather depends on the conditions today, and the weather the day after tomorrow depends on
the conditions tomorrow, small errors in measuring the current weather eventually grow until
all hope of predictability is lost — the ‘butterfly effect.’ "

#YuWang|#ChaoticSystem #ButterflyEffect| thinkeccel

[dr.myungjunkim]

I have 2 questions.

1. What is your favourite chaotic attractor? And why?

2. What is the most simplest chaotic attractor that requires a very small number of nonlinear terms?

[thinkeccel]

Two neighbouring initial conditions and the resulting trajectories...

[dioneyalfonso]

What do you use to make the animations?