filmov
tv
ICFP 2018 Keynote Address: Conveying the Power of Abstraction

Показать описание
Conveying the Power of Abstraction, presented by Eugenia Cheng
ICFP 2018 Keynote Address: Conveying the Power of Abstraction
ICFP 2018 Keynote Address: Gradual Typing
ICFP 2018 Keynote Address: The Role of Functional Programming and DSLs in Hardware
ICFP 2018: Youth Conference
Lead On Canada at ICFP 2018
Birth of the New Model - WBM Nanovisu~ - ICFP Programming Contest 2018
Session Moderator @ ICFP 2018, Kigali, Rwanda
ICFP 2018 Day 2 Highlights
Keep Your Laziness in Check
2018 ICFP Welcome Video featuring Gates Institute Director Jose 'Oying' Rimon II
ICFP 2018 Program Chair's Report
It Doesn’t End Here: Final Recap Video – International Conference on Family Planning 2018
Report on ICFP and Climate Change
ICFP 2018 Youth Pre conference
Murinzi Alexandre Edgard, Youth Delegate for ICFP 2018, Kigali Rwanda
Investing for a Lifetime of Returns (ICFP 2018) by Muhammad A. Busairi
How to register for 2018 ICFP Youth Pre-conference. Kigali, Rwanda
Typing, Representing, and Abstracting Control: Functional Pearl
Family Planning Superheroes – International Conference on Family Planning 2018
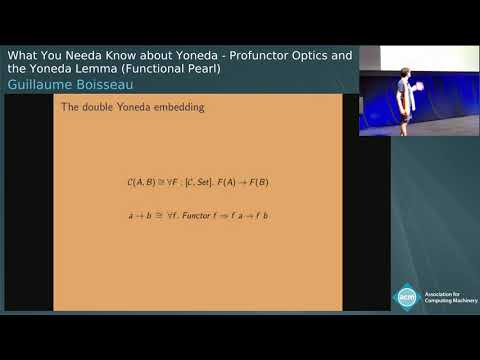
What You Needa Know about Yoneda - Profunctor Optics and the Yoneda Lemma
Keynote: Assuring AI
ICFP Session 5
02 Contributing to GHC
How to Bake Pi
Комментарии
 1:00:30
1:00:30
 1:02:36
1:02:36
 1:01:09
1:01:09
 0:01:00
0:01:00
 0:01:31
0:01:31
 0:02:23
0:02:23
 0:03:31
0:03:31
 0:01:00
0:01:00
 0:22:26
0:22:26
 0:02:42
0:02:42
 0:10:18
0:10:18
 0:06:10
0:06:10
 0:22:44
0:22:44
 0:01:26
0:01:26
 0:04:35
0:04:35
 0:05:00
0:05:00
 0:02:35
0:02:35
 0:20:54
0:20:54
 0:02:38
0:02:38
 0:22:10
0:22:10
 0:53:22
0:53:22
 1:44:02
1:44:02
 0:23:27
0:23:27
 1:02:07
1:02:07