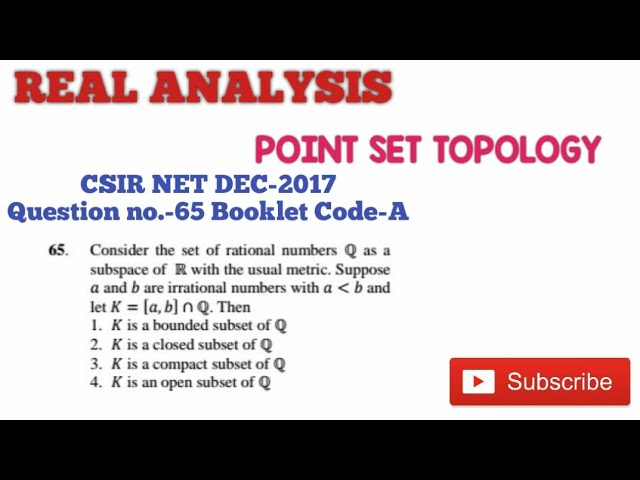
CSIR NET Dec 2017,Q-65 CODE-A || Topology, Real Analysis ||with various concept and approach

Hello Learners,
I have explained in this question so many concept like open set, closed set, compact set in simple way and solved this question by various approach.

at 20:18 u take open cover as arbitrary union(a+1/n,b-1/n)intersection Q which is equivalent to (a,b)intersectionQ .if u suppose finite sub cover then u will get a no {a+1/g:g€N} which is rational belong to K less to { a+1/k:k€N} by using archmedian property which is left end of finite sub cover so not in finite sub cover. so contradict our assumptions that K is compact. u can understand as here I have taken one open cover as arbitrary union of (a+1/n,b-1/n)intersectionQ which has no finite sub cover. So, K is not compact
at24:00 place of e there will be 1/e which is irrational .

For more videos visit my playlist or go through link .

#open set, closed set, limit point(Complex Analysis)

#epsilon delta definition of limit

#epsilon delta definition of continuity

#Stereographic Projection, Complex Analysis

Previous year CSIR NET solution :-

If u like this video please don't forget to like, share and subscribe this channel.
Thanks

#CSIRNETDec2017 #PointsetTopology #RealAnalysis #Sharpenmaths

[neerushimar]

First tym maine aapki video dekhi.... Bht achha explain kr rkha... Thanks for sharing your knowledge

[madhurimamukherjee]

thanks for solving this conceptual problem....
also thanx for using alternative is so helpful

[madhurimamukherjee]

please make some more videos solving questions of 🙏🏻🙏🏻🙏🏻🙏🏻🙏🏻
I have many confusions regarding this topic

[SharpenMaths]

at 20:18 u take open cover as arbitrary union(a+1/n, b-1/n)intersection Q which is equivalent to (a, b)intersectionQ .if u suppose finite sub cover then u will get a no {a+1/g:g€N} which is rational belong to K less to { a+1/k:k€N} by using archmedian property which is left end of finite sub cover so not in finite sub cover. so contradict our assumptions that K is compact. u can understand as here I have taken one open cover as arbitrary union of (a+1/n, b-1/n)intersectionQ which has no finite sub cover. So, K is not compact
at24:00 place of e there will be 1/e which is irrational.

[sripad]

Please use a big white board.If u go back and front it's a bit confusing

[SharpenMaths]

Please share and subscribe this link to who are preparing for CSIR NET GATE.

[laxmividyaclasses]

By hein borel Theorem every closed and bounded set is compact.

[KB-wuxd]

For third option
We should choose U=(a+1/n, b-1/n)

In your choice of U, K is already a subset of every element of ur open cover