Translation and Inverse Laplace Transforms

Показать описание

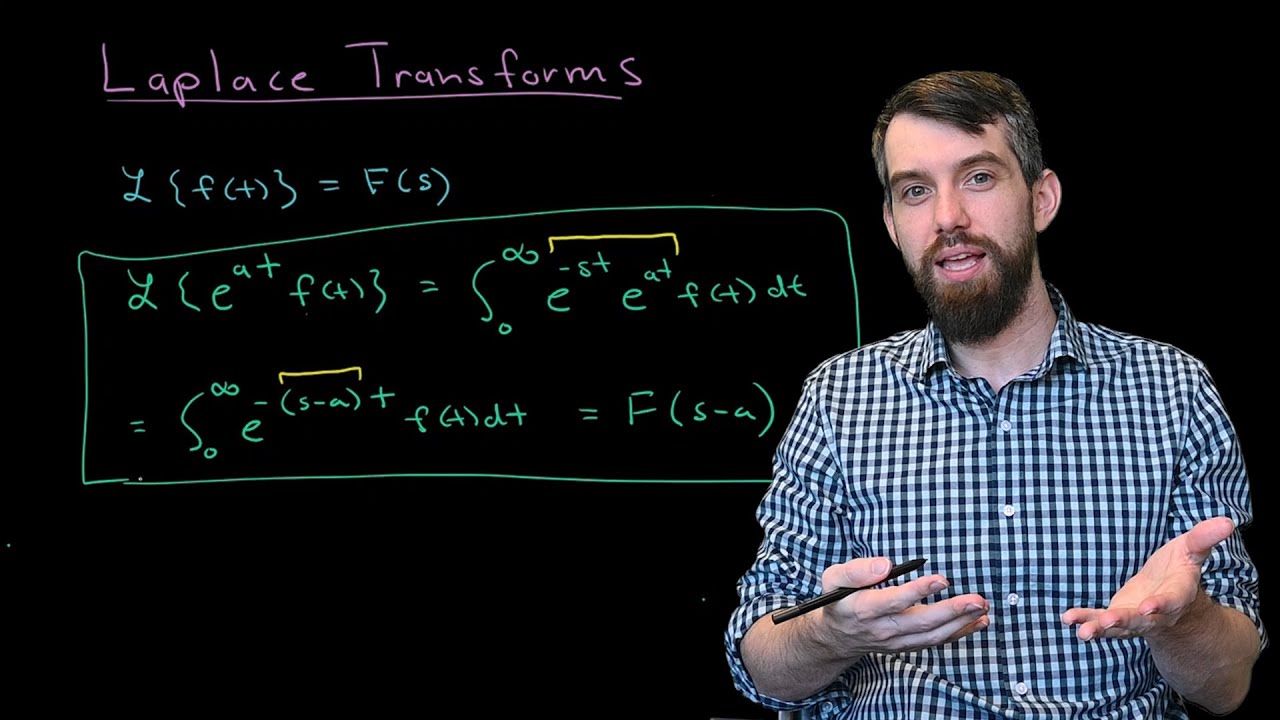
In episode 5 of our series on Laplace Transforms, we compute more Inverse Laplace Transforms. First we introduce a property called the Translation Property which allows us to deal with a horizontal shift. This dramatically increases the set of functions we can compute the inverse laplace transform of. One algebraic trick we deal with in this video is completing the square, which converts and expression to one that more obviously uses the translation property. In the next video we'll use partial fractions to convert rational functions into a form easier to take the Inverse Laplace Transform.

****************************************************
Other Course Playlists:

***************************************************

► Want to learn math effectively? Check out my "Learning Math" Series:

►Want some cool math? Check out my "Cool Math" Series:

****************************************************

*****************************************************

This video was created by Dr. Trefor Bazett. I'm an Assistant Teaching Professor at the University of Victoria.

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

Рекомендации по теме

Комментарии

The entire series on Laplace transform is just exceptional

juhisaxena

thank you very much for the clear explanation. you are the best teacher I have ever seen 💜💜

xcxqdwk

Thank you so much for a clear and precise video!! I’m so lucky to have just discovered your channel and I hope you gain a lot more recognition soon!!

mili

Thanks so much for this really explanatory video! I'm learning the theory behind the Laplace transforms so that I can use it modelling situations on SIMULINK. As an ChemEng student this is a life saver as it really clears things up!

orueom

Thank you very much for your videos. I recall watching your integration ones 1 year ago when the Pandemic began and in the middle of everything I needed to submit an assignment.

ricardobautista-garcia

So far I have a 100 in differential equations because of these videos :D

scottboyer

You're a life saver!! Thanks from Brazil!

caosspearbr

Thank you 😊😊 because of your explanation was able to win a quick solve contest and I got a prize 🏆 😊🥰🔥🔥in Laplace transforms

mileslegend

You just threw the idea of t^2 becoming a cube in the denominator without deriving it, so I had no idea what you were talking about.

I wish you had said more about how the shift applies when you have two functions being multiplied together as in e^t sin(kt).

the_eternal_student

Thank you so much.If teaching with laplace transform table simultaneously is way better . I love your video

Yuen

well done. You have planned this presentation so well.

wunboonail

At 12:00, this is division by 2 not by ½, or multiplication by ½

VeritasEtAequitas

at 11:35 I think you forget to include translation part e^t in second inverse transform
isn't it
Im sorry I didn't see that caption, sorry again :)

ManojKumar-cjoj

Damn these videos are a godsend. My professor sucks... at least the one I have in person ;)

namelecc

i have a question. why di he put (s-1)+1 in the numerator at minute 8:53?

asahbernard

You explained very well, it was very clear and easy to understand, I understand how inverse Lapplace Transform works. But are we going to be asked to show the detailed steps of the process of inverse Laplace Transform? Because you didn't show them in the video, and doing the normal Laplace Transform can show the steps of the integration

yoyochan

Nice one professor plz can you solve laplace-1(ln(1+s))

josephhajj

Hi, Trevor, what tablet do you use to write and share the screen with?

gatesfather

this might be a stupid question but why do we add a e^t in front of both cos(2t) and 1/2 sin(2t)

terramyr

The final answer i thought it could have e^t as a coefficient of cos 2t +(1/2)sin 2t...i need help on this🙏🏾

benetsonichulangula

Translation and Inverse Laplace Transforms

Translation and Inverse Laplace Transforms

Laplace Transforms: The First Translation Theorem || Full Tutorial

How to Use the First Translation Theorem to Find Inverse Laplace Transforms

Laplace Transform: Translation Theorem in s

Inverse laplace transform, translation in s vs translation in t

inverse laplace transform, example#5, with completing the square

Laplace Transform. First Translation Theorem.

Laplace Transform - First Translation Theorem | Math with Janine

Inverse Laplace Transform of (s - 1)/(s + 1)^3 with the First Translation Theorem

Inverse Laplace Transform :: Completing the Square :: First Translation Theorem in Reverse

Inverse Laplace Transform Example using Partial Fractions

Inverse Laplace

Inverse Laplace Transform with unit step function, sect7.6#15

Differential Equations: Examples of inverse Laplace transforms of translations on the s-axis

Laplace Transform of a Piecewise Function (Unit Step Function)

Inverse Laplace Transforms - Full Tutorial

Laplace Transform. Second Translation Theorem.

Laplace Transform: First Shifting Theorem

56. First Translation Theorem, Computing Laplace transforms and inverse Laplace transforms

inverse laplace transform, example#1

How to Use the First Translation Theorem to Find Laplace Transforms

7.3 Laplace transform example using s-translation

Laplace Transform of the product of 't' and some Function(s) | ζ{tⁿf(t)}

2nd shift Theorem Inverse Laplace Transform Example 2