filmov
tv
Linear Approximations and Differentials

Показать описание
Linear Approximation
In this video, I explain the concept of a linear approximation, which is just a way of approximating a function of several variables by its tangent planes, and I illustrate this by approximating complicated numbers f without using a calculator. Enjoy!
In this video, I explain the concept of a linear approximation, which is just a way of approximating a function of several variables by its tangent planes, and I illustrate this by approximating complicated numbers f without using a calculator. Enjoy!
Linear Approximation
Calculus 1: Linear Approximations and Differentials (Video #18) | Math with Professor V
Calculus 3.10 Linear Approximations and Differentials
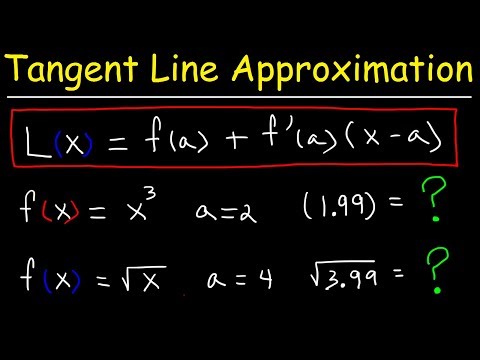
Finding The Linearization of a Function Using Tangent Line Approximations
Linear Approximations and Differentials
Differentials and Derivatives - Local Linearization
Local linearization | Derivative applications | Differential Calculus | Khan Academy
Linear and Differential approximation 3.10 Calculus1
Linear Approximations | Using Tangent Lines to Approximate Functions
Linear Approximation Differential AP Calculus for Exponential Function
Calculus 1 | Linear Approximations and Differentials
Approximation Using Linearization (Calculus)
What is linear approximation?
MAT183 - Differentials & Approximation
Calculus I: Linear approximations and differentials
Calculus I - Linear Approximations and Differentials
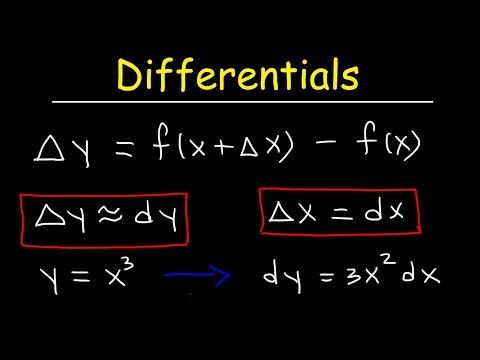
Linear Approximations and Differentials
4.2 Linear Approximations and Differentials
Linear Approximation and Differentials.
Calculus 3 Lecture 13.4: Finding Differentials of Multivariable Functions
Calculus - Linear Approximations & Differentials
Linear Approximation Using Differentials
Linear Approximations and Differentials
MATH 109 - Section 3.10 Linear Approximations and Differentials.
Комментарии
 0:05:31
0:05:31
 0:31:59
0:31:59
 0:23:46
0:23:46
 0:13:12
0:13:12
 0:22:14
0:22:14
 0:10:13
0:10:13
 0:09:38
0:09:38
 0:25:15
0:25:15
 0:09:49
0:09:49
 0:08:57
0:08:57
 0:10:29
0:10:29
 0:10:35
0:10:35
 0:08:57
0:08:57
 0:29:00
0:29:00
 0:42:30
0:42:30
 0:51:27
0:51:27
 0:44:29
0:44:29
 0:28:24
0:28:24
 0:24:54
0:24:54
 1:51:04
1:51:04
 0:36:00
0:36:00
 0:06:12
0:06:12
 2:28:40
2:28:40
 0:27:03
0:27:03