Surface of Revolution - Best ever Maths Lecture - By Danish

preview_player
Показать описание
A surface of revolution is a surface in Euclidean space created by rotating a curve (the generatrix) around an axis of rotation.

Examples of surfaces of revolution generated by a straight line are cylindrical and conical surfaces depending on whether or not the line is parallel to the axis. A circle that is rotated around any diameter generates a sphere of which it is then a great circle, and if the circle is rotated around an axis that does not intersect the interior of a circle, then it generates a torus which does not intersect itself (a ring torus).
  1. EDUMORF INFOTAINMENT
  2. edumorf infotainment
  3. edumorf
  4. reddy kumara simha
  5. ankush
  6. startup
Рекомендации по теме
Surface of Revolution 0:14:22

Surface of Revolution - Best ever Maths Lecture - By Danish

Surface Area of 0:30:36

Surface Area of Revolution By Integration Explained, Calculus Problems, Integral Formula, Examples

Torus: a surface 0:01:05

Torus: a surface of revolution

Surfaces of Revolution 0:06:28

Surfaces of Revolution

Parameterization of surface 0:11:57

Parameterization of surface of revolution

Understanding Areas of 0:03:20

Understanding Areas of Surfaces of Revolution

Meshes in 5 0:04:28

Meshes in 5 minutes: creating a surface of revolution

Parameterization of Surface 0:13:13

Parameterization of Surface of Revolution

Equation of a 0:05:43

Equation of a surface of revolution in 3D

Calculus II: Surface 0:13:16

Calculus II: Surface of revolution

Surface Area of 0:19:47

Surface Area of Revolution of Parametric Equations: X-axis & Y-axis

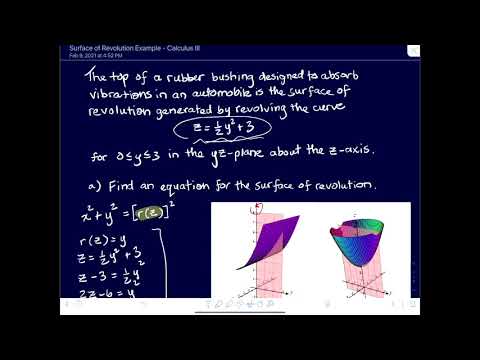
Surface of Revolution 0:26:54

Surface of Revolution Example - Calculus III

Surface Area of 0:06:22

Surface Area of a Solid of Revolution - Q4

Surface of Revolution 0:12:58

Surface of Revolution - Equation and Examples

Surface of Revolution 0:05:40

Surface of Revolution

surfaces of revolution 0:18:09

surfaces of revolution

Area of a 0:21:40

Area of a Surface of Revolution

Surface Area of 0:09:41

Surface Area of a Solid of Revolution using Integration with an Example.

Vectors: Space Coordinates 0:05:52

Vectors: Space Coordinates - 10. Surfaces of Revolution

Arc Length & 0:17:35

Arc Length & Surface Area examples

Area of surfaces 0:33:06

Area of surfaces of Revolution (8.2)

Area of Surface 0:44:20

Area of Surface of Revolution

Surface of revolution 0:08:09

Surface of revolution of curve

[Math 402] 15. 0:22:28

[Math 402] 15. Surface of Revolution

Комментарии
Автор

if xy=2, rotate about y=-x from x=√2 to 2 find volume

عطاالدهامشة-عح