filmov
tv
Proof: a³ - a is always divisible by 6 (2 of 2: Proof by exhaustion)

Показать описание
Proof: a³ - a is always divisible by 6 (1 of 2: Two different approaches)
Proof: a³ - a is always divisible by 6 (2 of 2: Proof by exhaustion)
a³+b³=? , a³-b³=?
How to derive identity a³+b³ 💁👌😍 A cube + b cube (a3+b3)
How to derive identity a³-b³ 💁👌😍 A cube - b cube (a3-b3)
a³+b³=(a+b)(a²-ab+b²) Proof | a Cube plus b Cube Identity Proof
a3+b3+c3-3abc all formula|a3 b3 c3 3abc formula|a3 + b3 + c3 - 3abc ka sutra|a3+b3+c3-3abc factorise
FORMULA PROVED a3 - b3 FOR CLASS IX.
If (a / b + b / a) = - 1 then find a³ - b³. Math class -9th. Symmetry Education #math
Verify Algebraic Identity a3 - b3 Formula Proof || Maths Activity, Project, TLM
Oppo A3 Pro 5g 🔥🔥💯 || Camera And Zoom test 📸 #oppo #oppoa3pro #shortvideo
Proof of (a+b)3=a3+3a2b+3ab2+b3 || Proof of (a+b)3 formula
#a³+b³ #a³-b³ #algebraicformula | a³+b³ | a³+b³ | Algebraic Formula | Algebraic Identity | Identity...
a³-b³=(a-b)(a²+ab+b²) Proof || a Cube minus b Cube Identity Proof
Prove that : a3 + b3 = (a+b) (a2-ab+b2).
REDMI A3 vs IPHONE 11 PRO BOOTUP TEST
Verify Algebraic Identity a3 + b3 Formula Proof || Maths Activity, Project, TLM
VERIFY THE IDENTITY (a³ - b³) = (a - b) (a² + ab + b²) #viral #shorts #shortvideo #short #education...
a3 + b3 + c3 - 3abc = ? (A Cube+ B Cube + C Cube- 3abc ) Formula of a cube plus b cube plus c cube...
Redmi Note 12 Vs Redmi A3 Camera Test 🤜📸🤛 #shorts #redminote12 #redmia3 #comparison #xiaomi
prove that a³ + b³+ c³ - 3abc = ( a + b + c) ( a² + b² + c ² - ab - bc - ca )
a3+b3 formula
Prove that : a3 - b3 = (a-b) (a2+ab+b2).
Manual VS Automatic Audi A3
Комментарии
 0:10:15
0:10:15
 0:15:06
0:15:06
 0:00:24
0:00:24
 0:04:54
0:04:54
 0:05:25
0:05:25
 0:01:26
0:01:26
 0:00:20
0:00:20
 0:01:17
0:01:17
 0:01:28
0:01:28
 0:11:59
0:11:59
 0:00:21
0:00:21
 0:01:48
0:01:48
 0:00:30
0:00:30
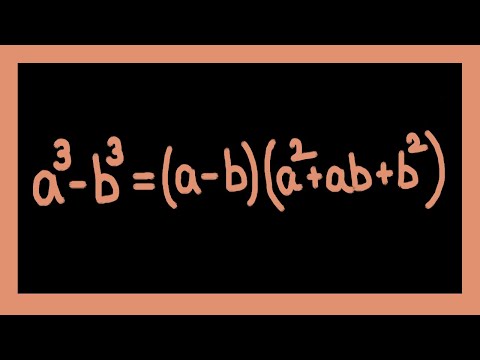 0:01:14
0:01:14
 0:02:20
0:02:20
 0:00:19
0:00:19
 0:12:29
0:12:29
 0:00:50
0:00:50
 0:00:15
0:00:15
 0:00:22
0:00:22
 0:07:55
0:07:55
 0:04:05
0:04:05
 0:02:07
0:02:07
 0:00:31
0:00:31