filmov
tv
Density of an irregular object | Density of an Irregular shaped object floating on water - Kisembo A

Показать описание
In this video, we get to learn about the density of an irregular shaped object. For those who keep funding me via
How to find density of an irregular shaped object / solid which floats in water - Kisembo Academy.
#RelativeDensityOfFloatingSolid #RelativeDensity
transcript;
0:00:00.030,0:00:04.319
in this session I'm going to describe an
0:00:01.949,0:00:06.899
experiment that we can use to determine
0:00:04.319,0:00:13.650
the density of an irregular solid which
0:00:06.899,0:00:16.170
floats in water now the overall mind
0:00:13.650,0:00:17.310
behind this experiment is simply this we
0:00:16.170,0:00:19.500
are going to first get the relative
0:00:17.310,0:00:20.789
density of a object and then after
0:00:19.500,0:00:22.320
Density of an irregular object
getting the relative density of the
0:00:20.789,0:00:24.630
object we are going to go ahead and
0:00:22.320,0:00:25.830
multiply it by the density of water when
0:00:24.630,0:00:28.170
we get the relative density of the
0:00:25.830,0:00:30.240
object multiply that by the density of
0:00:28.170,0:00:32.850
water we will be able to get the density
0:00:30.240,0:00:35.219
of that regular object that we are
0:00:32.850,0:00:37.410
talking about just like though we've
0:00:35.219,0:00:40.770
been doing in our previous sessions in
0:00:37.410,0:00:42.870
the calculations so right before us we
0:00:40.770,0:00:45.870
are having this is called a spring
0:00:42.870,0:00:48.600
balance and we have definitely tied
0:00:45.870,0:00:50.760
something on it this is the solid that
0:00:48.600,0:00:54.570
floats in water the regular solid that
0:00:50.760,0:00:57.180
flow the irregular solid that floats in
0:00:54.570,0:00:59.309
water that whose density we want so as
0:00:57.180,0:01:02.280
you go ahead to find the density first
0:00:59.309,0:01:04.320
of all we are going to put a thread we
0:01:02.280,0:01:07.049
are going to tie a thread to this solid
0:01:04.320,0:01:09.360
and get the weight of this irregular
0:01:07.049,0:01:12.450
solid in air and we are going to call
0:01:09.360,0:01:15.150
that w1 that's our first step since this
0:01:12.450,0:01:16.680
solid but we needed to first get fully
0:01:15.150,0:01:19.080
immersed in water so it means we are
0:01:16.680,0:01:21.780
going to tie a sinker to this solid so
0:01:19.080,0:01:24.119
that it sinks inside now that we have
0:01:21.780,0:01:26.280
attached a sinker to this solid now
0:01:24.119,0:01:28.470
before we actually put this into water
0:01:26.280,0:01:30.270
we need to first get the weight of these
0:01:28.470,0:01:32.280
two so what we do is that we get the
0:01:30.270,0:01:34.860
weight of the solid and the sinker and
0:01:32.280,0:01:36.780
recall that w2 we get them when they are
0:01:34.860,0:01:39.659
still outside before we sink them in
0:01:36.780,0:01:42.150
water we've read we take the reading on
0:01:39.659,0:01:45.299
the spring balance and we determine the
0:01:42.150,0:01:47.420
weight of the solid at the sinker after
0:01:45.299,0:01:49.110
determining the weight of the solid and
0:01:47.420,0:01:51.780
the sinker
0:01:49.110,0:01:53.790
we now emerged baath of them in water
0:01:51.780,0:01:55.920
and when we must both of them in water
0:01:53.790,0:01:58.170
of course this upthrust that is going to
0:01:55.920,0:02:01.020
act on them so meaning there's going to
0:01:58.170,0:02:02.430
be a drop in the reading here so we are
0:02:01.020,0:02:05.130
able to determine the weight of the
0:02:02.430,0:02:07.310
stone and the sinker when they are both
0:02:05.130,0:02:09.200
immersed in water and we call that
0:02:07.310,0:02:12.110
w3
0:02:09.200,0:02:16.130
then afterwards we are going to take
0:02:12.110,0:02:18.920
away the regular solid that sinks in
0:02:16.130,0:02:19.880
that floats on water then we get the
0:02:18.920,0:02:22.2
#Physicsmaths
#Physicsmaths
How to find density of an irregular shaped object / solid which floats in water - Kisembo Academy.
#RelativeDensityOfFloatingSolid #RelativeDensity
transcript;
0:00:00.030,0:00:04.319
in this session I'm going to describe an
0:00:01.949,0:00:06.899
experiment that we can use to determine
0:00:04.319,0:00:13.650
the density of an irregular solid which
0:00:06.899,0:00:16.170
floats in water now the overall mind
0:00:13.650,0:00:17.310
behind this experiment is simply this we
0:00:16.170,0:00:19.500
are going to first get the relative
0:00:17.310,0:00:20.789
density of a object and then after
0:00:19.500,0:00:22.320
Density of an irregular object
getting the relative density of the
0:00:20.789,0:00:24.630
object we are going to go ahead and
0:00:22.320,0:00:25.830
multiply it by the density of water when
0:00:24.630,0:00:28.170
we get the relative density of the
0:00:25.830,0:00:30.240
object multiply that by the density of
0:00:28.170,0:00:32.850
water we will be able to get the density
0:00:30.240,0:00:35.219
of that regular object that we are
0:00:32.850,0:00:37.410
talking about just like though we've
0:00:35.219,0:00:40.770
been doing in our previous sessions in
0:00:37.410,0:00:42.870
the calculations so right before us we
0:00:40.770,0:00:45.870
are having this is called a spring
0:00:42.870,0:00:48.600
balance and we have definitely tied
0:00:45.870,0:00:50.760
something on it this is the solid that
0:00:48.600,0:00:54.570
floats in water the regular solid that
0:00:50.760,0:00:57.180
flow the irregular solid that floats in
0:00:54.570,0:00:59.309
water that whose density we want so as
0:00:57.180,0:01:02.280
you go ahead to find the density first
0:00:59.309,0:01:04.320
of all we are going to put a thread we
0:01:02.280,0:01:07.049
are going to tie a thread to this solid
0:01:04.320,0:01:09.360
and get the weight of this irregular
0:01:07.049,0:01:12.450
solid in air and we are going to call
0:01:09.360,0:01:15.150
that w1 that's our first step since this
0:01:12.450,0:01:16.680
solid but we needed to first get fully
0:01:15.150,0:01:19.080
immersed in water so it means we are
0:01:16.680,0:01:21.780
going to tie a sinker to this solid so
0:01:19.080,0:01:24.119
that it sinks inside now that we have
0:01:21.780,0:01:26.280
attached a sinker to this solid now
0:01:24.119,0:01:28.470
before we actually put this into water
0:01:26.280,0:01:30.270
we need to first get the weight of these
0:01:28.470,0:01:32.280
two so what we do is that we get the
0:01:30.270,0:01:34.860
weight of the solid and the sinker and
0:01:32.280,0:01:36.780
recall that w2 we get them when they are
0:01:34.860,0:01:39.659
still outside before we sink them in
0:01:36.780,0:01:42.150
water we've read we take the reading on
0:01:39.659,0:01:45.299
the spring balance and we determine the
0:01:42.150,0:01:47.420
weight of the solid at the sinker after
0:01:45.299,0:01:49.110
determining the weight of the solid and
0:01:47.420,0:01:51.780
the sinker
0:01:49.110,0:01:53.790
we now emerged baath of them in water
0:01:51.780,0:01:55.920
and when we must both of them in water
0:01:53.790,0:01:58.170
of course this upthrust that is going to
0:01:55.920,0:02:01.020
act on them so meaning there's going to
0:01:58.170,0:02:02.430
be a drop in the reading here so we are
0:02:01.020,0:02:05.130
able to determine the weight of the
0:02:02.430,0:02:07.310
stone and the sinker when they are both
0:02:05.130,0:02:09.200
immersed in water and we call that
0:02:07.310,0:02:12.110
w3
0:02:09.200,0:02:16.130
then afterwards we are going to take
0:02:12.110,0:02:18.920
away the regular solid that sinks in
0:02:16.130,0:02:19.880
that floats on water then we get the
0:02:18.920,0:02:22.2
#Physicsmaths
#Physicsmaths
 0:01:28
0:01:28
 0:03:50
0:03:50
 0:02:25
0:02:25
 0:01:19
0:01:19
 0:05:07
0:05:07
 0:01:34
0:01:34
 0:05:43
0:05:43
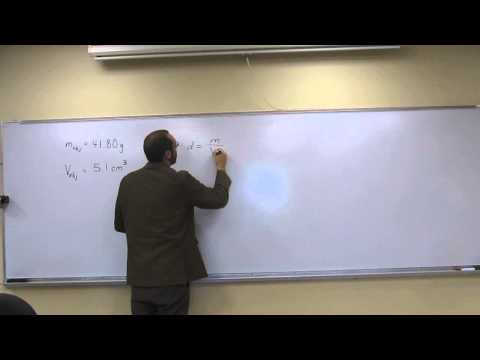 0:02:06
0:02:06
 0:01:13
0:01:13
 0:05:59
0:05:59
 0:08:22
0:08:22
 0:06:19
0:06:19
 0:02:45
0:02:45
 0:03:20
0:03:20
 0:03:42
0:03:42
 0:06:15
0:06:15
 0:00:23
0:00:23
 0:05:32
0:05:32
 0:03:48
0:03:48
 0:03:45
0:03:45
 0:20:53
0:20:53
 0:10:01
0:10:01
 0:01:31
0:01:31
 0:03:36
0:03:36