filmov
tv
Image and Inverse Image of a Normal Subgroup is Normal - Chapter 8 - Lecture 7

Показать описание
We prove that if there is a group homomorphism from one group to another, then the homomorphic image of a normal subgroup in the domain is a normal subgroup of the range of the function. We also prove that the inverse image of a normal subgroup in the codomain is a normal subgroup of the domain.
Link to the previous lecture
Link to the next lecture
Link to the first lecture of this chapter
Link to the previous lecture
Link to the next lecture
Link to the first lecture of this chapter
 0:13:21
0:13:21
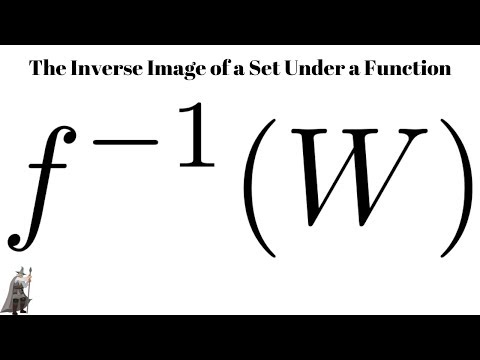 0:06:52
0:06:52
 0:16:43
0:16:43
 0:04:21
0:04:21
 0:43:15
0:43:15
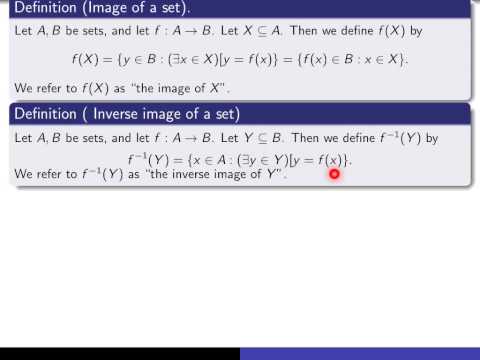 0:06:07
0:06:07
 0:03:56
0:03:56
 0:29:54
0:29:54
 0:15:30
0:15:30
 0:02:15
0:02:15
 0:24:25
0:24:25
 0:03:43
0:03:43
 0:06:22
0:06:22
 0:02:04
0:02:04
 0:07:48
0:07:48
 0:05:39
0:05:39
 0:04:56
0:04:56
 0:02:17
0:02:17
 0:21:27
0:21:27
 0:19:34
0:19:34
 0:06:34
0:06:34
 0:02:30
0:02:30
 0:06:14
0:06:14
 0:13:50
0:13:50