filmov
tv
Inverse Image(Preimage) of Intersection of Sets Proof

Показать описание
Inverse Image(Preimage) of Intersection of Sets Proof. Given a function from X to Y and subsets A and B of Y, in this video we prove that f^(-1)(A n B) = f^(-1)(A) n f^(-1)(B). For a subset A of Y, inverse image or preimage of A under f is the set of all elements of X which get mapped to A; i .e., f^(-1)(A) = {x in X | f(x) in A}. I hope this video helps.
Inverse Image(Preimage) of Intersection of Sets Proof
Preimage(Inverse Image) Explanation and Intersection of Sets Proof
Inverse Image(Preimage) of Union of Sets Proof and Explanation
01 intersections of images and preimages of functions
Inverse Image of Intersection of Two Sets
Images and Preimages of set intersections and unions
Section 4.5, part 4 Inverse image of unions and of intersections
Section 4.5, part 5 Image of unions and of intersections
Inverse Images of Sets Contained in Sets | Set Theory
The Inverse Image of a Set Under a Function: Definition and Examples
Functions (inverses and preimages/inverse images)
Start Learning Sets 5 | Range, Image and Preimage
Preimage of Unions
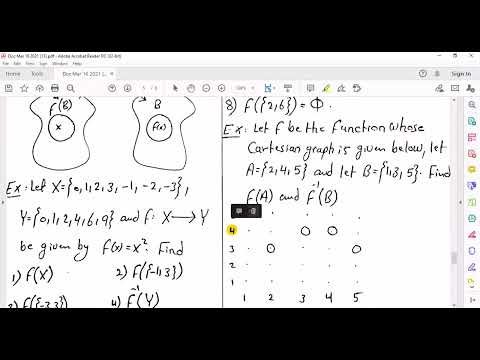
Section 4.5, part 3 Example involving inverse image
Lecture 42 - Image Set and Inverse Image
Properties of the Inverse Image of a Function on Sets: Practice With Proof
What is the image and preimage of a function and how to write it
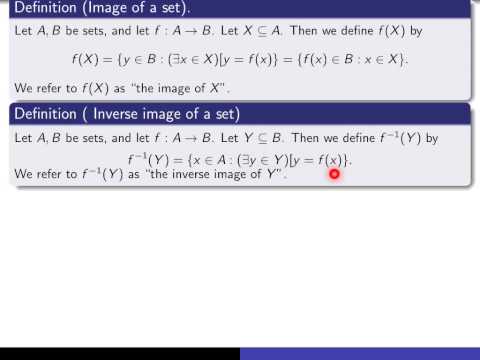
Images and Inverse Images of Sets
Direct Images and Inverse Images--- Problem 1.2.1
Interactions between function images and preimages
Images, Inverse Images, Coimages of Set Maps
Section 4.5, part 1 Image and inverse image of sets
Lecture 21 - Inverse Image of some sets
Images and Preimages of Subsets
Комментарии
 0:05:39
0:05:39
 0:03:56
0:03:56
 0:04:21
0:04:21
 0:07:04
0:07:04
 0:01:46
0:01:46
 0:20:07
0:20:07
 0:07:40
0:07:40
 0:06:56
0:06:56
 0:04:06
0:04:06
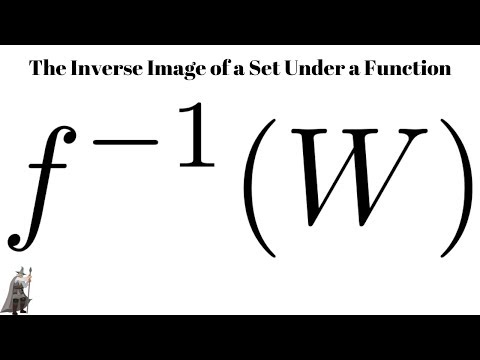 0:06:52
0:06:52
 0:13:54
0:13:54
 0:09:54
0:09:54
 0:04:10
0:04:10
 0:06:08
0:06:08
 0:43:15
0:43:15
 0:29:54
0:29:54
 0:02:15
0:02:15
 0:22:09
0:22:09
 0:21:27
0:21:27
 0:11:21
0:11:21
 0:02:59
0:02:59
 0:06:07
0:06:07
 0:22:10
0:22:10
 0:03:24
0:03:24