filmov
tv
Complex analysis: Cauchy's theorem

Показать описание
This is a lecture recorded by Prof. Steven Strogatz for his undergraduate class on "Applied Complex Analysis" at Cornell University. In this lecture, Prof. Strogatz walks the students through a magnificent proof of one of the main results of complex analysis, known as Cauchy's theorem. (It's also sometimes called Cauchy's integral theorem, or the Cauchy-Goursat theorem, since the proof shown here is due to Goursat.)
Complex Analysis 23 | Cauchy's theorem
Complex Analysis L10: Cauchy Integral Formula
Complex analysis: Cauchy's theorem
Complex integration, Cauchy and residue theorems | Essence of Complex Analysis #6
Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula
Complex Analysis 25 | Cauchy's Theorem (general version)
Complex Analysis | Unit 2 | Lecture 11 | Cauchy Goursat Theorem
Complex Analysis 27 | Cauchy's Integral Formula
Taylor's Theorem | Maclaurin's Theorem | Rolle's Theorem | Lagrange's Theorem | ...
Cauchy's Residue Theorem with Examples | Complex Integration | Complex Analysis #16
Cauchy Integral Formula with Examples - Complex Analysis by a Physicist
Complex Analysis 34 | Residue theorem
Cauchy's Integral Formula For Analytic Function | Example & Solution
# cauchy integral theorem # mathematical physics
Cauchy's Integral Formula with Examples | Complex Integration | Complex Analysis #14
Complex Analysis 22 | Goursat's Theorem
Basic Complex Analysis - Unit 3 - Lecture 4 - Cauchy's Residue Theorem
Complex Analysis | Unit 2 | Lecture 12 | Cauchy Integral Formula
Complex Analysis - 38: Global Version of Cauchy' s Theorem -1
Cauchy integral formula proof. #Complex Analysis
Complex Analysis Mcq-Cauchy's Integral formula -Pgtrb-polytechnic trb -scert lecturers-csir net...
Cauchy’s Theorem (Complex Analysis)
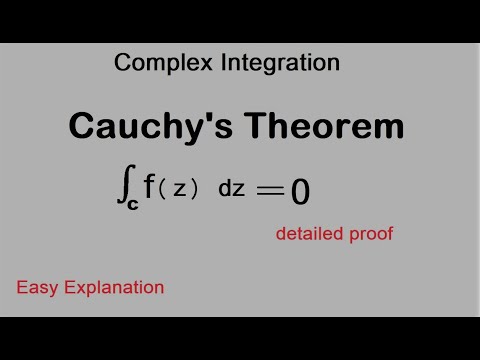
Complex Integration| Cauchy's Theorem| Theta Classes
Cauchy Integral Theorem
Комментарии
 0:09:52
0:09:52
 0:16:43
0:16:43
 0:54:16
0:54:16
 0:40:45
0:40:45
 0:09:05
0:09:05
 0:11:24
0:11:24
 0:03:48
0:03:48
 0:10:55
0:10:55
 0:40:11
0:40:11
 0:10:16
0:10:16
 0:09:27
0:09:27
 0:08:26
0:08:26
 0:20:42
0:20:42
 0:00:15
0:00:15
 0:13:35
0:13:35
 0:13:50
0:13:50
 0:01:55
0:01:55
 0:06:36
0:06:36
 0:27:11
0:27:11
 0:00:35
0:00:35
 0:00:55
0:00:55
 0:14:49
0:14:49
 0:13:57
0:13:57
 0:09:39
0:09:39