Show f(z)={x³(1+i)-y³(1-i)}/(x² + y²) ,f(0) = 0 continuous & C-R eq are satisfy yet f'(0) not exist.

Complex Analysis Theorem from Analytic function
Statement/Theorem /Prove that :-

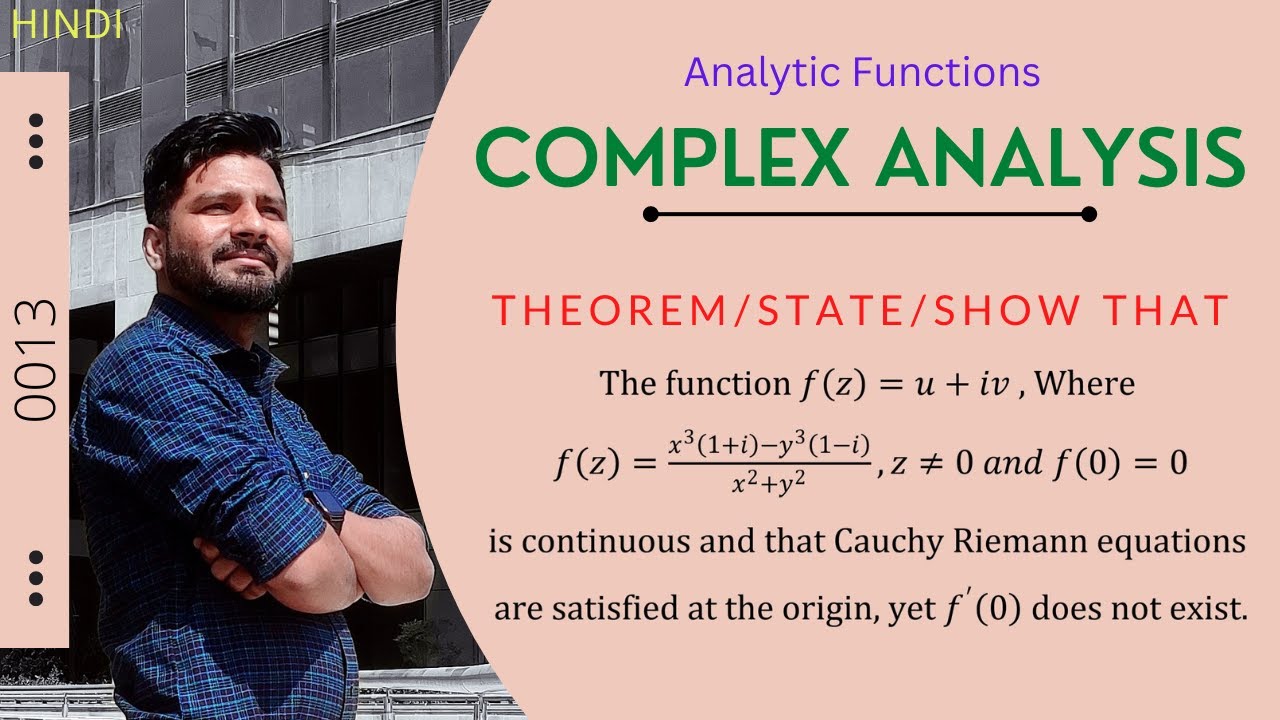
Show that the function f(z) = u+iv, where
f(z) = {x³(1+i)-y³(1-i)}/(x² + y²) , z ≠ 0 and f(0) = 0
is continuous and that Cauchy-Riemann equations are satisfited at the origin, yet f'(0) does not exist.

Solution/ Proof:-

We have

f(z) = {x³(1+i)-y³(1-i)}/(x² + y²)
⇒u+iv = {(x³-y³)+i(x³+y³)}/(x² + y²)
⇒u= (x³-y³)/(x² + y²) and v=(x³+y³)/(x² + y²)
By definition of Continuity

lim z→z₀ f(z) = lim z→z₀ {x³(1+i)-y³(1-i)}/(x² + y²)
lim z→z₀ f(z) = lim ₓ→ₓ₀ , ᵧ→ᵧ₀ {x³(1+i)-y³(1-i)}/(x² + y²)
lim z→z₀ f(z) = {x₀³(1+i)-y₀³(1-i)}/(x₀² + y₀²)

lim z→z₀ f(z) = f(z₀)

Hence f(z) is continuous.

Now at origin (0,0)

(∂u/∂x)= lim ₓ→₀ {u(x,0)-u(0,0)}/x
= lim ₓ→₀ (x³/x²)/x
= lim ₓ→₀ x³/x³
= 1

(∂u/∂y)= lim ᵧ→₀ {u(0,y)-u(0,0)}/y
= lim ᵧ→₀ (-y³/y²)/y
= lim ᵧ→₀ (-y³/y³)
= -1

(∂v/∂x)= lim ₓ→₀ {v(x,0)-v(0,0)}/x
= lim ₓ→₀ (x³/x²)/x
= lim ₓ→₀ x³/x³
= 1

(∂v/∂y)= lim ᵧ→₀ {v(0,y)-v(0,0)}/y
= lim ᵧ→₀ (y³/y²)/y
= lim ᵧ→₀ y³/y³
= 1
Here
(∂v/∂x)=(∂v/∂y) and (∂v/∂x)=-(∂u/∂y)
∴ u and v satisfy Cauchy-Riemann equations at origin.

Now for existence of differentiability

f'(0)=lim z→o {f(z)-f(0)}/(z-0)
f'(0)=lim z→o {(x³-y³)+i(x³+y³)}/{(x² + y²)(x+iy)}

Along real axis
z=x , y=0 ⇒z→0 ∴ x→0

f'(0)=lim ₓ→₀ {(x³-0)+i(x³+0)}/x(x² +0)
=lim ₓ→₀ (x³+ix³)/x³
=(1+i)

Along imaginary axis
z=iy , x=0 ⇒z→0 ∴ y→0

f'(0)=lim ᵧ→₀ {(0-y³)+i(0+y³)}/(iy)(0 + y²)
=lim ₓ→₀ (-y³+iy³)/iy³
=(-1+i)/i
=(1+i)

Along a line curve y=x

f'(0)= lim z→o {(x³-x³)+i(x³+x³)}/{(x² + x²)(x+ix)}
f'(0)= lim z→o (0+i2x³)/{2x³(1+i)}
f'(0)= lim z→o (i2x³)/{2x³(1+i)}
f'(0)= i/(1+i)
f'(0)= (i+1)/2

This shows that f'(0) has different values Along difference curve
∴ f'(z) is not unique , Hence f'(0) does not exist.

Hence Proved...!!!
.
.
.
.
.
.
Kindly join us for CSIR-UGC National Eligibility Test (NET) for Junior Research Fellowship and Lecturer-ship COMMON SYLLABUS FOR PART 'B' AND 'C' MATHEMATICAL SCIENCE
.
.
.
.
.
All BSc, B.Tech, MSc, MA Mathematics students join with us A great platform to get knowledge in Mathematics and science... Stay tuned....for detailed videos on whole SYLLABUS with topic wise...
.
.
.
.
.
.
.
#complexanalysis #csirnet #analyticfunction
.
.
Videos links 🔥🔥🔥

Play List ( Complex Analysis)

[yadav]

Prove that the function f(z)={ 𝑥
3(1+𝑖)−𝑦
3(1−𝑖)
𝑥
2+𝑦2
, (z≠ 0)
0, (z= 0) is continuous and the cauchys-riemann equations are
satisfied at the origin .if f
′(0)does not exist.

[divyasati]

Or dimak kharab ho gya khali chap rkha rt rkha smjh me to kuch nhi aara