filmov
tv
Prob & Stats, Lec 19B: Binomial Distribution Method of Moments Estimator for p (M.O.M. Estimator)

Показать описание
What is the Method of Moments (M.O.M) estimator for the parameter p of a Binomial distribution B(n,p)? We use the fact that the mean (expected value) of X ∼ B(n,p) is μ = E[X] = np. On each side of this equation relating parameters, we replace them with their estimators: X̄ = M1 = np̂. Then we solve for p̂ to get p̂ = X̄/n. If the random sample X1, X2,..., Xm has sample size m, this can be written as p̂ = sum(Xi)/(mn). We can also say p̂ is an unbiased estimator for p. That is, the expected value E[p̂]=p (the expected value of the statistic equals the parameter value.
Links and resources
===============================
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Links and resources
===============================
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
 0:05:29
0:05:29
 0:31:23
0:31:23
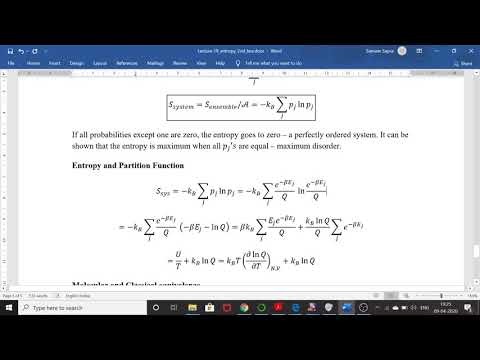 0:19:25
0:19:25
 0:10:01
0:10:01
 1:00:32
1:00:32
 0:30:12
0:30:12
 0:33:26
0:33:26
 0:30:47
0:30:47
 0:25:59
0:25:59
 0:24:34
0:24:34
 0:12:19
0:12:19
 0:02:38
0:02:38
 0:06:41
0:06:41
 0:10:43
0:10:43
 0:01:04
0:01:04
 0:20:25
0:20:25
 0:06:50
0:06:50
 0:53:30
0:53:30
 0:15:16
0:15:16
 0:14:03
0:14:03
 1:17:07
1:17:07
 0:08:54
0:08:54
 0:35:39
0:35:39
 0:05:20
0:05:20