filmov
tv
Antiderivatives and indefinite integrals, pt. 4: integrals of exponential functions.
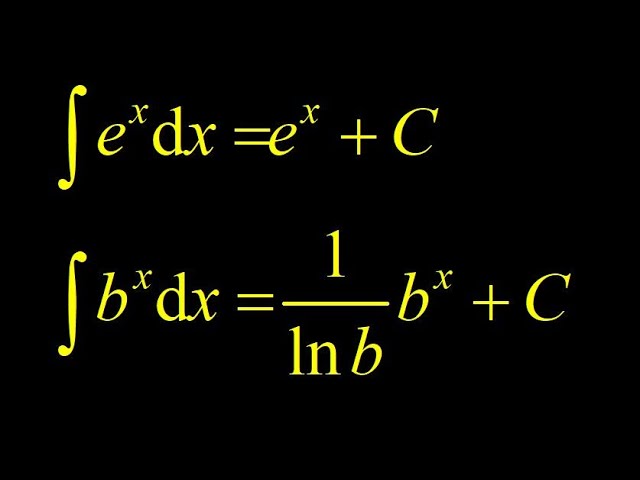
Показать описание
Part of a short series on antiderivatives and indefinite integrals:
Part 1: basic definitions, linear combinations, examples
Part 2: families of curves, ODEs, IVPs, 1D kinematics
Part 3: power rule for antiderivatives, and the antiderivative of 1/x
Part 4: integrals of exponential functions
Part 5: antiderivatives resulting in basic trig functions
Part 6: integrals of tangent and cotangent
Part 7: integrals of secant and cosecant
00:00 Introduction to antiderivatives and indefinite integrals, pt. 4: exponential functions. We start with the simplest case of finding the antiderivative of the natural exponential function e^x. When computing integrals of exponential functions, we know there will be an additive arbitrary constant, and we are trying to guess the essential piece of the antiderivative, which is an unknown function of x. To gain insight on the indefinite integral, we look at the derivative behavior of the natural exponential: e^x is the unique nontrivial function equal to its own derivative. This means the antiderivative of e^x is e^x, since we can see at a glance that differentiating the proposed antiderivative yields the correct result.
00:49 Derivatives of exponentials with a nonstandard base, b: we extend our discussion of antiderivatives of exponentials to the case of a nonstandard base, or a base not necessarily equal to e. We want the indefinite integral of b^x, and we start by investigating the derivative of b^x. The derivative of b^x is lnb*b^x, so an extra factor of lnb is generated when we differentiate. To guess the antiderivative of b^x then, we have the essential piece: b^x. If we differentiate this proposed antiderivative, we miss by a factor of lnb, so all we have to do to correctly construct the antiderivative is supply a 1/lnb in front of b^x, and the derivative of 1/lnb*b^x now gives us back the original function b^x.
01:57 Examples of antiderivatives/indefinite integrals of exponential functions:
1. Find two functions f(x) such that f'(x)=5e^x. We use the formula for the integral of an exponential to find the general antiderivative of 5e^x, then we simply choose two different arbitrary constants to find two unique functions satisfying the differential equation.
2. Compute the indefinite integral of 3^x dx. We simply apply the formula for the integral of an exponential function with a nonstandard base, then we take the time to verify that the derivative of the antiderivative gives back the original function.
3. Compute the indefinite integral of 2^x+3e^x-5/x dx. In this cumulative example, we have the integral of an exponential function with a base not equal to e, the integral of a natural exponential, and the integral of a 1/x term, yielding a natural log function.
Part 1: basic definitions, linear combinations, examples
Part 2: families of curves, ODEs, IVPs, 1D kinematics
Part 3: power rule for antiderivatives, and the antiderivative of 1/x
Part 4: integrals of exponential functions
Part 5: antiderivatives resulting in basic trig functions
Part 6: integrals of tangent and cotangent
Part 7: integrals of secant and cosecant
00:00 Introduction to antiderivatives and indefinite integrals, pt. 4: exponential functions. We start with the simplest case of finding the antiderivative of the natural exponential function e^x. When computing integrals of exponential functions, we know there will be an additive arbitrary constant, and we are trying to guess the essential piece of the antiderivative, which is an unknown function of x. To gain insight on the indefinite integral, we look at the derivative behavior of the natural exponential: e^x is the unique nontrivial function equal to its own derivative. This means the antiderivative of e^x is e^x, since we can see at a glance that differentiating the proposed antiderivative yields the correct result.
00:49 Derivatives of exponentials with a nonstandard base, b: we extend our discussion of antiderivatives of exponentials to the case of a nonstandard base, or a base not necessarily equal to e. We want the indefinite integral of b^x, and we start by investigating the derivative of b^x. The derivative of b^x is lnb*b^x, so an extra factor of lnb is generated when we differentiate. To guess the antiderivative of b^x then, we have the essential piece: b^x. If we differentiate this proposed antiderivative, we miss by a factor of lnb, so all we have to do to correctly construct the antiderivative is supply a 1/lnb in front of b^x, and the derivative of 1/lnb*b^x now gives us back the original function b^x.
01:57 Examples of antiderivatives/indefinite integrals of exponential functions:
1. Find two functions f(x) such that f'(x)=5e^x. We use the formula for the integral of an exponential to find the general antiderivative of 5e^x, then we simply choose two different arbitrary constants to find two unique functions satisfying the differential equation.
2. Compute the indefinite integral of 3^x dx. We simply apply the formula for the integral of an exponential function with a nonstandard base, then we take the time to verify that the derivative of the antiderivative gives back the original function.
3. Compute the indefinite integral of 2^x+3e^x-5/x dx. In this cumulative example, we have the integral of an exponential function with a base not equal to e, the integral of a natural exponential, and the integral of a 1/x term, yielding a natural log function.
 0:05:37
0:05:37
 0:04:21
0:04:21
 0:03:29
0:03:29
 0:09:10
0:09:10
 0:12:39
0:12:39
 0:03:39
0:03:39
 0:10:53
0:10:53
 0:07:11
0:07:11
 0:06:00
0:06:00
 0:13:43
0:13:43
 0:02:00
0:02:00
 0:00:13
0:00:13
![Indefinite integral[Integration]](https://i.ytimg.com/vi/O-yEtadwzPM/hqdefault.jpg) 0:04:07
0:04:07
 0:00:44
0:00:44
 0:06:37
0:06:37
 0:01:07
0:01:07
 0:00:43
0:00:43
 0:00:13
0:00:13
 0:00:55
0:00:55
 0:07:26
0:07:26
 0:00:43
0:00:43
 0:07:53
0:07:53
 0:00:55
0:00:55
 0:11:19
0:11:19