filmov
tv
Finding the end behavior of a polynomial to the fifth degree

Показать описание
👉 Learn how to determine the end behavior of the graph of a polynomial function. To do this we will first need to make sure we have the polynomial in standard form with descending powers. We will then identify the leading terms so that we can identify the leading coefficient and degree of the polynomial.
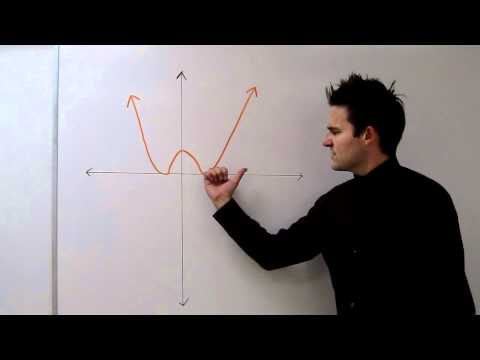
The end behavior of a polynomial depends on the leading coefficient (the coefficient of the term with the greatest power) and the degree (the exponent of the term with the greatest power) of the polynomial. If the degree is even and the leading coefficient is positive, the graph of the polynomial rises left and rises right. If the degree is even and the leading coefficient is negative, the graph of the polynomial falls left and falls right. If the degree is odd and the leading coefficient is positive, the graph of the polynomial falls left and rises right. If the degree is odd and the leading coefficient is negative, the graph of the polynomial rises left and falls right.
Organized Videos:
✅End Behavior of Polynomial Functions
✅How to Find the End Behavior From an Equation
✅How to Find the End Behavior From a Graph
✅End Behavior | Learn About
✅How to Find the End Behavior From Factors
Connect with me:
#polynomials #brianmclogan
The end behavior of a polynomial depends on the leading coefficient (the coefficient of the term with the greatest power) and the degree (the exponent of the term with the greatest power) of the polynomial. If the degree is even and the leading coefficient is positive, the graph of the polynomial rises left and rises right. If the degree is even and the leading coefficient is negative, the graph of the polynomial falls left and falls right. If the degree is odd and the leading coefficient is positive, the graph of the polynomial falls left and rises right. If the degree is odd and the leading coefficient is negative, the graph of the polynomial rises left and falls right.
Organized Videos:
✅End Behavior of Polynomial Functions
✅How to Find the End Behavior From an Equation
✅How to Find the End Behavior From a Graph
✅End Behavior | Learn About
✅How to Find the End Behavior From Factors
Connect with me:
#polynomials #brianmclogan
Комментарии
 0:04:24
0:04:24
 0:04:05
0:04:05
 0:00:54
0:00:54
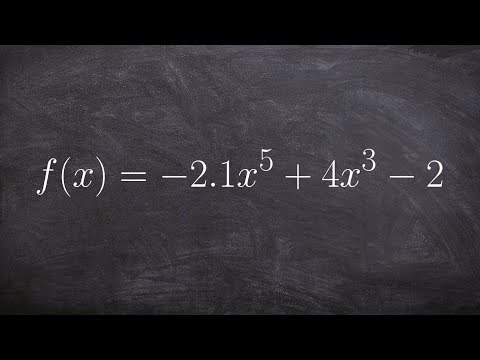 0:02:25
0:02:25
 0:20:28
0:20:28
 0:02:36
0:02:36
 0:06:00
0:06:00
 0:00:13
0:00:13
 0:07:49
0:07:49
 0:07:09
0:07:09
 0:01:54
0:01:54
 0:00:48
0:00:48
 0:07:13
0:07:13
 0:04:16
0:04:16
 0:10:24
0:10:24
 0:03:33
0:03:33
 0:03:28
0:03:28
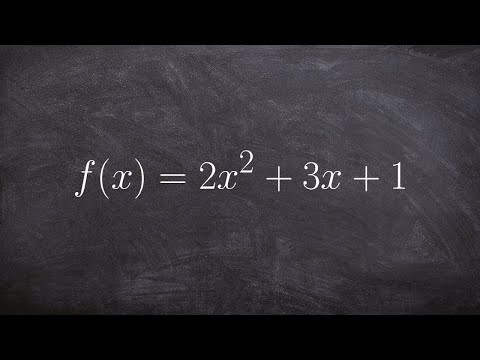 0:03:11
0:03:11
 0:03:23
0:03:23
 0:03:02
0:03:02
 0:03:50
0:03:50
 0:10:06
0:10:06
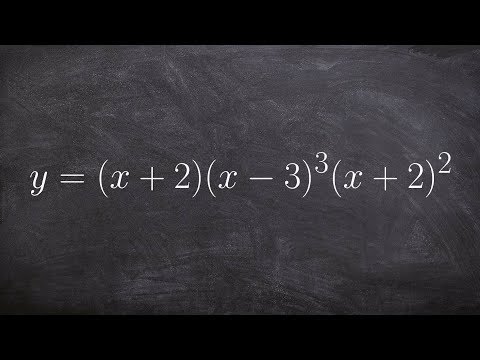 0:02:26
0:02:26
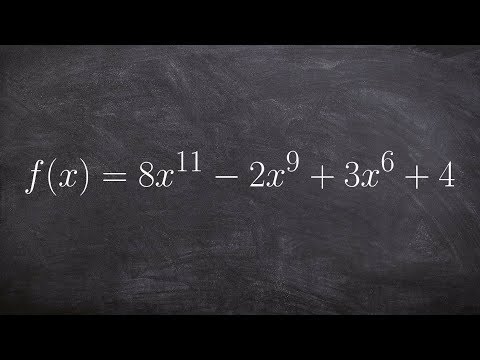 0:02:16
0:02:16