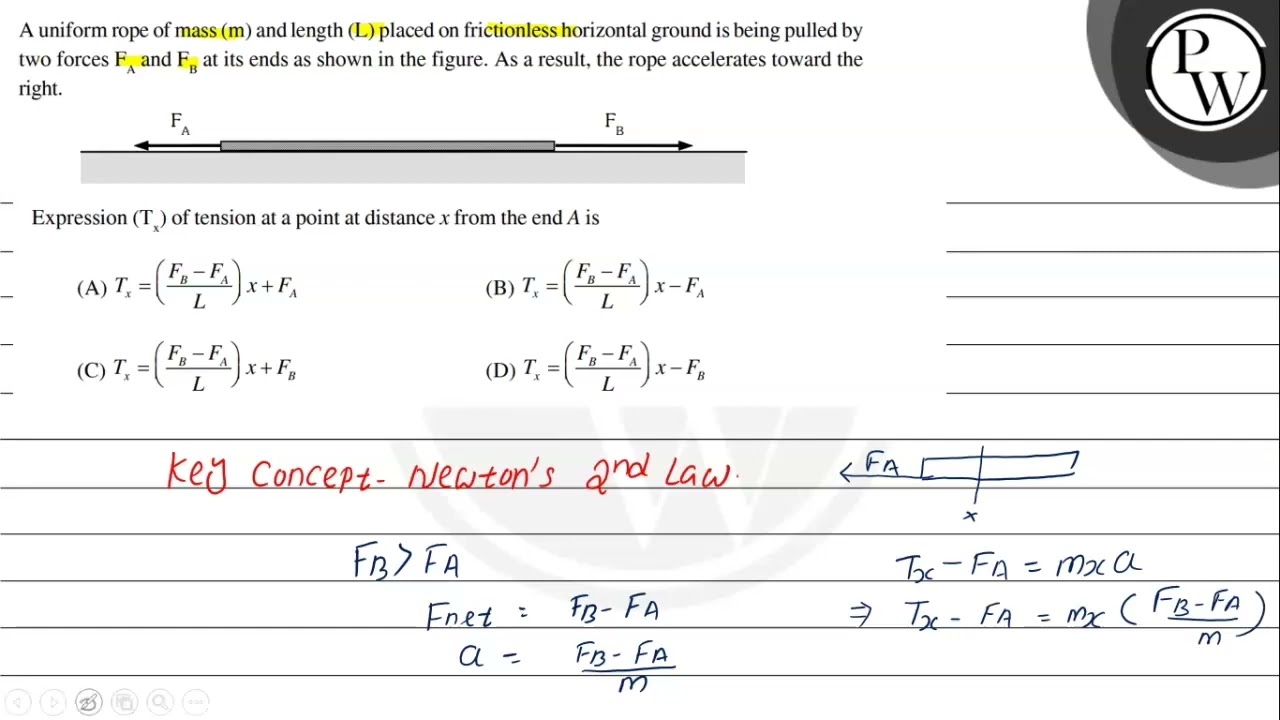
A uniform rope of mass \( (\mathrm{m}) \) and length \( (\mathrm{L}...

A uniform rope of mass \( (\mathrm{m}) \) and length \( (\mathrm{L}) \) placed on frictionless horizontal ground is being pulled by two forces \( \mathrm{F}_{\mathrm{A}} \) and \( \mathrm{F}_{\mathrm{B}} \) at its ends as shown in the figure. As a result, the rope accelerates toward the right.
Expression \( \left(\mathrm{T}_{\mathrm{x}}\right) \) of tension at a point at distance \( x \) from the end \( A \) is
(A) \( T_{x}=\left(\frac{F_{B}-F_{A}}{L}\right) x+F_{A} \)
(B) \( T_{x}=\left(\frac{F_{B}-F_{A}}{L}\right) x-F_{A} \)
(C) \( T_{x}=\left(\frac{F_{B}-F_{A}}{L}\right) x+F_{B} \)
(D) \( T_{x}=\left(\frac{F_{B}-F_{A}}{L}\right) x-F_{B} \)