filmov
tv
A riccati differential equation
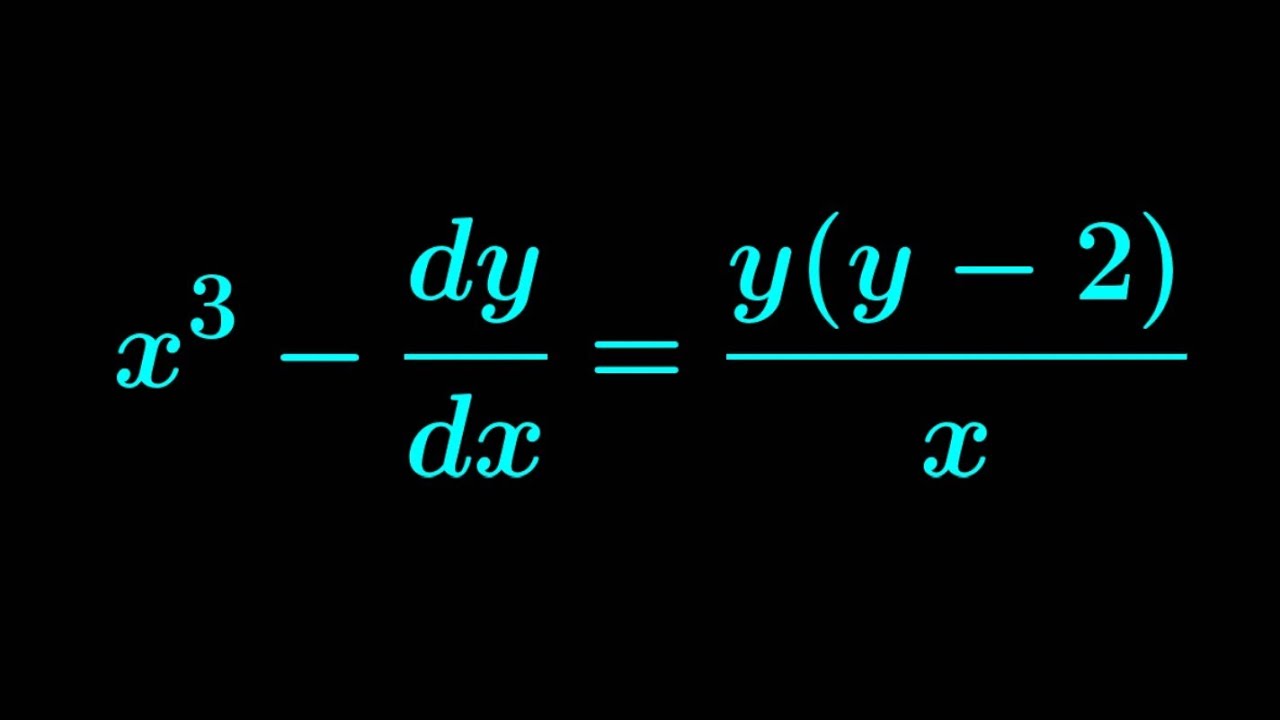
Показать описание
Here's an example on how to solve a class of differential equations called ricatti equations.
My complex analysis lectures:
If you like the videos and would like to support the channel:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
My complex analysis lectures:
If you like the videos and would like to support the channel:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
A riccati differential equation
Riccati Differential Equations: Solution Method
🔵17 - Ricatti Differential Equation 1 - (dy/dx + p(x)y = r(x)y^2 + q(x))
Riccati Differential Equation Example
How to solve differential equations
Riccati Differential Equation
Why the Riccati Equation Is important for LQR Control
Riccati ode
Solving a Riccati Differential Equation
Riccati Differential Equation - Differential Equations
Riccati Differential Equations | Concept with Examples
Riccati Differential Equation: Solution Methods
Lecture 10 - Riccati Equation | Ordinary Differential Equations
24 First-Order Differential Equations
This is why you're learning differential equations
Riccati Ordinary Differential Equations | Lecture 9
Differential Equations: Riccati Equations | Example Problem #1
🔴 11 - DIFFERENTIAL EQUATION - How to Solve Riccati and Bernouli with fx199ES
Bernoulli’s solution of Riccati Differential Equation
Riccati Differential Equation | Riccati Equation 1 | Riccati Equation Lesson | Riccati
Solving a Riccati Equation
How to Solve Bernoulli Differential Equations (Differential Equations 23)
(DE03) The Bernoulli and Riccati Equations
Riccati Equation and some special cases and its solution
Комментарии
 0:08:48
0:08:48
 0:11:04
0:11:04
 0:17:46
0:17:46
 0:16:46
0:16:46
 0:00:46
0:00:46
 0:20:24
0:20:24
 0:14:30
0:14:30
 0:08:21
0:08:21
 0:09:30
0:09:30
 0:40:16
0:40:16
 0:38:38
0:38:38
 0:49:14
0:49:14
 0:13:57
0:13:57
 4:56:20
4:56:20
 0:18:36
0:18:36
 0:20:02
0:20:02
 0:01:06
0:01:06
 0:05:42
0:05:42
 0:11:03
0:11:03
 0:18:01
0:18:01
 0:34:56
0:34:56
 1:43:35
1:43:35
 0:32:32
0:32:32
 0:24:40
0:24:40