filmov
tv
Laplace equation
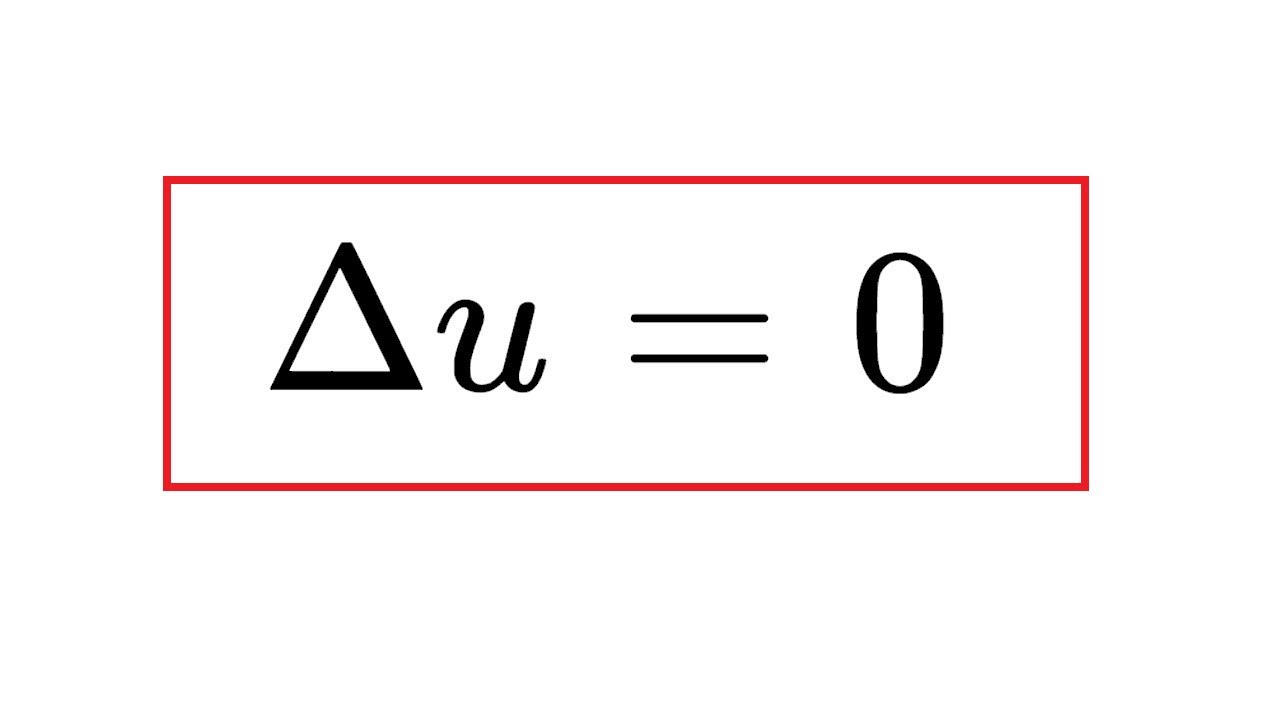
Показать описание
By popular demand, here’s a video of me solving a PDE! Here I find a nontrivial solution of Laplace’s equation Delta u = 0 by turning it into an ODE. At the same time I’m finding a formula of the derivative of |x| in multiple dimensions. Enjoy!
Laplace Equation
Laplace's Equation and Poisson's Equation
Laplace's Equation and Potential Flow
Laplacian intuition
Poisson's Equation and Laplace's Equation
Partial Differential Equations - 5.9 - Laplace's Equation - Part 1 of 2
What does the Laplace Transform really tell us? A visual explanation (plus applications)
Poisson's and Laplace Equations: Derivations and Explanations
PDE 101: Separation of Variables! ...or how I learned to stop worrying and solve Laplace's equa...
Laplace Equation | Separation of Variables Method in PDE | Example & Concepts by GP Sir
Laplace equation
ECE221: Laplace's Equation and Poisson's Equation
LAPLACE EQUATION WITH EXAMPLE PROBLEM ||M3||OU EDUCATION
Poisson's Equation for Beginners: LET THERE BE GRAVITY and How It's Used in Physics | Part...
Derivation and Solution of Laplace’s Equation
Young Laplace Equation
How to solve differential equations
Fourier Series Solution of Laplace's Equation
2.3.3 Poisson's Equation and Laplace's Equation
3.1.1 Introduction to Laplace's Equation
Solution of Laplace Equation with 9 mesh squares || Numerical Methoda || Dr Prashant Patil
Laplace equation
Complex Analysis L07: Analytic Functions Solve Laplace's Equation
solve differential with laplace transform, sect 7.5#3
Комментарии
 0:13:17
0:13:17
 0:17:55
0:17:55
 0:15:32
0:15:32
 0:05:31
0:05:31
 0:09:59
0:09:59
 0:18:02
0:18:02
 0:20:25
0:20:25
 0:12:19
0:12:19
 0:49:54
0:49:54
 0:24:38
0:24:38
 0:24:58
0:24:58
 0:12:42
0:12:42
 0:07:18
0:07:18
 0:12:12
0:12:12
 0:33:40
0:33:40
 0:14:08
0:14:08
 0:00:46
0:00:46
 0:14:04
0:14:04
 0:02:23
0:02:23
 0:04:15
0:04:15
 0:11:18
0:11:18
 0:16:46
0:16:46
 0:41:15
0:41:15
 0:07:52
0:07:52