filmov
tv
Phase space & Liouville's Theorem
Показать описание
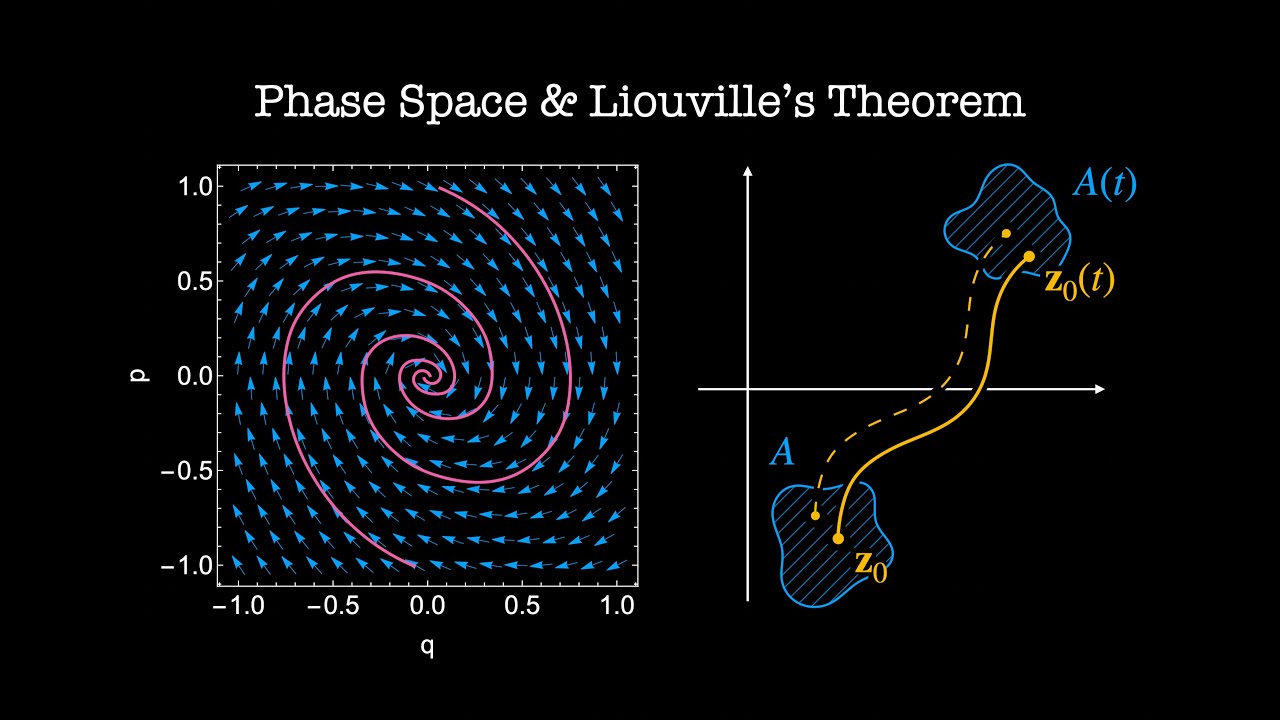
Hamiltonian dynamics exists in phase space -- a space of formed of all the generalized positions and generalized momenta. We explore ways to solve Hamilton's equations in this space.
Music "Everything" by Vi Hart
Music "Everything" by Vi Hart
Phase space & Liouville's Theorem
Liouville and Noether's theorem | phase space, conservation laws and symetries
Phase space and Liouville's theorem
Classical Mechanics, Lecture 19: Hamiltonian Evolution on Phase Space. Liouville's Theorem.
Liouville's theorem
Liouville's Theorem through Symplectic Geometry
Mathematical proof of Liouville theorem
liouville s theorem |phase space statistical mechanics lecture
Liouville's Theorem and phase space
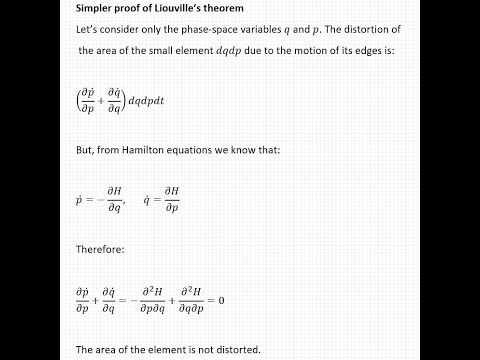
Simple proof of Liouville's theorem
Liouville's Theorem
Hamiltonian Mechanics: Liouville's theorem
Statistical Mechanics 2
Phase Space & Liouvilli Theorem | Easy Method to Understand | SP
Liouville's Theorem
PG TRB Physics Statistical Mechanics Phase space, Liouville's theorem
Liouville's Theorem.
Let's Learn Physics: Chaos in Phase Space
Liouville’s Theorem
LIOUVILLE'S THEOREM||
Statistical Mechanics - Classical Statistics : Liouville’s Theorem - Classical Version
Liouville's theorem
Statmech3 Ensemble theory and Liouvilles theorem
Liouville Theorem | #7 Analytical Mechanics for Chemistry
Комментарии
 0:10:59
0:10:59
 0:03:40
0:03:40
 0:42:35
0:42:35
 1:20:27
1:20:27
 0:31:24
0:31:24
 0:13:37
0:13:37
 0:11:13
0:11:13
 0:44:32
0:44:32
 0:29:10
0:29:10
 0:04:35
0:04:35
 0:29:39
0:29:39
 0:53:51
0:53:51
 1:23:18
1:23:18
 0:10:17
0:10:17
 0:08:35
0:08:35
 0:10:03
0:10:03
 0:55:13
0:55:13
 2:28:01
2:28:01
 0:00:15
0:00:15
 0:07:44
0:07:44
 0:53:12
0:53:12
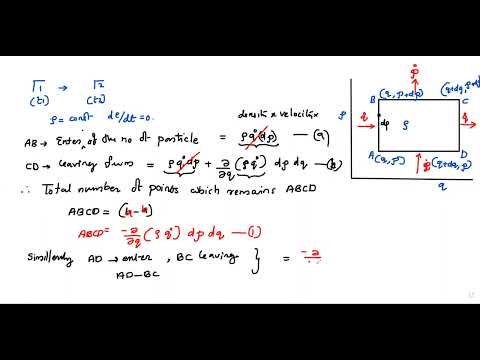 0:20:06
0:20:06
 0:48:09
0:48:09
 0:04:12
0:04:12