filmov
tv
A Nice Homemade Exponential Log Equation

Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
x^{1-log(x)}=1/100
#ChallengingMathProblems #ExponentialEquations #Logarithms
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
x^{1-log(x)}=1/100
#ChallengingMathProblems #ExponentialEquations #Logarithms
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
A Nice Homemade Exponential Log Equation
A Homemade Exponential Log Equation
A Nice Exponential Log #algebra
Solving A Homemade Exponential Equation
A Homemade Exponential Logarithmic Equation
A Nice Homemade Exponential Equation
Solving a Nice Homemade Exponential Equation
A Nice Homemade Exponential Equation
A Nice Homemade Exponential Equation | x^x=y^{1/y}
An Interesting Homemade Exponential Equation
A Logarithmic Exponential System
An Exponential Logarithmic Expression
Solving A Homemade Exponential Equation
rubik's cube magic trick solve...#shorts
A Homemade Exponential Equation | Problem 297
A Homemade Functional Equation with Natural Log
A Homemade Exponential Equation
This trick can make your rubik's cube 2x faster😱🔥#ytshorts#shorts#drcuber
A Homemade Equation with Natural Logs
A Log System With Exponentials
An Interesting Homemade Exponential Equation
Solving A Nice And Easy Exponential System
Last Words of Albert Einstein #shorts
NEWYES Calculator VS Casio calculator
Комментарии
 0:08:52
0:08:52
 0:09:14
0:09:14
 0:05:06
0:05:06
 0:08:46
0:08:46
 0:08:47
0:08:47
 0:05:31
0:05:31
 0:11:52
0:11:52
 0:10:52
0:10:52
 0:09:00
0:09:00
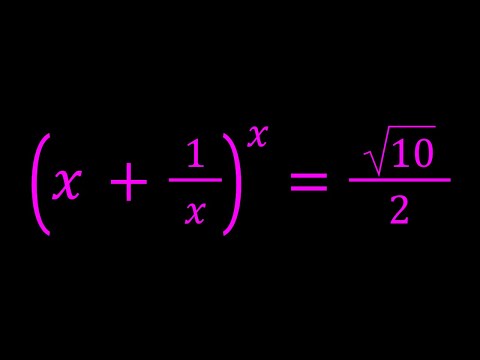 0:08:55
0:08:55
 0:00:29
0:00:29
 0:08:10
0:08:10
 0:08:48
0:08:48
 0:00:17
0:00:17
 0:07:51
0:07:51
 0:09:18
0:09:18
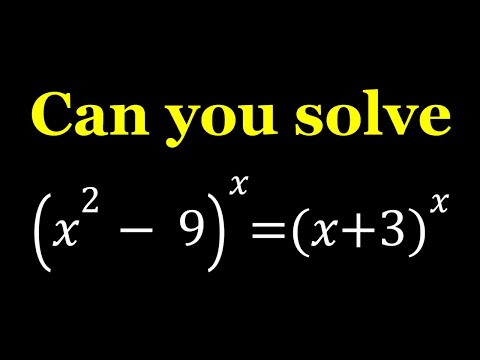 0:08:30
0:08:30
 0:00:16
0:00:16
 0:08:31
0:08:31
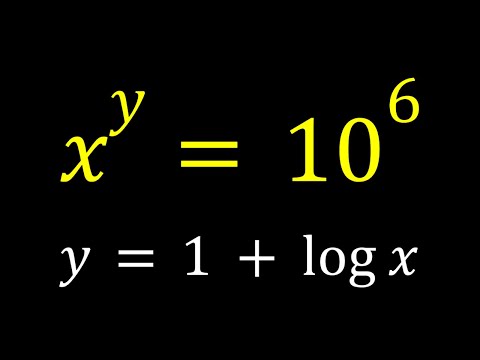 0:09:06
0:09:06
 0:10:04
0:10:04
 0:09:38
0:09:38
 0:00:37
0:00:37
 0:00:14
0:00:14