filmov
tv
Jim Coroneos' 100 Integrals ~ 029 ~ ∫1/(5 + 3cosx).dx

Показать описание
Partly to honour Jim, and partly to fulfil an international need, I have decided to produce 100 videos, showing how to solve his 100 integration 'problems.' I hope you find the videos useful!
This twenty-ninth problem is to evaluate ∫1/(5 + 3cosx).dx
When we integrate a function that is in the form of a fraction containing a simple trigonometric ratio (and sometimes more than one), the best substitution to use is usually what we call "t formulae" or "half angle formulae."
In a later video series I will be deriving and explaining how to use these formulae in considerably more detail. In this video I simply use them to evaluate the integral.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
If you wish to be kept up to date with what I am producing on the website (ad free, spam free, cost free mathematics and study materials), please add your name to the mailing list there.
Best wishes for your study and your mathematics!
Thank you.
Jim Coroneos' 100 Integrals ~ 016 ~ ∫1/[x²√(1-x²)].dx
Jim Coroneos' 100 Integrals ~ 030 ~ ∫1/(3 + 5cosx).dx
Jim Coroneos' 100 Integrals ~ 028 ~ ∫tan³x.dx
Jim Coroneos' 100 Integrals ~ 009 ~ ∫tan‾¹2x.dx
Jim Coroneos' 100 Integrals ~ 019 ~ ∫1/[x√(x²-a²)].dx
Jim Coroneos' 100 Integrals ~ 026 ~ ∫1/[x²(1+x²)].dx
Jim Coroneos' 100 Integrals ~ 020 ~ ∫x/(√x+1).dx
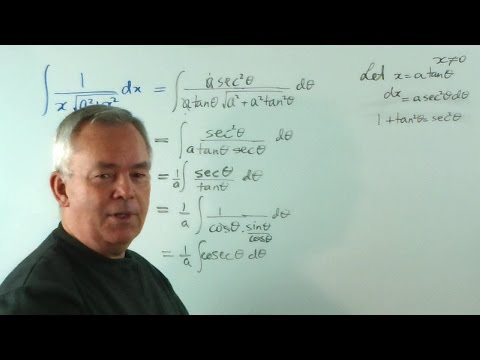
Jim Coroneos' 100 Integrals ~ 017 ~ ∫1/[x√(a²+x²)].dx
Jim Coroneos' 100 Integrals ~ 018 ~ ∫1/[x√(a²-x²)].dx
Jim Coroneos' 100 Integrals ~ 011 ~ ∫x/[(x+4)(x+2)].dx
Jim Coroneos' 100 Integrals ~ 031 ~ ∫sinx/(5 + 3cosx).dx
1-10 OF JIM CORONEOS' 100 INTEGRALS SOLVED!
Jim Coroneos' 100 Integrals ~ 029 ~ ∫1/(5 + 3cosx).dx
Jim Coroneos' 100 Integrals ~ 021 ~ ∫(cos‾¹x)/√(1-x²).dx
Jim Coroneos' 100 Integrals ~ 027 ~ ∫1/(1+x²)².dx
Jim Coroneos' 100 Integrals ~ 015 ~ ∫(1+x)/√(1-x-x²).dx
Jim Coroneos' 100 Integrals ~ 032 ~ ∫1/(1 + cos²x).dx
Jim Coroneos' 100 Integrals ~ 005 ~ ∫sinx.sec³x.dx
Jim Coroneos' 100 Integrals ~ 010 ~ ∫x³/(x² + 1).dx
Jim Coroneos' 100 Integrals ~ 001 ~ ∫x/(x²+4).dx
Jim Coroneos' 100 Integrals ~ 005 ~ ∫sinx.sec³x.dx (Three Methods)
Jim Coroneos' 100 Integrals ~ 014 ~ ∫x³/(2x-1).dx
Jim Coroneos' 100 Integrals ~ 022 ~ ∫√[(x+1)/(x-1)].dx
Jim Coroneos' 100 Integrals ~ 006 ~ ∫cos²(x/2).dx
Комментарии
 0:10:52
0:10:52
 0:12:05
0:12:05
 0:05:09
0:05:09
 0:03:51
0:03:51
 0:15:00
0:15:00
 0:06:00
0:06:00
 0:14:46
0:14:46
 0:17:41
0:17:41
 0:17:36
0:17:36
 0:05:21
0:05:21
 0:03:49
0:03:49
 0:25:01
0:25:01
 0:07:15
0:07:15
 0:12:07
0:12:07
 0:13:12
0:13:12
 0:10:18
0:10:18
 0:07:03
0:07:03
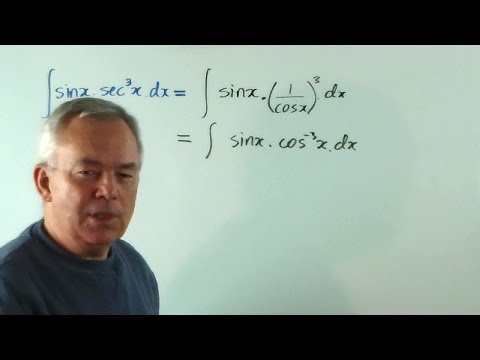 0:05:17
0:05:17
 0:04:49
0:04:49
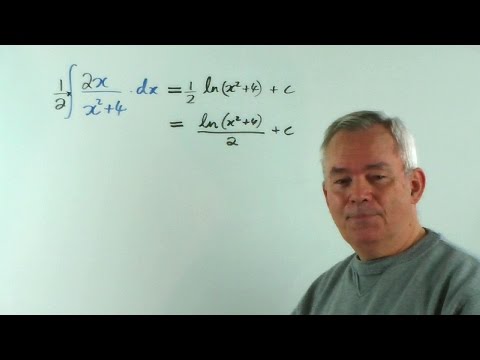 0:03:33
0:03:33
 0:09:47
0:09:47
 0:05:40
0:05:40
 0:05:31
0:05:31
 0:03:33
0:03:33