filmov
tv
Find the value of k if k-3, 2k-3 and 4k+3 are in GP | Geometric Progression
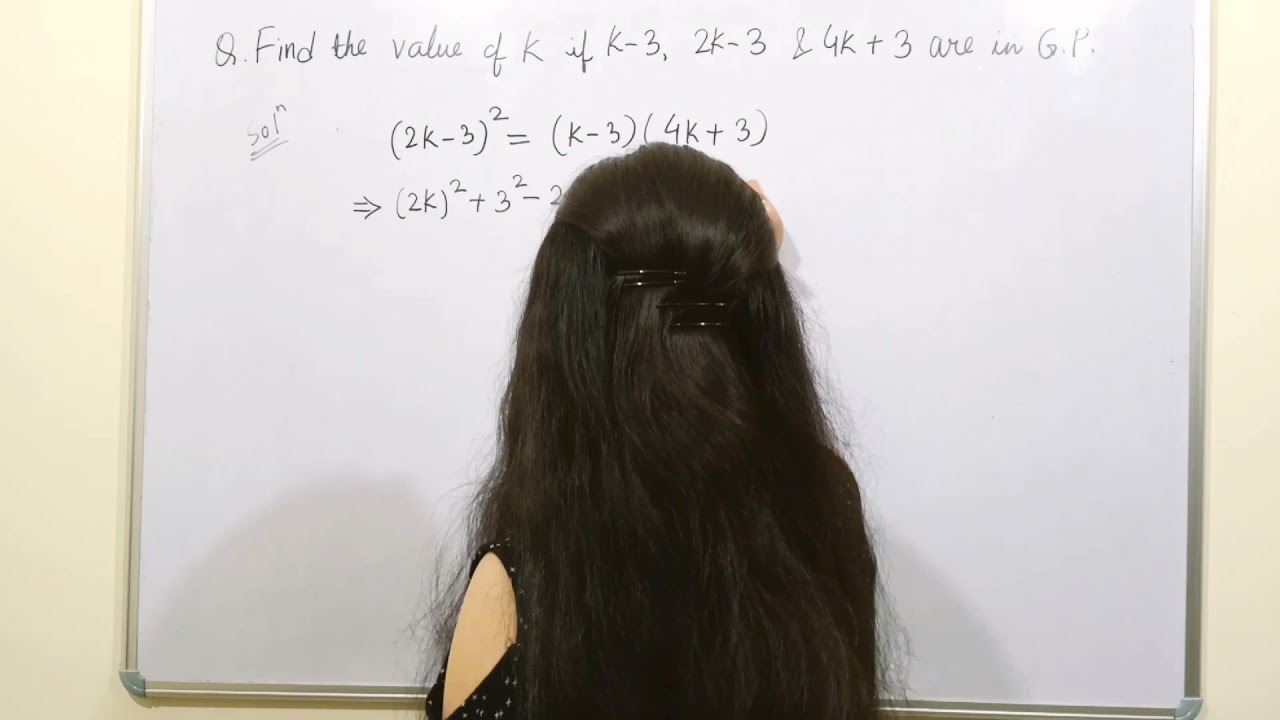
Показать описание
Find the value of k if k-3, 2k-3 and 4k+3 are in GP | Geometric Progression
In mathematics, a geometric progression, also known as a geometric sequence, is a sequence of non-zero numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. For example, the sequence 2, 6, 18, 54, ... is a geometric progression with common ratio 3. Similarly 10, 5, 2.5, 1.25, ... is a geometric sequence with common ratio 1/2.
Generally, to check whether a given sequence is geometric, one simply checks whether successive entries in the sequence all have the same ratio.
The common ratio of a geometric sequence may be negative, resulting in an alternating sequence, with numbers alternating between positive and negative. For instance
1, −3, 9, −27, 81, −243, ...
is a geometric sequence with common ratio −3.
The behaviour of a geometric sequence depends on the value of the common ratio.
If the common ratio is:
✓positive, the terms will all be the same sign as the initial term.
✓negative, the terms will alternate between positive and negative.
✓greater than 1, there will be exponential growth towards positive or negative infinity (depending on the sign of the initial term).
✓1, the progression is a constant sequence.
✓between −1 and 1 but not zero, there will be exponential decay towards zero (→ 0).
✓−1, the absolute value of each term in the sequence is constant and terms alternate in sign.
✓less than −1, for the absolute values there is exponential growth towards infinity, due to the alternating sign.
✓Geometric sequences (with common ratio not equal to −1, 1 or 0) show exponential growth or exponential decay, as opposed to the linear growth (or decline) of an arithmetic progression such as 4, 15, 26, 37, 48, … (with common difference 11). This result was taken by T.R. Malthus as the mathematical foundation of his Principle of Population. Note that the two kinds of progression are related: exponentiating each term of an arithmetic progression yields a geometric progression, while taking the logarithm of each term in a geometric progression with a positive common ratio yields an arithmetic progression.
In mathematics, a geometric progression, also known as a geometric sequence, is a sequence of non-zero numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. For example, the sequence 2, 6, 18, 54, ... is a geometric progression with common ratio 3. Similarly 10, 5, 2.5, 1.25, ... is a geometric sequence with common ratio 1/2.
Generally, to check whether a given sequence is geometric, one simply checks whether successive entries in the sequence all have the same ratio.
The common ratio of a geometric sequence may be negative, resulting in an alternating sequence, with numbers alternating between positive and negative. For instance
1, −3, 9, −27, 81, −243, ...
is a geometric sequence with common ratio −3.
The behaviour of a geometric sequence depends on the value of the common ratio.
If the common ratio is:
✓positive, the terms will all be the same sign as the initial term.
✓negative, the terms will alternate between positive and negative.
✓greater than 1, there will be exponential growth towards positive or negative infinity (depending on the sign of the initial term).
✓1, the progression is a constant sequence.
✓between −1 and 1 but not zero, there will be exponential decay towards zero (→ 0).
✓−1, the absolute value of each term in the sequence is constant and terms alternate in sign.
✓less than −1, for the absolute values there is exponential growth towards infinity, due to the alternating sign.
✓Geometric sequences (with common ratio not equal to −1, 1 or 0) show exponential growth or exponential decay, as opposed to the linear growth (or decline) of an arithmetic progression such as 4, 15, 26, 37, 48, … (with common difference 11). This result was taken by T.R. Malthus as the mathematical foundation of his Principle of Population. Note that the two kinds of progression are related: exponentiating each term of an arithmetic progression yields a geometric progression, while taking the logarithm of each term in a geometric progression with a positive common ratio yields an arithmetic progression.
Комментарии
 0:03:35
0:03:35
 0:06:17
0:06:17
 0:03:18
0:03:18
 0:05:03
0:05:03
 0:06:26
0:06:26
 0:03:50
0:03:50
 0:00:54
0:00:54
 0:02:50
0:02:50
 0:00:58
0:00:58
 0:04:50
0:04:50
 0:10:01
0:10:01
 0:05:12
0:05:12
 0:04:59
0:04:59
 0:03:22
0:03:22
 0:13:41
0:13:41
 0:07:53
0:07:53
 0:05:08
0:05:08
 0:05:33
0:05:33
 0:00:36
0:00:36
 0:07:08
0:07:08
 0:02:33
0:02:33
 0:04:35
0:04:35
 0:07:08
0:07:08
 0:04:45
0:04:45