filmov
tv
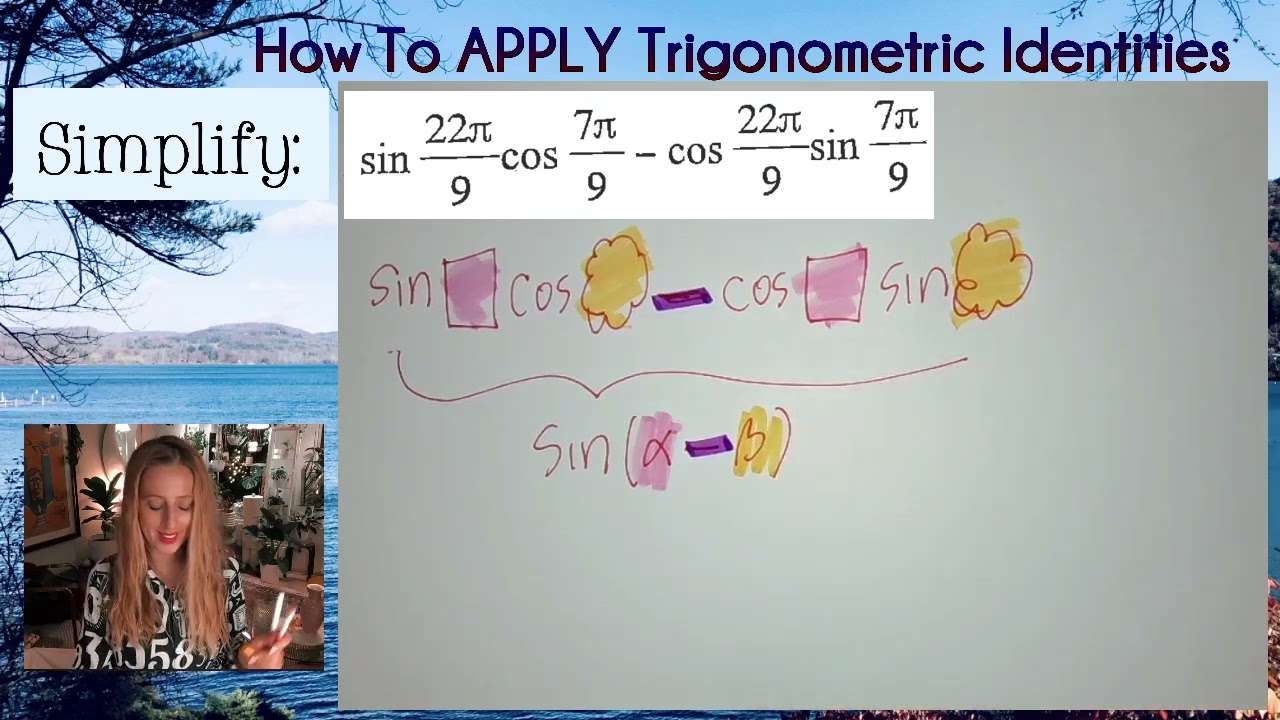
Simplify sin(22π/9)cos(7π/9) - cos(22π/9)sin(7π/9)
Показать описание
🌈 Applying Trig Identities ⭐️
----- • ----- • ----- • ----- • ----- • -----
P15
🟣
🔗
🔵
🔗
🟢
🔗
🟡
🔗
🟠
🔗
🔴 If csc𝜃 = 25/7 & 90º<𝜃<180º, find csc(2𝜃).
P16
🟣
🔗
🔵
🔗
🟢
🔗
🟡 If sin𝜃 = -3/5 & 3π/2<𝜃<2π, find sec(𝜃/2).
🟠 If csc𝜃 = 8/3 & π/2<𝜃<π, find cot(𝜃/2).
🔴 If sec𝜃 = 5/4 & 0º<𝜃<90º, find cos(𝜃/2).
P17
🟣
🔗
🔵
🔗
🟢
🔗
🟡
🔗
🟠
🔗
🔴
🔗
P18
🟣
🔗
🔵
🔗
🟢
🔗
🟡
🔗
🟠
🔗
🔴
🔗
P18
🔴 If cot(-𝜃)= 0.47, find tan( 𝜃-(π/2) ).
🔗 lllllll
🟠 If sec𝜃 = -5.76, find csc( 𝜃-(π/2) ).
🔗 llllll
🟡 Find the exact value of cos15º by using
the appropriate Angle Sum/Diff Identities.
🟢 Find the exact value of tan15º by using
the appropriate Angle Sum/Diff Identities.
🔵 Simplify: ( tan(3u)-tan(-u) ) / ( 1+ tan(3u)•tan(-u) )
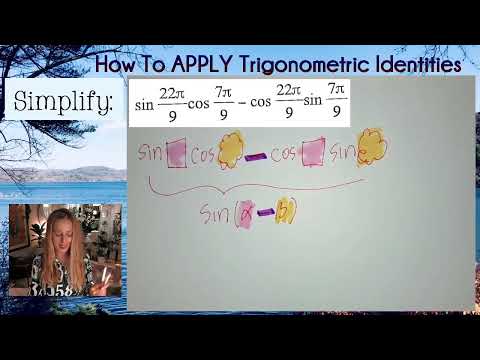
🟣 Simplify: cos(-6v)cos(-5v) + sin(-6v)sin(-5v)
P19
🟣 Find the exact value:
( tan(46º) - tan(1º) ) / ( 1 + tan(46º)•tan(1º) )
🔵 Find the exact value:
sin(22π/9)cos(7π/9) - cos(22π/9)sin(7π/9)
🟢 Find the exact value:
cos(25π/9)cos(10π/9) + sin(25π/9)sin(10π/9)
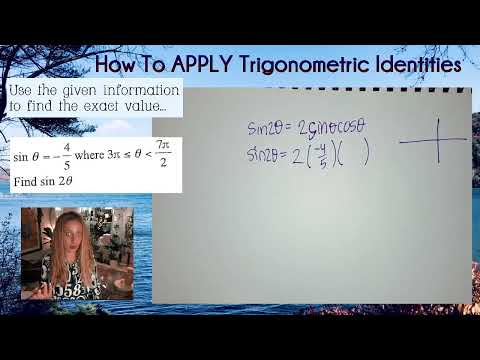
🟡 If sin𝜃 = -4/5 & 3π<𝜃<7π/2, find sin(2𝜃).
🟠 If cos𝜃 = 3/5 & 0<𝜃<π, find cos(2𝜃).
🔴 If cos𝜃 =-3/5 & 450º<𝜃<540º, find sin(𝜃/2).
P20
🔴 If sin𝜃 = 12/13 & 450º<𝜃<540º, find sin(2𝜃).
🟠 If cos𝜃 = √(7)/7 & 270º<𝜃<360º, find cos(2𝜃).
🟡 If cos𝜃 = -3/5 & 90º<𝜃<180º, find tan(2𝜃).
🟢 If tan𝜃 = -4/3 & 90º<𝜃<180º, find tan(𝜃/2).
🔵 If sin𝜃 = 12/13 & π/2<𝜃<π, find tan(2𝜃).
🟣 If cos𝜃 = -3/5 & 450º<𝜃<540º, find sin(𝜃/2).
P21
💜 Use a half-angle identity to find cos(9π/8).
💙 Use a half-angle identity to find tan(15º).
💚 Use a half-angle identity to find sin(112.5º).
💛 Use a half-angle identity to find tan(23π/12).
🧡 If tan𝜃 = 15/8 & π<𝜃<3π/2, find cos(𝜃/2).
❤️ If tan𝜃 = 5√(39)/39 & 3π/2<𝜃<2π, find sin(𝜃/2).
↑ ↑ ↑ ↑ error at the end of this video...
(I fix it in the orange heart video.)
----- • ----- • ----- • ----- • ----- • -----
P15
🟣
🔗
🔵
🔗
🟢
🔗
🟡
🔗
🟠
🔗
🔴 If csc𝜃 = 25/7 & 90º<𝜃<180º, find csc(2𝜃).
P16
🟣
🔗
🔵
🔗
🟢
🔗
🟡 If sin𝜃 = -3/5 & 3π/2<𝜃<2π, find sec(𝜃/2).
🟠 If csc𝜃 = 8/3 & π/2<𝜃<π, find cot(𝜃/2).
🔴 If sec𝜃 = 5/4 & 0º<𝜃<90º, find cos(𝜃/2).
P17
🟣
🔗
🔵
🔗
🟢
🔗
🟡
🔗
🟠
🔗
🔴
🔗
P18
🟣
🔗
🔵
🔗
🟢
🔗
🟡
🔗
🟠
🔗
🔴
🔗
P18
🔴 If cot(-𝜃)= 0.47, find tan( 𝜃-(π/2) ).
🔗 lllllll
🟠 If sec𝜃 = -5.76, find csc( 𝜃-(π/2) ).
🔗 llllll
🟡 Find the exact value of cos15º by using
the appropriate Angle Sum/Diff Identities.
🟢 Find the exact value of tan15º by using
the appropriate Angle Sum/Diff Identities.
🔵 Simplify: ( tan(3u)-tan(-u) ) / ( 1+ tan(3u)•tan(-u) )
🟣 Simplify: cos(-6v)cos(-5v) + sin(-6v)sin(-5v)
P19
🟣 Find the exact value:
( tan(46º) - tan(1º) ) / ( 1 + tan(46º)•tan(1º) )
🔵 Find the exact value:
sin(22π/9)cos(7π/9) - cos(22π/9)sin(7π/9)
🟢 Find the exact value:
cos(25π/9)cos(10π/9) + sin(25π/9)sin(10π/9)
🟡 If sin𝜃 = -4/5 & 3π<𝜃<7π/2, find sin(2𝜃).
🟠 If cos𝜃 = 3/5 & 0<𝜃<π, find cos(2𝜃).
🔴 If cos𝜃 =-3/5 & 450º<𝜃<540º, find sin(𝜃/2).
P20
🔴 If sin𝜃 = 12/13 & 450º<𝜃<540º, find sin(2𝜃).
🟠 If cos𝜃 = √(7)/7 & 270º<𝜃<360º, find cos(2𝜃).
🟡 If cos𝜃 = -3/5 & 90º<𝜃<180º, find tan(2𝜃).
🟢 If tan𝜃 = -4/3 & 90º<𝜃<180º, find tan(𝜃/2).
🔵 If sin𝜃 = 12/13 & π/2<𝜃<π, find tan(2𝜃).
🟣 If cos𝜃 = -3/5 & 450º<𝜃<540º, find sin(𝜃/2).
P21
💜 Use a half-angle identity to find cos(9π/8).
💙 Use a half-angle identity to find tan(15º).
💚 Use a half-angle identity to find sin(112.5º).
💛 Use a half-angle identity to find tan(23π/12).
🧡 If tan𝜃 = 15/8 & π<𝜃<3π/2, find cos(𝜃/2).
❤️ If tan𝜃 = 5√(39)/39 & 3π/2<𝜃<2π, find sin(𝜃/2).
↑ ↑ ↑ ↑ error at the end of this video...
(I fix it in the orange heart video.)
 0:03:03
0:03:03
 0:04:17
0:04:17
 0:02:15
0:02:15
 0:03:02
0:03:02
 0:06:59
0:06:59
 0:07:51
0:07:51
 0:03:45
0:03:45
 0:03:59
0:03:59
 0:04:04
0:04:04
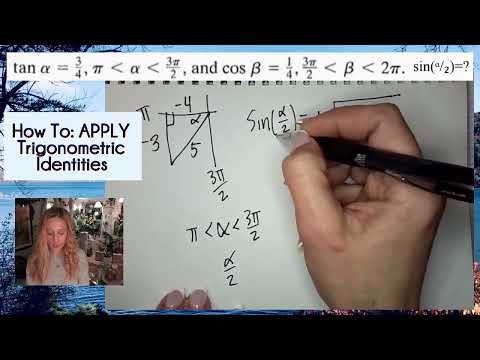 0:03:00
0:03:00
 0:05:35
0:05:35
 0:05:42
0:05:42
 0:03:37
0:03:37
 0:05:55
0:05:55
 0:03:57
0:03:57
 0:02:46
0:02:46
 0:03:17
0:03:17
 0:04:16
0:04:16
 0:08:48
0:08:48
 0:03:12
0:03:12
 0:05:45
0:05:45
 0:08:35
0:08:35
 0:05:47
0:05:47