filmov
tv
Permutations Involving Repeated Symbols - Example 2

Показать описание
Permutations Involving Repeated Symbols - Example 1
Permutations Involving Repeated Symbols - Example 2
Permutations with Repeated Symbols
PERMUTATIONS INVOLVING REPEATED SYMBOLS
Permutations and Combinations Tutorial
Probability & Statistics (41 of 62) Permutations and Combinations - Example 6
Permutations: how do we account for repetitions?
Permutations and Combinations : Arrangements involving repeated characters.
Solving Problems Part 3-Word and people arrangement problems(Permutations and combinations)
Permutations with Repetition | Combinatorics, Permutations and Combinations
Permutations with restrictions - items not together | ExamSolutions
Permutations and Combinations (Repeated letters)
Counting Permutations with Duplicates
Permutations with Repetition
Permutations with Repeated Letters / Elements
Permutations : Repeated characters when arranging n objects
Algebra II 15.2a, Permutations & repeated use of the same object (repetition)
Permutations | MathHelp.com
13 7 Combinations & Repeated Permutations Problem Video
Example Question of Permutations Involving Restrictions
ACT Math: How to do Permutations & Distinct Permutations
Permutations Involving Non Ordered Elements
PN-7 Distinguishable Permutations
FDN30 4 4 Permutations of Objects that repeat
Комментарии
 0:05:17
0:05:17
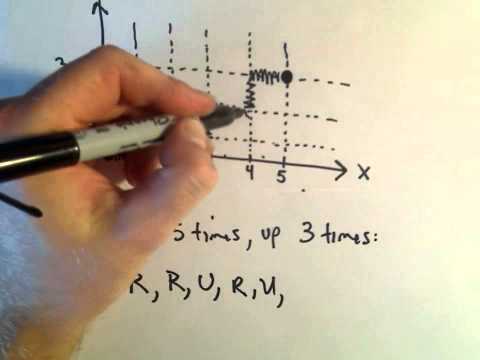 0:03:36
0:03:36
 0:08:09
0:08:09
 0:10:00
0:10:00
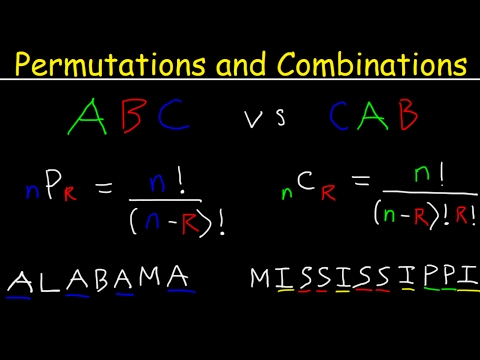 0:17:41
0:17:41
 0:04:54
0:04:54
 0:12:55
0:12:55
 0:05:00
0:05:00
 0:07:15
0:07:15
 0:07:01
0:07:01
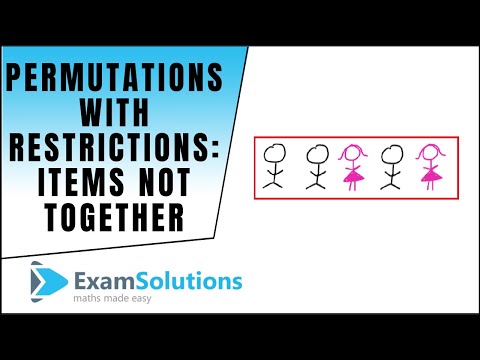 0:08:10
0:08:10
 0:01:01
0:01:01
 0:10:13
0:10:13
 0:06:31
0:06:31
 0:03:20
0:03:20
 0:05:12
0:05:12
 0:05:11
0:05:11
 0:01:15
0:01:15
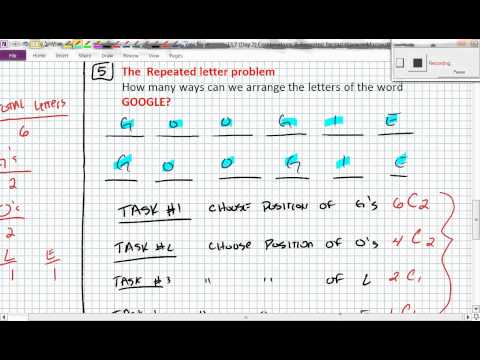 0:06:07
0:06:07
 0:04:36
0:04:36
 0:08:28
0:08:28
 0:07:43
0:07:43
 0:05:00
0:05:00
 0:23:38
0:23:38