filmov
tv
Can you do this crazy integral?
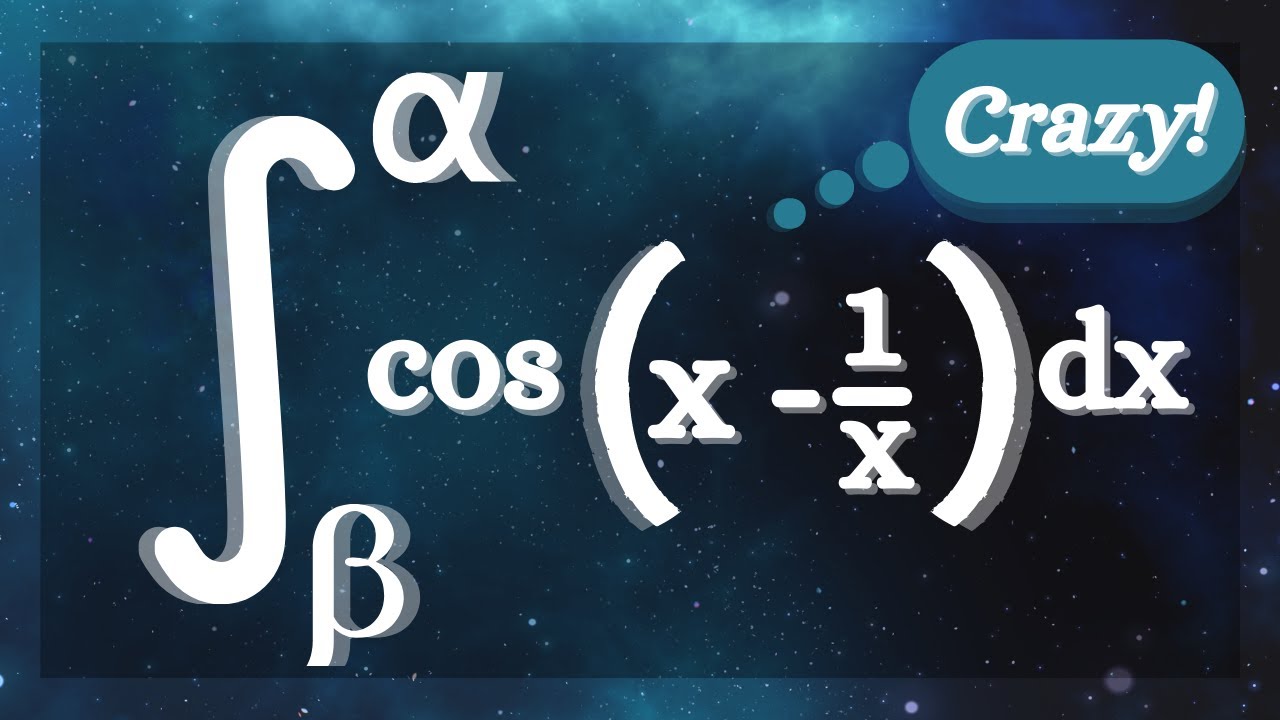
Показать описание
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
David Guetta & Becky Hill & Ella Henderson - Crazy What Love Can Do (Lyric Video)
David Guetta & Becky Hill & Ella Henderson - Crazy What Love Can Do (Official Music Video)
Gnarls Barkley - Crazy (Official Video) [4K Remaster]
David Guetta & Becky Hill & Ella Henderson - Crazy What Love Can Do (Acoustic Video)
Ekoh x Crazy Town - Butterfly 2021 (Official Music Video)
Seal - Crazy (Official Music Video) [HD]
David Guetta & Becky Hill & Ella Henderson - Crazy What Love Can Do | Caleb Marshall | Dance...
Can You Do This Crazy Crunch-Up Challenge?
16 CRAZY SCIENCE EXPERIMENTS
Do You Like Broccoli Ice Cream? | Food Song for Kids! | Super Simple Songs
4 CRAZY CODES! THIS IS HOW YOU DO AN ANNIVERSARY! KR SUMMARY! [Solo Leveling: Arise]
Crazy Rich Asians Official Soundtrack | Can't Help Falling in Love (Wedding Scene) - Kina Grann...
Crazy Cello Trick - Can you do it too?
Can Silence Actually Drive You Crazy?
World’s *STRONGEST* Objects vs Hydraulic Press! (CRAZY)
CUTE CRAZY HAIR DAY HAIR TUTORIAL
You're CRAZY if you would do THIS...
David Guetta & Becky Hill & Ella Henderson - Crazy What Love Can Do (Live Performance)
5 CRAZY Magic Tricks Anyone Can Do | Revealed
Cal Meets Hannah’s Boyfriend | Crazy Stupid Love | Max
Can you do this crazy integral?
Do you know why Germans are crazy? 🇩🇪 #memes #germany #deutschland
YOU DRIVE ME CRAZY 😈
Crazy Dave checking on Doom-shroom (gone wrong) #pvz #plantsvszombies
Комментарии
 0:02:49
0:02:49
 0:02:58
0:02:58
 0:03:01
0:03:01
 0:03:12
0:03:12
 0:02:44
0:02:44
 0:04:31
0:04:31
 0:03:11
0:03:11
 0:00:36
0:00:36
 0:07:28
0:07:28
 0:02:43
0:02:43
 0:08:12
0:08:12
 0:03:12
0:03:12
 0:00:22
0:00:22
 0:10:33
0:10:33
 0:14:08
0:14:08
 0:00:22
0:00:22
 0:00:57
0:00:57
 0:02:49
0:02:49
 0:10:03
0:10:03
 0:04:01
0:04:01
 0:10:35
0:10:35
 0:00:22
0:00:22
 0:00:13
0:00:13
 0:00:06
0:00:06