filmov
tv
Brent's Minimization Method
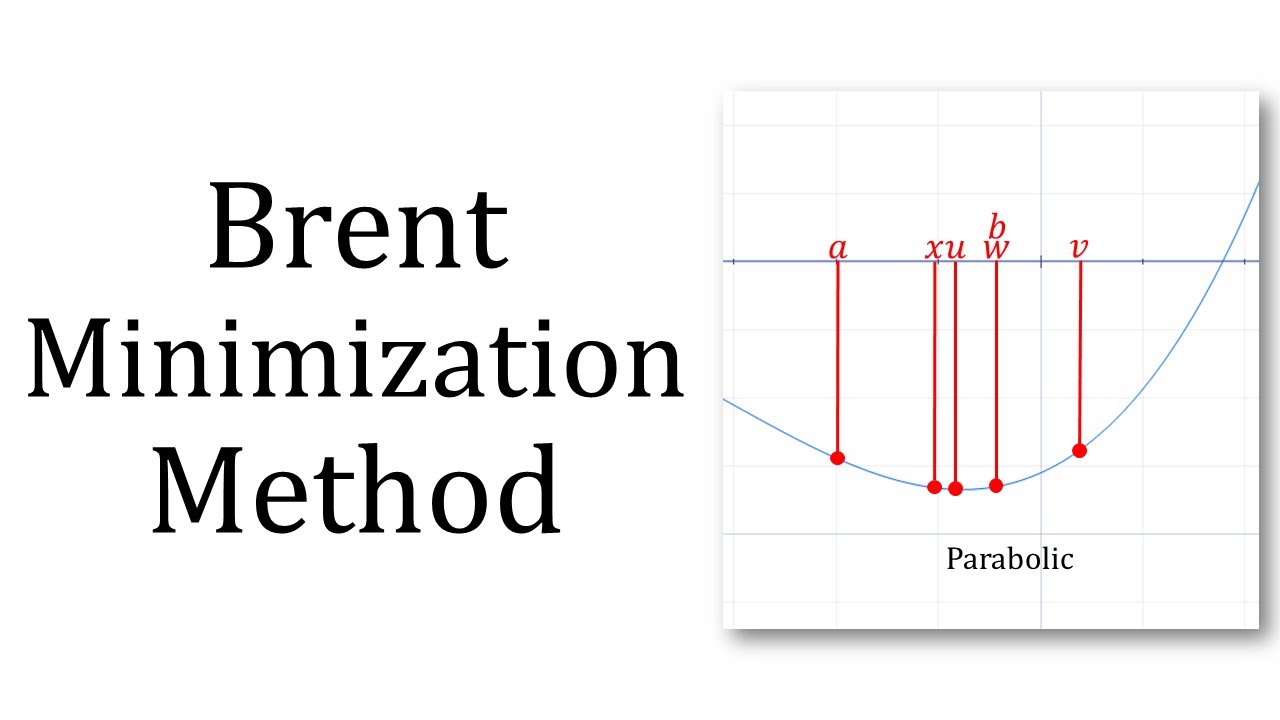
Показать описание
Chapters:
0:00 Intro
0:16 Scaffolding
0:31 Motivation
1:17 Parabolic Interpolation Review
1:48 Renaming Variables
2:40 Brent's Method Algorithm
3:19 SPI Behaving?
4:08 Note on Updating
4:38 Brent's Method Visualization
6:02 Numerical Example
6:29 Note on Steps
6:43 MATLAB fminbnd
7:12 Minimum Strategy - Derivative
7:49 Note on Convergence Order
8:04 Oscar's Notes
8:39 Outro
Suggested Viewing:
References:
Background music "The Golden Present" by @JesseGallagher
#GoldenSectionSearch #SuccessiveParabolicInterpolation #NumericalAnalysis
Brent's Minimization Method
8.1.5 Brent's method
Brent's Method
Optimization in Machine Learning - Univariate Optimization - Brent's method
Brent's Method - An algorithm with guaranteed convergence for finding a zero of a function.
Lab02_7 Brent Method
Lecture 13 ROE Brents Method
6.2.7 Brent-Dekker method
Brent’s Method with MATLAB | Numerical Methods | @MATLABHelper
2.2.5-Roots: Brent's (Hybrid) Method
Brent's Method (Part 2)
Application of Brent's Cycle Detection Algorithm
Numerical Methods Lec23 Ch08 1D Optimization6 MATLAB for Optimization - fmndbound and Brents Method
Brent's Method (Part 3)
CHE 531 Brent's Method Final Project Video
Intro to Scipy Optimization: Minimize Method
Brent's Theorem Part 1
Lecture 12 - Scan (Brent-Kung)
Lecture 12 - Scan (Brent Kung)
Sprinters Fighting For It 😅
Brent's Theorem Part 2
Ecuaciones NO lineales en Python (Parte 4) - Método de Brent!
2018, Macroeconomics Lecture, Brent Neiman, 'Accounting for Factorless Income'
Lecture 21: Minimizing a Function Step by Step
Комментарии
 0:09:01
0:09:01
 0:06:18
0:06:18
 0:14:01
0:14:01
 0:09:34
0:09:34
 0:06:03
0:06:03
 0:07:58
0:07:58
 0:07:31
0:07:31
 0:05:24
0:05:24
 0:08:29
0:08:29
 0:05:39
0:05:39
 0:11:09
0:11:09
 0:02:09
0:02:09
 0:04:55
0:04:55
 0:18:58
0:18:58
 0:06:19
0:06:19
 0:26:26
0:26:26
 0:04:11
0:04:11
 1:26:07
1:26:07
 1:26:07
1:26:07
 0:00:06
0:00:06
 0:02:27
0:02:27
 0:07:38
0:07:38
 0:22:44
0:22:44
 0:53:45
0:53:45