filmov
tv
Quantum field theory, Lecture 5

Показать описание
This winter semester (2016-2017) I am giving a course on quantum field theory. This course is intended for theorists with familiarity with advanced quantum mechanics and statistical physics. The main objective is introduce the building blocks of quantum electrodynamics.
Here in Lecture 5 I begin the argument that the quantum Klein-Gordon field is a relativistic quantum theory.
Here in Lecture 5 I begin the argument that the quantum Klein-Gordon field is a relativistic quantum theory.
Quantum field theory, Lecture 5
Quantum Field Theory I - Lecture 5
Advanced quantum field theory, Lecture 5
Quantum Field Theory II - Lecture 5
Quantum Field Theory - Lecture 5: Lehmann Representation and Renormalisation of the 2-Point Function
Lecture 5 | Introduction to Topological Quantum Field Theories | Andrey Losev | Лекториум
Advanced Quantum Mechanics Lecture 5
Brian Cox explains quantum mechanics in 60 seconds - BBC News
Introduction to AdS/QCD
BHT50 - Quantum Field Theory in Curved Spacetime - Níckolas Alves - Lecture 5
Quantum Field Theory Lecture 6: Mastering the Gamma Matrices (Part 1)
Quantum Field Theory I: Lecture 5 by Ashoke Sen
The Bridge Between Math and Quantum Field Theory
Introduction to conformal field theory, Lecture 5
Lecture 5: Operators and the Schrödinger Equation
MASTER THE DIRAC GAMMA MATRICES! Quantum Field Theory Lecture 7
METU - Quantum Mechanics II - Week 5 - Lecture 3
Field Theory Fundamentals in 20 Minutes!
Quantum Fields: The Most Beautiful Theory in Physics!
The Terrifying Quantum Theory Scientists Don’t Even Want To Talk About
How Physicists Proved The Universe Isn't Locally Real - Nobel Prize in Physics 2022 EXPLAINED
Solving the Impossible in Quantum Field Theory
Theoretical Physicist Brian Greene Explains Time in 5 Levels of Difficulty | WIRED
Quantum Field Theory
Комментарии
 1:21:22
1:21:22
 2:34:13
2:34:13
 1:28:23
1:28:23
 2:19:40
2:19:40
 1:25:21
1:25:21
 1:38:55
1:38:55
 1:43:32
1:43:32
 0:01:22
0:01:22
 0:19:16
0:19:16
 0:40:51
0:40:51
 0:49:57
0:49:57
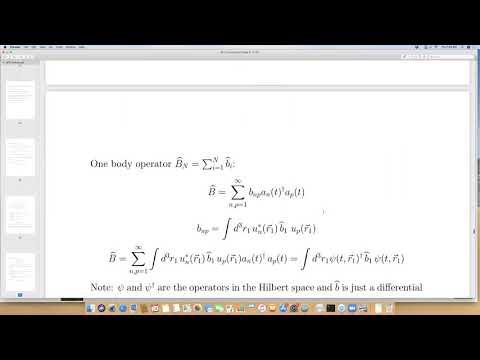 1:30:31
1:30:31
 0:02:46
0:02:46
 1:30:44
1:30:44
 1:23:14
1:23:14
 1:56:47
1:56:47
 0:40:33
0:40:33
 0:22:44
0:22:44
 0:14:31
0:14:31
 0:22:48
0:22:48
 0:12:48
0:12:48
 0:15:21
0:15:21
 0:31:26
0:31:26
 0:05:30
0:05:30