filmov
tv
Hamiltonian Mechanics in 10 Minutes
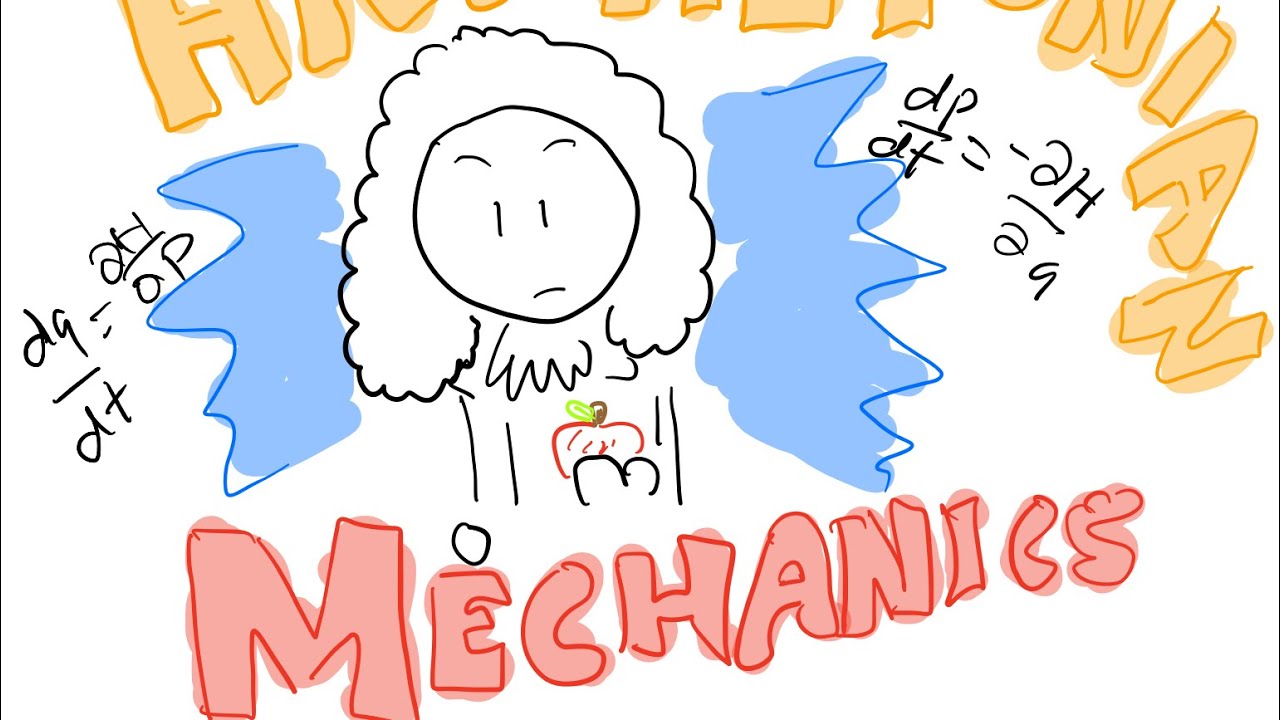
Показать описание
In this video I go over the basics of Hamiltonian mechanics. It is the first video of an upcoming series on a full semester university level Hamiltonian mechanics series.
Corrections
-4:33 the lagrangian should have a minus sign between the first two terms, not a plus.
Corrections
-4:33 the lagrangian should have a minus sign between the first two terms, not a plus.
Hamiltonian Mechanics in 10 Minutes
Lagrangian and Hamiltonian Mechanics in Under 20 Minutes: Physics Mini Lesson
Three ways to do #classsicalmechanics. #hamiltonian #newtonian #lagrangian
Is this the biggest equation in Physics? The lagrangian of the standard model #physics #science
Newtonian VS Lagrangian Mechanics #Shorts
Streamlines, Pathlines, and Streaklines - Eulerian vs. Lagrangian in 10 Minutes!
Block on an Incline: Newtonian, Lagrangain and Hamiltonian Solutions
What is a Hamiltonian? Quantum Jargon Explained
The Most Beautiful Result in Classical Mechanics
What is the Hamiltonian in Classical Mechanics? Lagrangian and Hamiltonian Mechanics - Ep 97 Clips
Understanding Hamiltonian mechanics: (1) The math
Deriving Hamilton's Principle
Classical Mechanics Video 13 -- Hamiltonian Mechanics Example
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
Lagrangian Mechanics I: Introducing the fundamentals
How REAL Men Integrate Functions
Field Theory Fundamentals in 20 Minutes!
H of Quantum. The Hamiltonian.
Classical Hamiltonian & Hamiltonian Operator in Quantum Mechanics (Kinetic+Potential=Total Energ...
Hamilton's principle for Hamiltonian mechanics
Hamiltonian mechanics with constraints
Brian Cox explains quantum mechanics in 60 seconds - BBC News
Understanding the Hamiltonian through a Falling Stone
Aspirants practicing eatingetiquette # SSB #SSBPreparation #NDA #CDS #Defence #DefenceAcademy
Комментарии
 0:09:51
0:09:51
 0:18:33
0:18:33
 0:00:59
0:00:59
 0:00:59
0:00:59
 0:01:00
0:01:00
 0:10:52
0:10:52
 0:24:31
0:24:31
 0:00:54
0:00:54
 0:11:35
0:11:35
 0:06:26
0:06:26
 0:07:38
0:07:38
 0:23:57
0:23:57
 0:27:37
0:27:37
 0:00:16
0:00:16
 0:22:58
0:22:58
 0:00:35
0:00:35
 0:22:44
0:22:44
 0:10:05
0:10:05
 0:05:08
0:05:08
 0:16:57
0:16:57
 0:20:51
0:20:51
 0:01:22
0:01:22
 0:00:25
0:00:25
 0:00:11
0:00:11