Transient Analysis of the RLC Circuit (with Examples)

In this video, you will learn about the transient analysis of the RLC circuit. So, in this video, the transient response for the series and parallel RLC Circuit have been discussed. And at the end, few problems related to this Series and Parallel RLC transients have been solved. Also, the response of the circuits in the given solved examples have been plotted for the better understanding of this transients in the RLC Circuit.

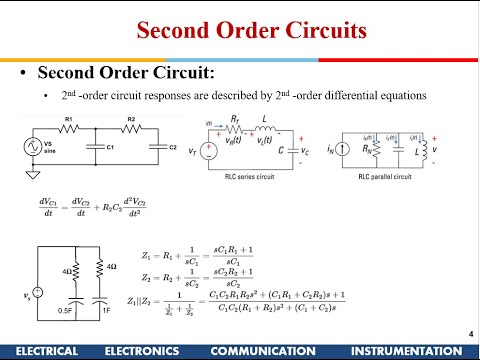
Now, in the case of RLC circuit, during the transient analysis, when we write KVL or KCL equation then we will get second order linear differential equation. And the response of the system depends upon the roots of this differential equation.
Now, in the RLC circuits, L and C have a tendency to induce the oscillation in the circuit, while Resistor R has a tendency to suppress or damp out this oscillation.
So, depending upon the value of R, L and C and ultimately on the roots of the equation, we can have 4 different types of response.

For, series RLC Circuit:

Case 1: (R/2L)^2 is greater than (1/LC)

If the roots are real, negative and different, then the response of the system will be an overdamped response.

Case 2: (R/2L)^2 = (1/LC)

If the roots are Real and equal, then the response of the system will be a critically damped response.

Case 3: (R/2L)^2 is less than (1/LC)

If the roots are complex conjugate then the transient response of the circuit will be underdamped and it will have some oscillation in the circuit, which will die out eventually.

Case 4: R=0
The roots will be purely imaginary, and response will be purely oscillatory without any damping.

For, parallel RLC Circuit:

Case 1: (1/2RC)^2 is greater than (1/LC)

If the roots are real, negative and different, then the response of the system will be an overdamped response.

Case 2: (1/2RC)^2 = (1/LC)

If the roots are Real and equal, then the response of the system will be a critically damped response.

Case 3: (1/2RC)^2 is less than (1/LC)

If the roots are complex conjugate then the transient response of the circuit will be underdamped and it will have some oscillation in the circuit, which will die out eventually.

Case 4: R=0
The roots will be purely imaginary, and response will be purely oscillatory without any damping.

The pdf file for the solution of differential equations for the different cases (critically damped and underdamped) is given here.

Timestamps for the different topics covered in this video is given below:

0:58 Transient Response of Series RLC Circuit

11:05 Graphical Representation of different transient Response

13:20 Transient Response of parallel RLC Circuit

18:48 Example 1: Series RLC Circuit

24:44 Example 2: Parallel RLC Circuit

Link for the other videos related to Transient Analysis:

1) Transient Analysis: Behaviour of basic circuit components

2) Transient Analysis: First order RL and RC Circuits

3) Transient analysis: Solved Examples on First order RC and RL Circuits

4) Transient Analysis using Laplace Transform:

This video will be helpful to all students of science and engineering in understanding the transient analysis for the series and parallel RLC circuit.

#TransientAnalysis
#ALLABOUTELECTRONICS

Check out the second channel :
@allaboutelectronics-quiz

Follow me on YouTube:

Follow me on Facebook:

Follow me on Instagram:

Music Credit:

[ALLABOUTELECTRONICS]

Transient Analysis using Laplace Transform:

The downloadable PDF file link has been updated in the description for the solution of the differential equation for different cases.(Under-damped and Critically damped)

[frankreiserm.s.]

I like how you dive into the calculus. I dropped out of electrical engineering school because I got lost in the calculus. I think that you should have given the resonant frequency equation, f = 1/ 2 pi times the square roof of L times C.. I have memorized all electronics equation, and know them well. I like to put circuitry on a breadboard and then screw around with the external components to create new engineered circuits. I am intending to try to change an am radio ic, where the frequency depends on the exterior descrete components, and try to turn it into an low frequency receiver. We will see, but that is the fun of it! NOW TEACH ME PROFESSOR!

[CorporateCat]

This is the best explanation of RLC ever!

[justmanojkc]

Best video about transient response yet. Thanks for such a crystal clear video.

[ketansharma]

At @8:11 it should be D1, D2 instead of -D1, -D2 which will make the solution C1*exp(D1*t)+C2*exp(D2*t)

[lioneldynasty]

Please check your arithmatic. on the solution equations "e" was raised to the -D and in the first example, D1=-4 and D2=-5 and when you plug those into the equation, i=c1e^4t + c2e^5t not i = c1e^-4t + c2e^-5t, unless if we supposed to substitute D and not -D.

Please rectify those and besides that, your explanations were on point. keep up the good work!

[martinzupet]

When you solve the differential equation that is equal to 0 (solving homegenus differential equation), you get system's natural response, not tranzient. Tranzient response is a particular solution of a differential equation with a given voltage imput function (non-homogenus).

[mnada]

Please add subtitle, I use it to keep notes for important point in the discussion
Great Explanation 👍👏

[Naga_]

Excellent explanation sir. Due to your explanation with examples I have learnt transient response in RLC case. I am requesting you sir please send depth of contents or basics or tricks to find easy way to solve problems etc. Thank you sir

[ugotmail]

WOW one of THE BEST EXPLANATION!!!! Thank you sir.

[jakatorussom]

Thank you for the video instruction...Very nice work

[bagadivenkatramesh]

A good detailed explanation.
Thank you very much.
Keep it up. 🙏

[meetpatel]

sir thanks for making me pass i was not knowing transient RL, RC and RLC but by learning from your videos i can easily learn what's going on.

[billionaire]

Amazing bro concepts r understood very well...

[johnpatrickobra]

Thank you sir you save me from getting zero ❤️

[ТамирланБельгибаев]

So, this is nothing, but a perfect video!

[noweare]

This is definitly one of your more math intensive videos. Excellent presentation compard to other videos I have watched. There is a free math program, I think it is also open source, called wxMaxima which is the graphical front end for Maxima. It is a computer algerbra system software. Very powerfull that allows you do engineering mathmatics and much more. Much better than doing the math by hand if you have to do it more than once.

[shaziatabassum]

Best explanation of rlc circuit...thank u sir😊

[adityasaurabh]

Sorry to say but Your concepts are very Confusing....Plz don't skip little concepts

[BentHestad]

Superb stuff! Thanks also for excellent PDF!