filmov
tv
Gate 2022 Mathematics Solution | Complete Solution | 1 to 65 | By Vanita Ma'am
Показать описание
GATE Mathematics Solution Series
Q.1 to Q.65
By Vanita Ma'am
Axiomatikos
Institution of Mathematics
Contact : +91-8433218478 & +91-7901716990
#gatecoachingonline #gatesolutions #mathematics #gate
As you grow older, an injury to your, In a 500 m race, P and Q have speeds in the ratio of 3 ∶ 4. Q starts the race
when P has already covered 140 m.Three bells P, Q, and R are rung periodically in a school,
Some bottles are cups,All cups are knives,The figure below shows the front and rear view of a disc, which is shaded with
identical patterns,Altruism is the human concern for the wellbeing of others,
There are two identical dice with a single letter on each of the faces,The price of an item is 10% cheaper in an online store S compared to the price
at another online store M,The letters P, Q, R, S, T and U are to be placed one per vertex on a regular convex
hexagon,An ant is at the bottom-left corner of a grid,Let M ∈ Rm×n with m n 2. If rankM = n, then the system of
linear equations Mx = 0 has x = 0 as the only solution,Consider the real function of two real variables given by
u(x, y) = e2xsin 3x cos 2y cosh 3y − cos 3x sin 2y sinh 3y,where C is the circle of radius 2 centred at the origin taken in the anti-clockwise
direction is,If X0 contains
two distinct points x and y and the line segment joining them,Let ek : k ∈ N be an orthonormal basis for a Hilbert space H,Neither M nor N is connected,converges to
2,The initial value problem,If eigenfunctions corresponding to distinct eigenvalues λ of the Sturm-Liouville
problem,boundary conditions
u(0, t) = 1 and u2, t = 3, t 0, at x = 1 is,Consider 0, 1, T1, where T1 is the subspace topology induced by the Euclidean
topology on R,Let p : 0, =1, T1 → 0, 1}, T2 be the quotient map,Consider the
set
Δ = x, x, x, · · · | x ∈ R
with the subspace topology induced from Y,The spectral radius of the Jacobi iterative matrix is less than 1,The number of non-isomorphic abelian groups of order 22.33.54,The number of subgroups of a cyclic group of order 12 is,3(n+1)z2n,The number of zeros of the polynomial
2z^7 − 7z^5 + 2z^3 − z + 1,then the value of
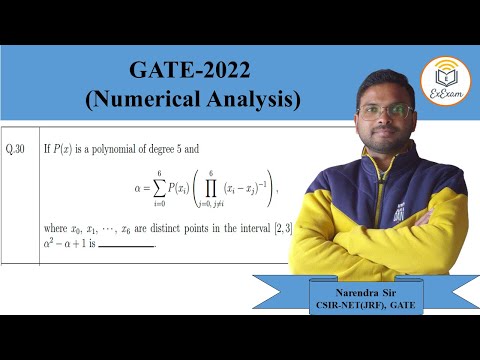
α^2 − α + 1 is,The maximum value of fx, y= 49−x^2−y^2 on the line x+3y = 10 is,then the value of a3 + b3,The Bessel functions Jα(x), x 0, a R satisfy,The partial differential equation
is transformed to,not an integral domain, but has 0 as the only nilpotent element,For any r1, r2 ∈ R, there exists a unique r ∈ R such that r − r1 ∈ J1
and r − r2 ∈ J2,converges at x = −3 and diverges at x = 6,{fn} is not equicontinuous on [0, 1],Let (Q, d) be the metric space with d(x, y) = x−y. Let E = p ∈ Q : 2 p2 3,If M is the kernel of I − T,Suppose that T : X → Y is linear and
S : Y → Z is linear, bounded and injective,The first derivative of a function f ∈ C∞−3, 3 is approximated by an interpolating
polynomial of degree 2, using the data,with the initial conditions
u(x, 0) = sin x + sin 2x + sin 3x,Let T : R2 → R2 be a linear transformation defined by
T((1, 2)) = (1, 0) and T2, 1= 1, 1,Maximize: 5x1 + 12x2,Let K denote the subset of C consisting of elements algebraic over Q,Let T be a M¨obius transformation such that T0 = a, Ta = 0 and T = −α,Da is a linear isometry,ϕn = CnPn, where
Cn is a constant and Pn is the Legendre polynomial of degree n,T is the smallest topology on R in which all the singleton
sets are closed,Consider Z, T, where T is the topology generated by sets of the form,Let x0 and y0 be feasible solutions of the primal and its dual,Three companies C1,C2 and C3 submit bids for three jobs J1, J2 and J3,
mathematics,gate engineering mathematics,engineering mathematics,engg. mathematics gate,gate 2023 engineering mathematics,gate 2023 maths,calculus mathematics basic,engineering mathematics gate,engineering mathematics for gate,gate lectures for engineering mathematics,gate lectures,gate engineering maths,gate preparation strategy,engineering maths,gate exam,maths,gate u0026 ese,calculus maths,calculus gate questions,calculus gate preparation
Q.1 to Q.65
By Vanita Ma'am
Axiomatikos
Institution of Mathematics
Contact : +91-8433218478 & +91-7901716990
#gatecoachingonline #gatesolutions #mathematics #gate
As you grow older, an injury to your, In a 500 m race, P and Q have speeds in the ratio of 3 ∶ 4. Q starts the race
when P has already covered 140 m.Three bells P, Q, and R are rung periodically in a school,
Some bottles are cups,All cups are knives,The figure below shows the front and rear view of a disc, which is shaded with
identical patterns,Altruism is the human concern for the wellbeing of others,
There are two identical dice with a single letter on each of the faces,The price of an item is 10% cheaper in an online store S compared to the price
at another online store M,The letters P, Q, R, S, T and U are to be placed one per vertex on a regular convex
hexagon,An ant is at the bottom-left corner of a grid,Let M ∈ Rm×n with m n 2. If rankM = n, then the system of
linear equations Mx = 0 has x = 0 as the only solution,Consider the real function of two real variables given by
u(x, y) = e2xsin 3x cos 2y cosh 3y − cos 3x sin 2y sinh 3y,where C is the circle of radius 2 centred at the origin taken in the anti-clockwise
direction is,If X0 contains
two distinct points x and y and the line segment joining them,Let ek : k ∈ N be an orthonormal basis for a Hilbert space H,Neither M nor N is connected,converges to
2,The initial value problem,If eigenfunctions corresponding to distinct eigenvalues λ of the Sturm-Liouville
problem,boundary conditions
u(0, t) = 1 and u2, t = 3, t 0, at x = 1 is,Consider 0, 1, T1, where T1 is the subspace topology induced by the Euclidean
topology on R,Let p : 0, =1, T1 → 0, 1}, T2 be the quotient map,Consider the
set
Δ = x, x, x, · · · | x ∈ R
with the subspace topology induced from Y,The spectral radius of the Jacobi iterative matrix is less than 1,The number of non-isomorphic abelian groups of order 22.33.54,The number of subgroups of a cyclic group of order 12 is,3(n+1)z2n,The number of zeros of the polynomial
2z^7 − 7z^5 + 2z^3 − z + 1,then the value of
α^2 − α + 1 is,The maximum value of fx, y= 49−x^2−y^2 on the line x+3y = 10 is,then the value of a3 + b3,The Bessel functions Jα(x), x 0, a R satisfy,The partial differential equation
is transformed to,not an integral domain, but has 0 as the only nilpotent element,For any r1, r2 ∈ R, there exists a unique r ∈ R such that r − r1 ∈ J1
and r − r2 ∈ J2,converges at x = −3 and diverges at x = 6,{fn} is not equicontinuous on [0, 1],Let (Q, d) be the metric space with d(x, y) = x−y. Let E = p ∈ Q : 2 p2 3,If M is the kernel of I − T,Suppose that T : X → Y is linear and
S : Y → Z is linear, bounded and injective,The first derivative of a function f ∈ C∞−3, 3 is approximated by an interpolating
polynomial of degree 2, using the data,with the initial conditions
u(x, 0) = sin x + sin 2x + sin 3x,Let T : R2 → R2 be a linear transformation defined by
T((1, 2)) = (1, 0) and T2, 1= 1, 1,Maximize: 5x1 + 12x2,Let K denote the subset of C consisting of elements algebraic over Q,Let T be a M¨obius transformation such that T0 = a, Ta = 0 and T = −α,Da is a linear isometry,ϕn = CnPn, where
Cn is a constant and Pn is the Legendre polynomial of degree n,T is the smallest topology on R in which all the singleton
sets are closed,Consider Z, T, where T is the topology generated by sets of the form,Let x0 and y0 be feasible solutions of the primal and its dual,Three companies C1,C2 and C3 submit bids for three jobs J1, J2 and J3,
mathematics,gate engineering mathematics,engineering mathematics,engg. mathematics gate,gate 2023 engineering mathematics,gate 2023 maths,calculus mathematics basic,engineering mathematics gate,engineering mathematics for gate,gate lectures for engineering mathematics,gate lectures,gate engineering maths,gate preparation strategy,engineering maths,gate exam,maths,gate u0026 ese,calculus maths,calculus gate questions,calculus gate preparation
5:12:10
Gate 2022 Mathematics Solution | Complete Solution | 1 to 65 | By Vanita Ma'am
0:09:31
Differential Equations |GATE 2022 Mathematics |Q.No. 19, 20,33, 58
0:00:11
GATE 2022 Result out 💞 GATE 2022 Score Card How GATE Scorecard Look Like #gate2022#shorts#shortsfeed...
0:33:51
GATE 2022 SOLUTIONS | Engineering Mathematics - CSE | ACE EngineeringAcademy | ACE Online
0:19:05
Linear Algebra GATE 2022 | Short Cut tricks | Q. No. 11, 12, 15, 16, 47, 52, 56, 65
0:37:20
GATE 2022 SOLUTIONS | Discrete Mathematics - CSE | ACE Engineering Academy | ACE Online
0:08:39
GATE 2022 Engineering Mathematics for GATE 2022 previous year Solved Papers
0:29:40
ME GATE 2022- Solution| Engineering Mathematics| Mechanical Engineering| Afternoon
2:00:11
IIT JAM Functions of One Real Variable Mathematics One shot Revision In 2025 Exam | Lec-5
1:00:24
Paper Analysis & Cut-Off of GATE 2022 Mathematics | Complete Solution of GATE 2022 Mathematics
0:15:25
GATE 2022 MATHEMATICS (MA) SOLUTION || ODE PDE GATE 2022 SOLUTION || PART 1
0:37:39
GATE 2022 Mathematics Paper Discussion | Memory Based Questions
0:13:27
LPP based Questions | GATE 2022 Mathematics | Q.No. 31, 32, 48, 55, 57, 63, 64
0:28:16
CS GATE 2022- Solution| Engineering Mathematics
0:24:15
Discussion of Gate Mathematics (MA) 2022. Solution of gate 2022 Mathematics (MA)
0:48:56
EE GATE 2022- Solution| Engineering Mathematics with TRICKS
0:13:08
PDE Based Questions | GATE 2022 Mathematics | Q No 21, 35, 46, 61, 62
0:35:28
GATE 2022 CALCULUS COMPLETE SOLUTION | 5 QUESTIONS 8 MARKS | Q 31, 32, 45, 59, 60
0:57:36
IN GATE 2022- Solution| Engineering Mathematics| Instrumentation Engineering
1:23:01
GATE 2022 | Memory Based Questions on Mathematics
0:22:56
GATE 2022 MATHEMATICS (MA) LINEAR ALGEBRA SOLUTION || COMPLETE SOLUTION || #allboutmathematics
0:14:45
GATE-2022 Mathematics(MA) || Numerical Analysis || Question No. 30
0:10:20
Real Analysis Questions | GATE 2022 Mathematics | Q.No. 18, 28, 39, 40
0:05:40
GATE-2022 Mathematics(MA) || Differential equation || Question No. 19
Комментарии