filmov
tv
Sums of triangular numbers and sums of squares
Показать описание
Amir Akbary, University of Lethbridge
Fields Number Theory Seminar
Date and Time: Monday, March 8, 2021 - 12:00pm to 1:00pm
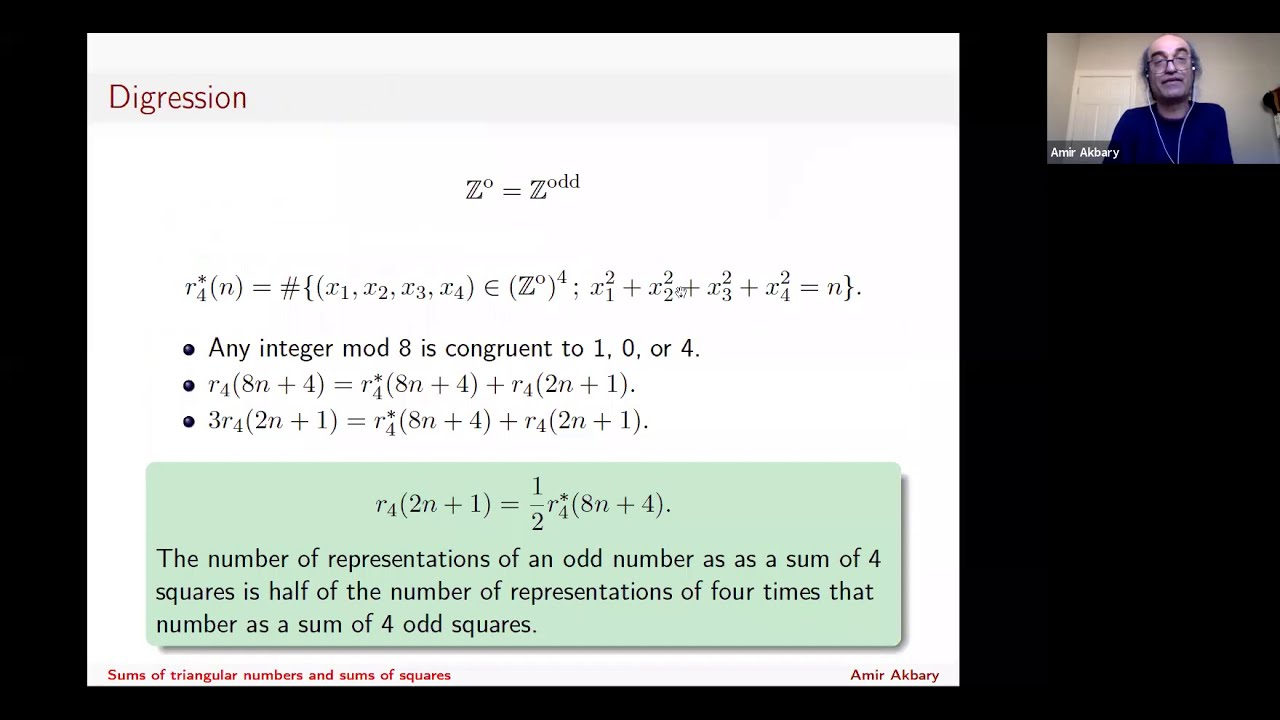
Abstract: For non-negative integers $a$, $b$, and $n$, let $t(a,b;n)$ be the number of representations of $n$ as a sum of triangular numbers with coefficients $1$ and $3$ ($a$ of ones and $b$ of threes) and let $r(a, b; n)$ be the number of representations of $n$ as a sum of squares with coefficients $1$ and $3$ ($a$ of ones and $b$ of threes). It is known that for $a$ and $b$ satisfying $1\leq a+3b \leq 7$, we have $$ t(a,b;{n}) = \frac{2}{2+{a\choose4}+ab} r(a,b;8n+a+3b) $$ and for $a$ and $b$ satisfying $a+3b=8$, we have $$ t(a,b;{n}) = \frac{2}{2+{a\choose4}+ab} \left( r(a,b;8n+a+3b) - r(a,b; (8n+a+3b)/4) \right). $$ Such identities are not known for $a+3b['larger than']8$.
Such identities are not known for $a+3b['larger than']8$. We report on our joint work with Zafer Selcuk Aygin (University of Calgary) in which, for general $a$ and $b$ with $a+b$ even, we prove asymptotic equivalence of formulas similar to the above, as $n\rightarrow\infty$. One of our main results extends a theorem of Bateman, Datskovsky, and Knopp where the case $b=0$ and general $a$ was considered. Our approach is different from Bateman-Datskovsky-Knopp's proof where the circle method and singular series were used. We achieve our results by explicitly computing the Eisenstein components of the generating functions of $t(a,b;n)$ and $r(a,b;n)$.
Fields Number Theory Seminar
Date and Time: Monday, March 8, 2021 - 12:00pm to 1:00pm
Abstract: For non-negative integers $a$, $b$, and $n$, let $t(a,b;n)$ be the number of representations of $n$ as a sum of triangular numbers with coefficients $1$ and $3$ ($a$ of ones and $b$ of threes) and let $r(a, b; n)$ be the number of representations of $n$ as a sum of squares with coefficients $1$ and $3$ ($a$ of ones and $b$ of threes). It is known that for $a$ and $b$ satisfying $1\leq a+3b \leq 7$, we have $$ t(a,b;{n}) = \frac{2}{2+{a\choose4}+ab} r(a,b;8n+a+3b) $$ and for $a$ and $b$ satisfying $a+3b=8$, we have $$ t(a,b;{n}) = \frac{2}{2+{a\choose4}+ab} \left( r(a,b;8n+a+3b) - r(a,b; (8n+a+3b)/4) \right). $$ Such identities are not known for $a+3b['larger than']8$.
Such identities are not known for $a+3b['larger than']8$. We report on our joint work with Zafer Selcuk Aygin (University of Calgary) in which, for general $a$ and $b$ with $a+b$ even, we prove asymptotic equivalence of formulas similar to the above, as $n\rightarrow\infty$. One of our main results extends a theorem of Bateman, Datskovsky, and Knopp where the case $b=0$ and general $a$ was considered. Our approach is different from Bateman-Datskovsky-Knopp's proof where the circle method and singular series were used. We achieve our results by explicitly computing the Eisenstein components of the generating functions of $t(a,b;n)$ and $r(a,b;n)$.
 0:02:13
0:02:13
 0:01:52
0:01:52
 0:00:35
0:00:35
 0:00:34
0:00:34
 0:03:20
0:03:20
 0:04:16
0:04:16
 0:02:04
0:02:04
 0:51:51
0:51:51
 2:03:11
2:03:11
 0:03:13
0:03:13
 0:07:21
0:07:21
 0:07:43
0:07:43
 0:01:46
0:01:46
 0:02:18
0:02:18
 0:00:46
0:00:46
 0:11:34
0:11:34
 0:26:14
0:26:14
 0:01:17
0:01:17
 0:02:17
0:02:17
 0:13:53
0:13:53
 0:01:41
0:01:41
 0:00:14
0:00:14
 0:02:56
0:02:56
 0:01:01
0:01:01