filmov
tv
Galois theory: Frobenius automorphism

Показать описание
This lecture is part of an online graduate course on Galois theory.
We show that the Frobenius automorphism of a finite field an sometimes be lifted to characteristic 0. As an example we use the Frobenius automorphisms of Q[i] to prove that -1 i a square mod an odd prime p if and only if p is 1 mod 4.
We show that the Frobenius automorphism of a finite field an sometimes be lifted to characteristic 0. As an example we use the Frobenius automorphisms of Q[i] to prove that -1 i a square mod an odd prime p if and only if p is 1 mod 4.
Galois theory: Frobenius automorphism
Galois 10
Frobenius Elements of the Absolute Galois Group
GALOIS THEORY||AUTOMORPHISM GROUPS||FIXED FIELDS|| M.SC MATHEMATICS
Example 48.17 and Frobenius Automorphism || Abstract Algebra 2 || Second Semester MSc Mathematics
Frobenius Endomorphism
Finite fields via Galois theory
Frobenius Automorphism and Quantum Cyclic Codes - Prof. Piyush P. Kurur
Field Theory - Automorphisms Groups of Fields/Galois Groups - Lecture 17
Constructing Finite Fields
Galois theory: Cyclotomic polynomials
Week 5-Lecture 24
431 — 06: Field Automorphisms and the Galois Group
Finite Fields
Lecture 8. Finite Fields
Galois theory: Cubics and quartics
Galois theory: Infinite Galois extensions
Galois Theory Lecture 1: Automorphism Group of a Field
The Frobenius Endomorphism Part 2
What is...Frobenius’ density theorem?
Galois theory Lecture -2
The Hopf category of Frobenius algebras
Galois group
'Galois Theory and Profinite Groups' (Part. 5/8) by Prof. Peter Stevenhagen
Комментарии
 0:21:03
0:21:03
 0:11:18
0:11:18
 0:09:09
0:09:09
 0:27:55
0:27:55
 0:31:54
0:31:54
 0:05:01
0:05:01
 0:20:17
0:20:17
 0:50:10
0:50:10
 0:26:55
0:26:55
 0:10:45
0:10:45
 0:22:18
0:22:18
 0:29:17
0:29:17
 0:30:35
0:30:35
 0:42:05
0:42:05
 0:34:19
0:34:19
 0:17:37
0:17:37
 0:36:31
0:36:31
 0:20:52
0:20:52
 0:13:18
0:13:18
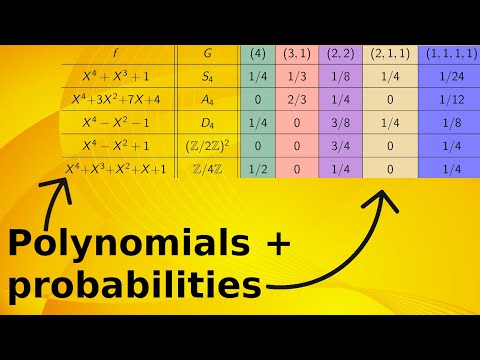 0:28:34
0:28:34
 0:55:07
0:55:07
 0:49:05
0:49:05
 0:03:52
0:03:52
 0:52:34
0:52:34