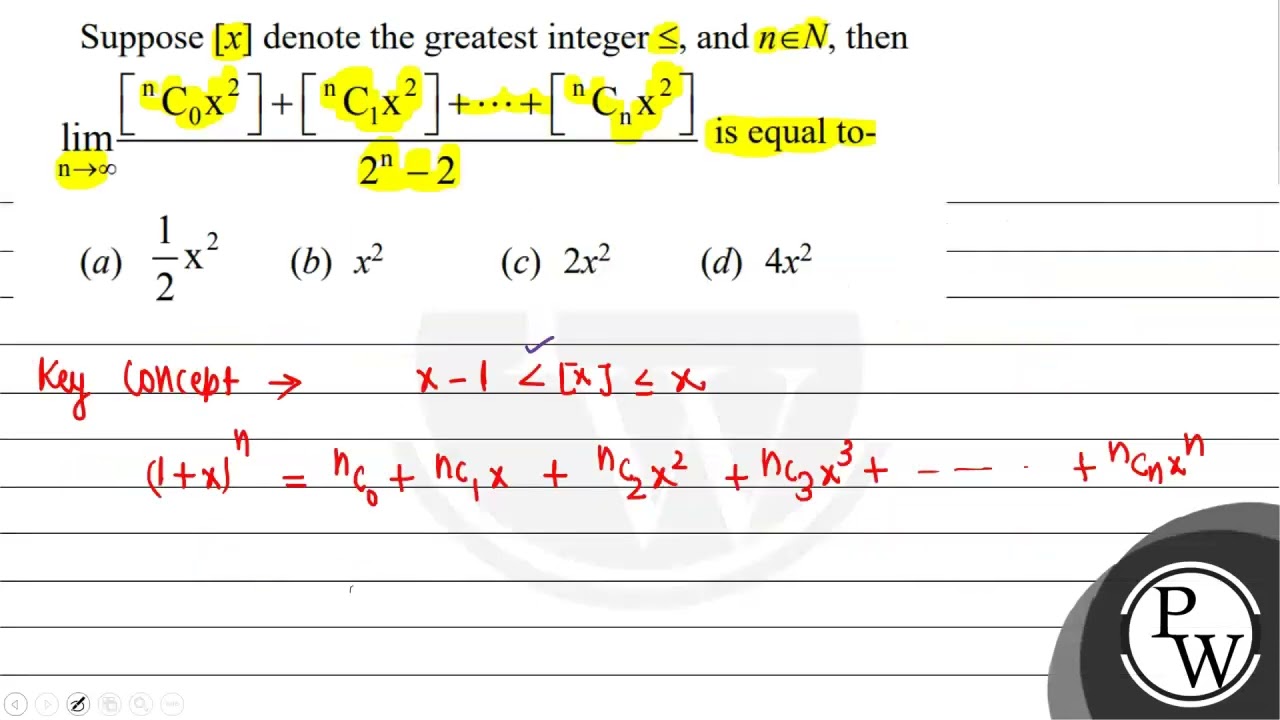
Suppose \( [x] \) denote the greatest integer \( \leq \), and \( n \in N \), then \( \lim _{n \r...

Suppose \( [x] \) denote the greatest integer \( \leq \), and \( n \in N \), then \( \lim _{n \rightarrow \infty} \frac{\left[{ }^{n} C_{0} x^{2}\right]+\left[{ }^{n} C_{1} x^{2}\right]+\cdots+\left[{ }^{n} C_{n} x^{2}\right]}{2^{n}-2} \) is equal to-
(a) \( \frac{1}{2} \mathrm{x}^{2} \)
(b) \( x^{2} \)
(c) \( 2 x^{2} \)
(d) \( 4 x^{2} \)