Geometry - Ch. 6: Circles (19 of 39) Tangent Lines: Another Example

To donate:

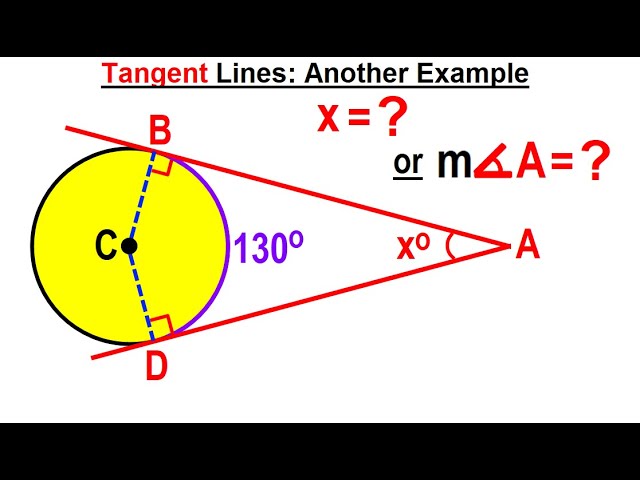
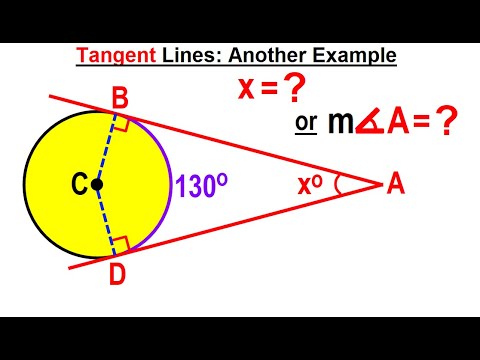
We will find x=?, an external angle that is outside of a circle, then the measure of the angle is ½ (half) the measure of the intercepted arc.

Title search of video on other plateforms: Geometry - Ch. 6: Circles (18 of 39) Tangent Lines

Title search of video on other plateforms: Geometry - Ch. 6: Circles (20 of 39) Angles Exterior to the Circle

[surendrakverma]

Excellent explanation Sir. Thanks 🙏🙏🙏🙏🙏🙏

[shannonmcdonald]

For whatever reason I was able to look at the problem and add 90+90+130 and subtract that total, 310 from 360 to arrive at 50° for x. Intuitively it made sense.
I have no geometry education.

[OLORDJESUS]

Hi Michel, could you please make a video on this viral age problem:
Mary is 24 years old. Mary is twice as old as Ann was when Mary was as old as Ann is now. How old is Ann?

[The_Green_Man_OAP]

Can you project the circumference of a circle onto a line that goes through the circle's origin and intercepts a tangent line or secant of that circle? (Similar to what you have depicted here)

[Gbhmagic]

weird.. i divided the 130/2 got 65.. 65-90=25 since we divided by 2 we multiply by 2 and get 50... I just drew a line from C to A creating 2 right triangles...