11 The excision axiom

This is episode 11 of a course on algebraic topology.
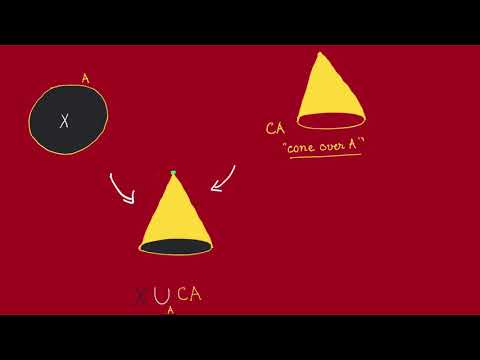
We motivate the proof of the excision axiom for singular homology. Proof details are given modulo a proof of the lemma of small simplices.

Outline:
00:00 Start
02:26 Def.: 𝒰-small
07:32 Lemma of small simplices
10:35 Proof of excision axiom based on the lemma of simplices
25:06 Idea of the lemma of small simplices

[mahhagogo]

At 16:50

Can use isomorphism theorem B (for modules):


Let M be a module, and let S and T be submodules of M. Then:

The sum S + T = {s + t | s ∈ S, t ∈ T} is a submodule of M,
The intersection S ∩ T is a submodule of M, and
The quotient modules (S + T) / T and S / (S ∩ T) are isomorphic.

[beback_]

15:40 Minor correction: Roman writes C_*(X/A) but he means C_*(X \setminus A)

[mrfladoodl]

What guarantees that the procedure described in minute 37 can have a finite number of steps? Lebesgue's covering lemma does