filmov
tv
Visual Proof: Natural Numbers Formula
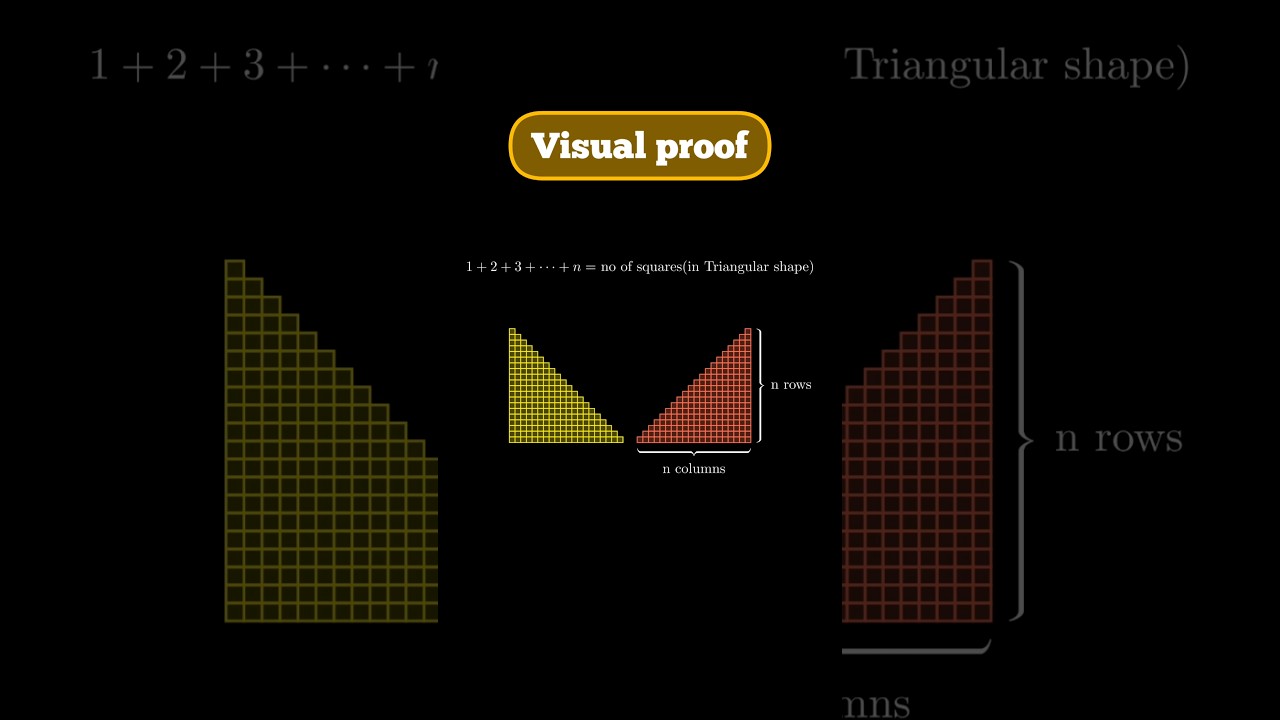
Показать описание
Discover the fascinating world of mathematics with MindSphere! In this maths shorts video, we'll take you on a visual journey to prove the formula for the sum of the first n natural numbers. From the fundamental principles to the stunning revelation, we'll break it down in an easy-to-understand and visually engaging way. Get ready to have your mind blown by the simplicity and beauty of mathematics!
#maths shorts, #MindSphere, mathematics, sum of first n natural numbers, formula, #visual proof
#maths shorts, #MindSphere, mathematics, sum of first n natural numbers, formula, #visual proof
Visual Proof: Natural Numbers Formula
Sum of n squares | explained visually |
Sum of n natural numbers | Visual Proof | MathVsScience | math
Summing Squares Geometrically
Sum of n natural numbers - visual proof
Visual Proof for the Sum of the First n Natural Numbers
A Dozen Proofs: Sum of Integers Formula (visual proofs) #SoME2
Sum of the First N Natural Numbers | Formula and Proof
Visual Algebra, Lecture 2.5: Groups of permutations
Visual Proofs - 1 : Sum of Natural Numbers
Products of Odd Squares and Triangular Numbers (visual proof - updated version)
Visual Cube Sums! (from Nicomachus)
Visual Proof for Sum of Squares Formula #SoME3
Visual proof for sum of natural numbers
Sum of n natural numbers (Visual proof) 🔥🔥
What's this infinite sum?
Finding the general formula for nth octagonal number | Visual proof |
Sum of first n natural numbers - an easy & intuitive proof
Sum of Squares I (visual proof)
Sum of first n natural numbers: A Visual Proof.
See Why 1+2+3+...+n = n(n+1)/2 Makes Sense | Sum of N natural numbers (Visual Proof)😮😲😱
Visual proof of sum of n integers | science.short | EP02
The Sum of Natural Numbers - A Visual Explanation and A Proof #mathematics #sumformula #geometry
sum of natural numbers | sum of natural numbers formula proof | Visualization + Proof
Комментарии
 0:00:36
0:00:36
 0:02:14
0:02:14
 0:00:57
0:00:57
 0:00:56
0:00:56
 0:02:10
0:02:10
 0:01:08
0:01:08
 0:20:58
0:20:58
 0:02:08
0:02:08
 0:44:42
0:44:42
 0:00:39
0:00:39
 0:01:33
0:01:33
 0:00:50
0:00:50
 0:05:53
0:05:53
 0:01:52
0:01:52
 0:00:57
0:00:57
 0:00:31
0:00:31
 0:01:34
0:01:34
 0:01:22
0:01:22
 0:01:38
0:01:38
 0:05:03
0:05:03
 0:00:35
0:00:35
 0:00:50
0:00:50
 0:01:26
0:01:26
 0:00:51
0:00:51