filmov
tv
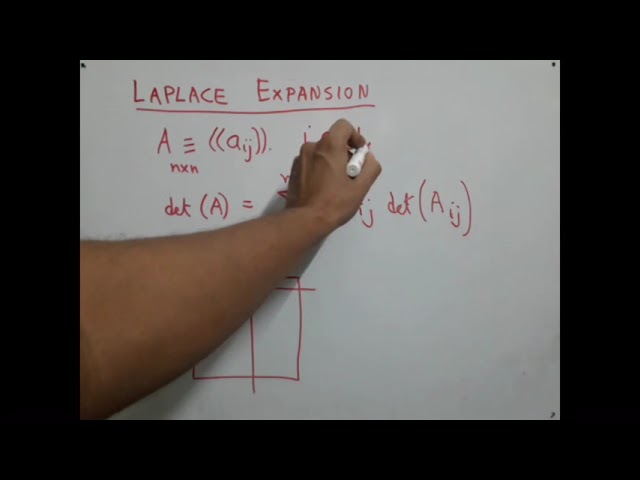
Laplace expansion: A simple, direct proof
Показать описание
This video is based on my book on Linear Algebra, and not on the book by Rao and Bhimasankaram.
Laplace expansion: A simple, direct proof
Laplace expansion for computing determinants | Lecture 29 | Matrix Algebra for Engineers
Laplace Expansion
21. MFE: Laplace Expansion
Cofactors and Laplace Expansion
3x3 Determinants - The Laplace Expansion
Determinants : Laplace Expansion/Co-factor (Linear Algebra)
Laplace expansion 6x6
Matrices (Determinant of 3x3 matrix using Laplace Expansion)
How To Find The Determinant of a 4x4 Matrix
Math 2.11.11. Laplace expansion of det(A)
Elon Musk Laughs at the Idea of Getting a PhD... and Explains How to Actually Be Useful!
Proof of the Cofactor Expansion Theorem
Expansion by Minors | Linear Algebra #18
Intro to the Laplace Transform & Three Examples
Beauty of the Brain😍 IQ - IIT Bombay
NEWYES Calculator VS Casio calculator
Quick determinant trick
Example 2: Finding the determinant of a matrix using cofactor expansion
How to Find The Determinant of a 4x4 Matrix (Shortcut Method)
Theorem 2.1.1 (Cofactor Expansion Theorem)
Most💯 Important Step Before any Procedure 🔥
How REAL Men Integrate Functions
Finding a determinant using cofactor expansion
Комментарии
 0:20:31
0:20:31
 0:13:10
0:13:10
 0:51:09
0:51:09
 0:39:45
0:39:45
 0:16:25
0:16:25
 0:11:42
0:11:42
 0:13:50
0:13:50
 0:29:55
0:29:55
 0:08:21
0:08:21
 0:11:29
0:11:29
 0:09:25
0:09:25
 0:00:39
0:00:39
 0:26:19
0:26:19
 0:27:18
0:27:18
 0:12:05
0:12:05
 0:00:19
0:00:19
 0:00:14
0:00:14
 0:01:13
0:01:13
 0:06:29
0:06:29
 0:05:46
0:05:46
 0:17:36
0:17:36
 0:00:16
0:00:16
 0:00:35
0:00:35
 0:07:58
0:07:58