filmov
tv
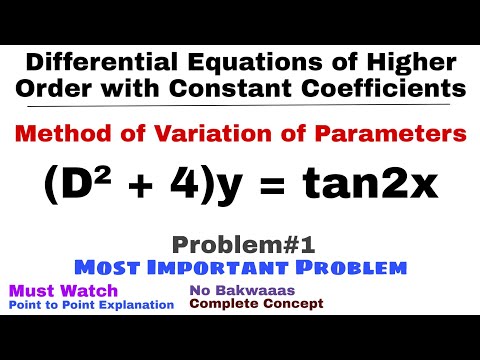
Solve d2y/dx2+4y=4tan2x
Показать описание
Variation of Parameters. The Wronskian method. Do you like this method?
How to solve a non-homogenous second order linear differential equation?
A method to skip "Let y=e^(mx)" for complementary function but it is fast and accurate.
Solve d2y/dx2+4y=0 by inserting the dummy "m", fast and accurate. The complementary function for the associated homogenous equation, a faster way.
This method is applicable for all homogenous second order linear differential equations.
Have no ideas on what is the general function to be used for the particular integral? The Wronskian determinant could help!
General form of yp=A(x)u1(x) + B(x)u2(x) where A(x) = integral of 1/W u2(x) f(x) dx while B(x) = integral of 1/W u1(x) f(x) dx and W=determinant of [{u1(x), u2(x)}, {u'1(x), u'2(x)}].
Note that, u(x) are the functions obtained from the derived complementary function.
Welcome to join and feel free to raise/ask questions (if any) 🤗
#Differential #Equation
How to solve a non-homogenous second order linear differential equation?
A method to skip "Let y=e^(mx)" for complementary function but it is fast and accurate.
Solve d2y/dx2+4y=0 by inserting the dummy "m", fast and accurate. The complementary function for the associated homogenous equation, a faster way.
This method is applicable for all homogenous second order linear differential equations.
Have no ideas on what is the general function to be used for the particular integral? The Wronskian determinant could help!
General form of yp=A(x)u1(x) + B(x)u2(x) where A(x) = integral of 1/W u2(x) f(x) dx while B(x) = integral of 1/W u1(x) f(x) dx and W=determinant of [{u1(x), u2(x)}, {u'1(x), u'2(x)}].
Note that, u(x) are the functions obtained from the derived complementary function.
Welcome to join and feel free to raise/ask questions (if any) 🤗
#Differential #Equation
Solve d2y/dx2+4y=4tan2x
0:15:18
#MethodofVariationofParameters#(D^2+4)y=4tan2x# #DifferentialEquationsofHigherOrder#BTech#Bsc#BCA#
0:08:55
d^2y/dx^2 +4y = 4 Tan 2x || Solve Using Variation of Parameters and Wronskian || Study With Nitin
0:01:05
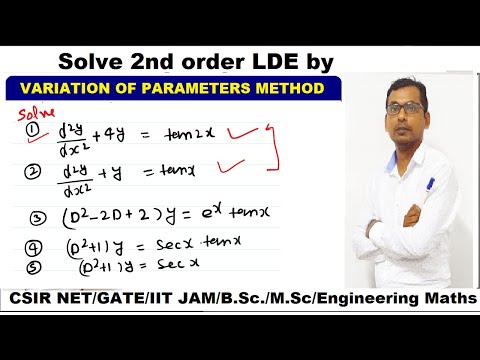
Solve by the method of variation of parameter d²y/dx² + 4y = 4 tan 2x
0:14:34
16. Method of Variation of Parameters | Problem 1 | Concept | Differential Equations of Higher Order
0:06:27
y'+4y=4tan2x Variation of Parameter of 2nd Order Differential Equation @confusedstudent
0:38:33
#14 Variation of parameters method | solve (D^2+4)y= tan 2x | (D^2+1)y=sec x tan x | (D^2+1)y=sec x
0:19:27
Variations of parameters | Example 1 | linear Equation of second order with variable coefficient
0:12:37
Solve d²y/dx²+4y=tan2x using Method of variation of parameters in Telugu
0:11:41
method of variation of parameter, solve y''+4y=sec(2x), mathematical method by s.m Yusuf
0:08:31
Solve d2y/dx2+4y=ex+sin2x+cos2x
0:07:49
Solve: (D²+4)y= sin2x
0:00:18
Variation method||Raleigh Ritz method||Ground state of Helium|Electron interaction energy#mscphysics
0:10:11
METHOD OF VARIATION OF PARAMETERS-2 IN TELUGU tan2x problem
0:06:10
Solve d2y/dx2+4dy/dx+3y=sin x
0:35:41
d^2y/dx^2-4y=xSinhx, (D^2-4)y=xSinhx #HigherOrderLinearDifferentialEquation L884
0:07:10
Solve d2y/dx2+a2y=secax
0:11:14
Wronskian method for variations of parameters | Examples 1 | Alternate method to find C S
0:07:02
@btechmathshub7050(D²+4)y=sec2x Method of variation of parameters
0:16:04
Method of variation of parameters | VTU Qp problems | 18MAT21 | Part 2
0:00:20
Solve 𝑦𝑒 𝑥𝑑𝑥 + (2𝑦 + 𝑒 𝑥)𝑑𝑦 = 0
0:02:19
4.3-5 Equações lineares homogêneas com coeficientes constantes
0:09:16
#37 || Problem#2 || (𝒅^𝟐 𝒚)/〖𝒅𝒙〗^𝟐 +𝒚=𝒕𝒂𝒏𝒙 || Method of variation parameter || 18MAT21 || BMATC/M101...
0:09:14
Solve d2y/dx2+3dy/dx+2y=sinh(2x+3)
Комментарии