filmov
tv
Equivalence Relations
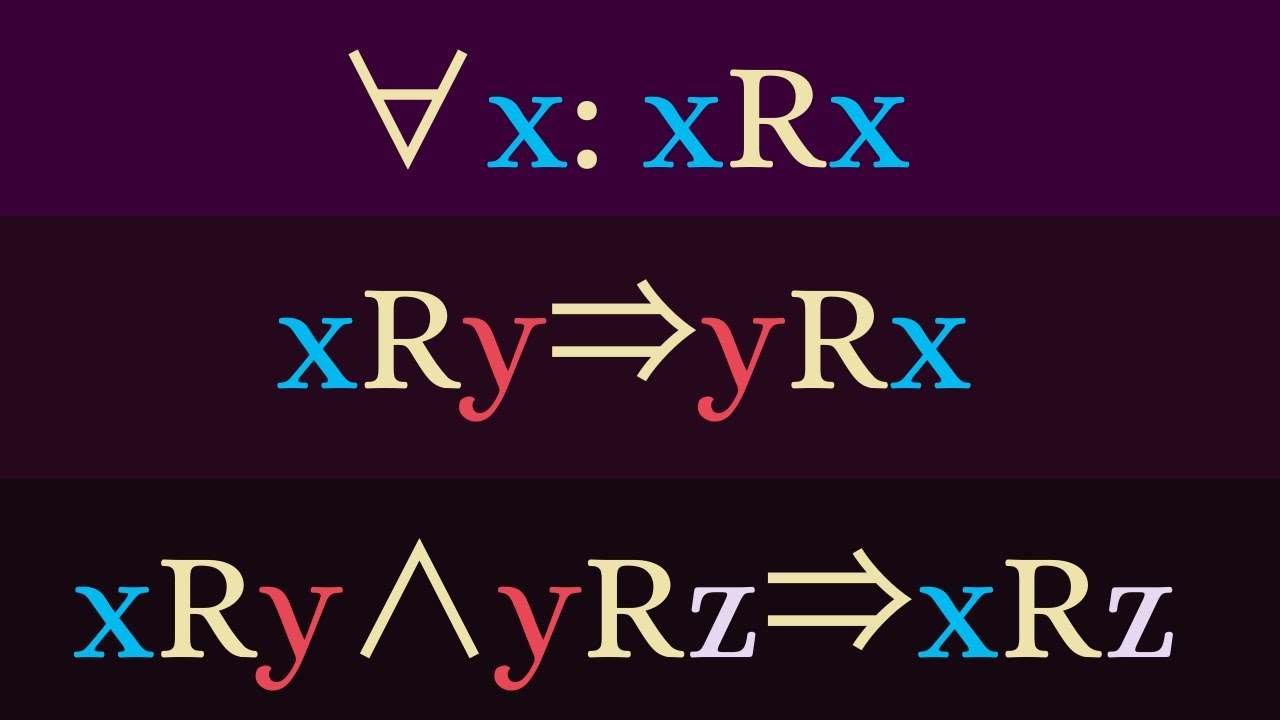
Показать описание
We look at the notion of an equivalence relation on a set, define an equivalence class, and consider several examples.
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
Equivalence Relations - Reflexive, Symmetric, and Transitive
Equivalence Relation
What is an Equivalence Relation? | Reflexive, Symmetric, and Transitive Properties
Discrete Math - 9.5.1 Equivalence Relations
Equivalence Relations Definition and Examples
Equivalence relations made easy
Equivalence Classes
Equivalence Relations!
Equivalence Class: Result, Properties, and Examples | Group Theory Lecture 02 (III) by Dubey Sir
Proving a Relation is an Equivalence Relation | Example 2
(Abstract Algebra 1) Partitions and Equivalence Relations
Abstract Algebra | Partitions and Equivalence Relations
Equivalence Relations: Sample Problems
Equivalence Relations -- Proof Writing 17
Equivalence Relation (Solved Problems)
Properties of Relations in Discrete Math (Reflexive, Symmetric, Transitive, and Equivalence)
Proving a Relation is an Equivalence Relation | Example 1
RELATIONS - DISCRETE MATHEMATICS
Equivalence Classes and Partitions
Equivalence Relations
Discrete Math 9.5.1 Equivalence Relations
Equivalence Relation in Discrete Mathematics with examples
How to Prove a Relation is an Equivalence Relation
Prove that R is an Equivalence Relation on Z: xRy if x + 3y is even
Комментарии
 0:04:36
0:04:36
 0:06:29
0:06:29
 0:05:01
0:05:01
 0:22:30
0:22:30
 0:13:01
0:13:01
 0:07:15
0:07:15
 0:07:19
0:07:19
 0:17:20
0:17:20
 0:51:00
0:51:00
 0:11:20
0:11:20
 0:06:35
0:06:35
 0:14:38
0:14:38
 0:07:48
0:07:48
 0:34:52
0:34:52
 0:11:06
0:11:06
 0:16:46
0:16:46
 0:14:56
0:14:56
 0:15:36
0:15:36
 0:13:10
0:13:10
 0:19:44
0:19:44
 0:17:26
0:17:26
 0:06:12
0:06:12
 0:08:18
0:08:18
 0:10:10
0:10:10