Reflection Across The Y Axis

Quickly review how to reflect an object across the y-axis. This video provides a simple rule for reflection across the y-axis.

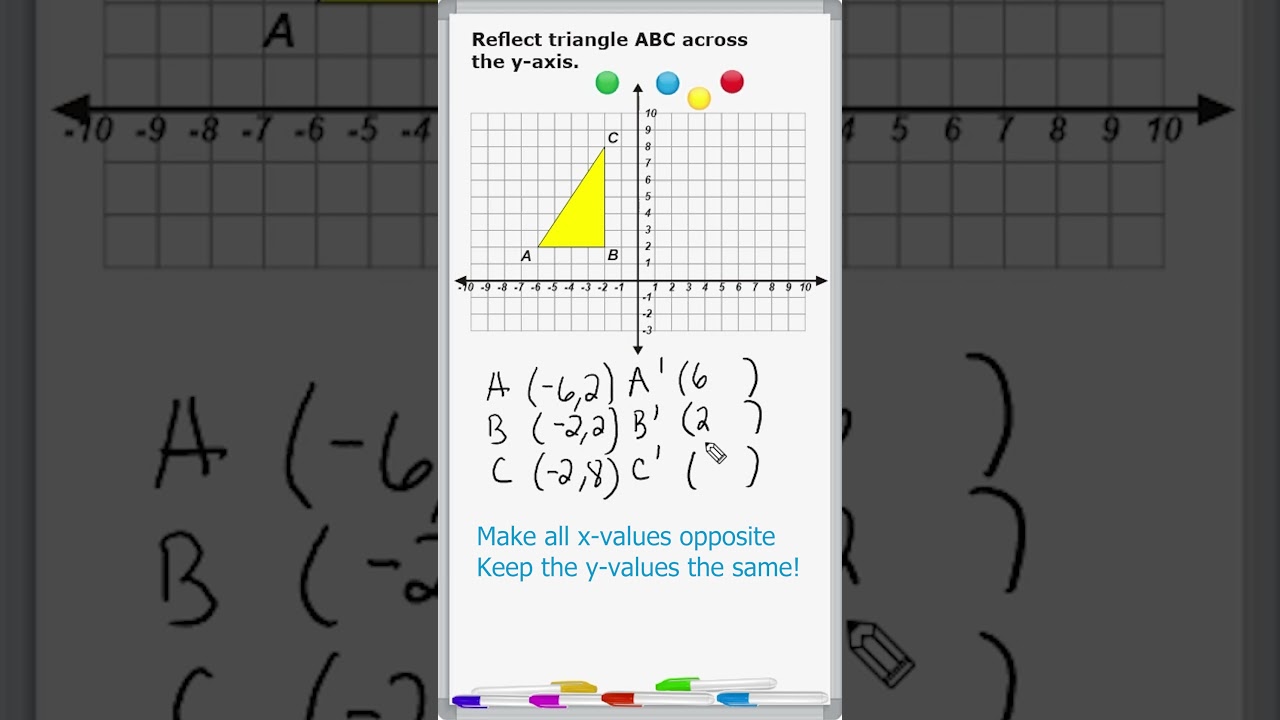
To reflect a triangle across the y-axis, you simply need to change the sign of the x-coordinate of each point while keeping the y-coordinate unchanged. Here are the steps required to reflect the triangle with points A(-6,2), B(-2,2), and C(-2,8) across the y-axis:

1. Identify the Original Triangle: Start by identifying the vertices of the original triangle on the coordinate plane. In this case, the vertices are A(-6,2), B(-2,2), and C(-2,8).

2. Reflect Each Point Across the Y-Axis: To reflect each point across the y-axis, simply change the sign of the x-coordinate while keeping the y-coordinate unchanged. 
   - For point A(-6,2), the reflected point will be A'(6,2).
   - For point B(-2,2), the reflected point will be B'(2,2).
   - For point C(-2,8), the reflected point will be C'(2,8).

3. Plot the Reflected Triangle: Plot the reflected points A'(6,2), B'(2,2), and C'(2,8) on the coordinate plane. Connect these points to form the reflected triangle.

4. Verify: Check that the reflected triangle appears symmetrical with respect to the y-axis. The distances between corresponding vertices of the original and reflected triangles should be the same, and the orientation of the triangle should be opposite.

Following these steps, you can accurately reflect the given triangle across the y-axis on the coordinate plane.

#transformations #coordinategeometry #maths