filmov
tv
Learn to identify if the discontinuity is a hole or asymptote
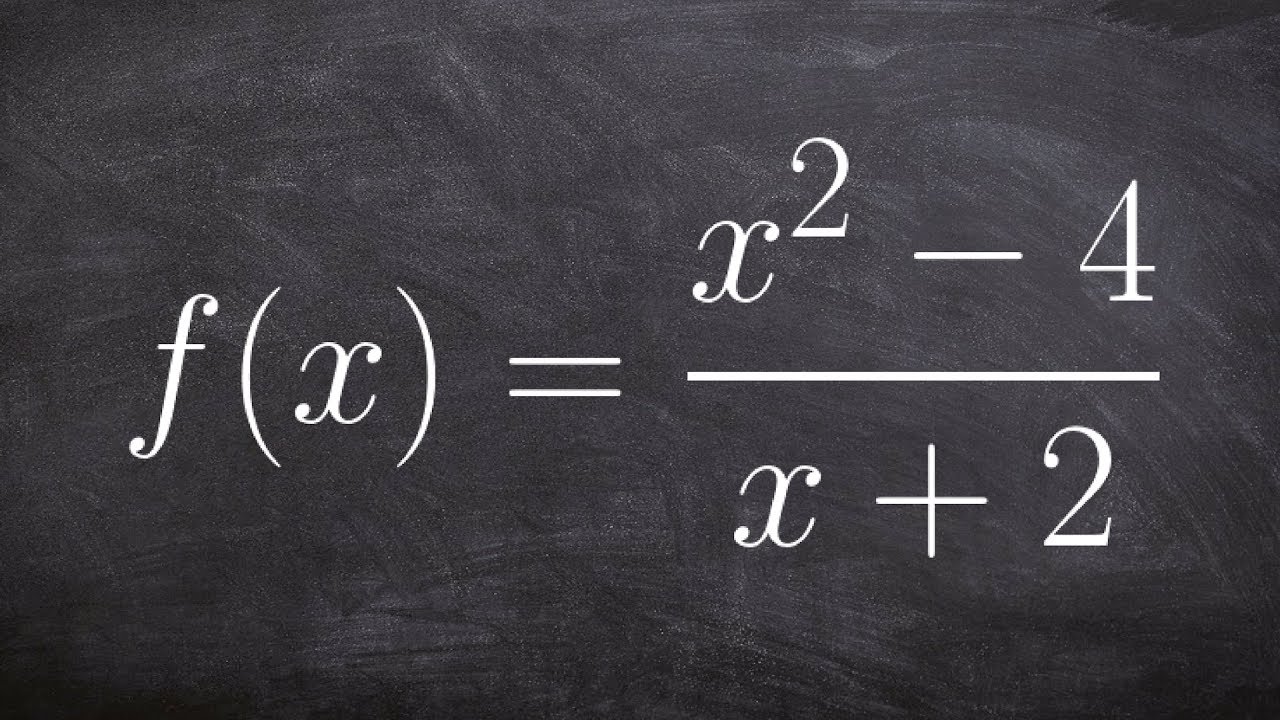
Показать описание
👉 Learn how to find the removable and non-removable discontinuity of a function. A function is said to be discontinuous at a point when there is a gap in the graph of the function at that point. A discontinuity is said to be removable when there is a factor in the numerator which can cancel out the discontinuous factor and is said to be non-removable when there is no factor in the numerator which can cancel out the discontinuous factor.
To find the discontinuities of a rational function, it is usually useful to factor the expressions in the function and we then set the denominator equal to 0 and solve for x. The value of x for which the factor appears in both the numerator and the denominator is the point of removable discontinuity while the value of x for which the factor appears in only the denominator is the point of non-removable discontinuity.
Organized Videos:
✅ Find the Asymptotes of Rational Functions
✅ Find the Vertical and Horizontal Asymptotes of a Rational Function y=0
✅ Asymptotes of Rational Functions | Learn About
✅ Find the Asymptotes of a Rational Function with Trig
✅ Find the Asymptotes and Holes of a Rational Function
✅ Find the Slant Asymptotes of the Rational Function
Connect with me:
#asymptotes #functions #brianmclogan
To find the discontinuities of a rational function, it is usually useful to factor the expressions in the function and we then set the denominator equal to 0 and solve for x. The value of x for which the factor appears in both the numerator and the denominator is the point of removable discontinuity while the value of x for which the factor appears in only the denominator is the point of non-removable discontinuity.
Organized Videos:
✅ Find the Asymptotes of Rational Functions
✅ Find the Vertical and Horizontal Asymptotes of a Rational Function y=0
✅ Asymptotes of Rational Functions | Learn About
✅ Find the Asymptotes of a Rational Function with Trig
✅ Find the Asymptotes and Holes of a Rational Function
✅ Find the Slant Asymptotes of the Rational Function
Connect with me:
#asymptotes #functions #brianmclogan
0:01:24
Learn to identify if the discontinuity is a hole or asymptote
0:08:42
How To Identify If You're Truly Learning Holistically
0:04:57
Learn how to identify if a function is a polynomial and identify the degree and LC
0:04:16
Ask a French Teacher #1 - How Can I Tell if a Noun is Masculine or Feminine?
0:06:25
Learn how to determine if there are 1 2 or no triangles
0:00:55
Please learn this if you haven't already.
0:02:37
Learn how to determine if a piecewise function is continuous and differentiable
0:00:27
What to Do if You Didn’t Study
0:33:51
If You're Happy and You Know It Dance! | @CoComelon | Learning Videos For Toddlers
0:07:28
Watch This If You Don't Know What To Study In University
0:02:21
If you're struggling to learn to code, you must watch this
0:01:47
If You Know All the Seasons - The Kiboomers Preschool Learning Videos for Circle Time
0:01:32
Learn Colors with The Kiboomers - If it's Yellow and You Know It Color Song for Preschoolers
0:28:08
Learn To Act As If Nothing Bothers You - Joe Dispenza Motivation
0:09:16
How I would learn to code (If I could start over)
0:00:51
Check the link in bio if you wanna learn acro!
0:03:13
If You're Happy and You Know it | Learning with Ms Houston | Kid Songs + Nursery Rhymes
0:00:21
Check the link in bio if you wanna learn acro!
0:00:48
Check the link in bio if you wanna learn acro!
0:06:55
How I'd Learn Web Development (If I Could Start Over)
0:00:51
Check the link in bio if you wanna learn acro!
0:00:57
Check the link in bio if you wanna learn acro!
1:03:18
If You Are Happy And You Know It | Learn Nursery Rhymes by Bob The Train
0:02:47
'If you think you know everything, you can't learn anything'
Комментарии