Why Lagrangian Mechanics is BETTER than Newtonian Mechanics F=ma | Euler-Lagrange Equation | Parth G

Newtonian Mechanics is the basis of all classical physics... but is there a mathematical formulation that is better?

In many cases, yes indeed there is! Lagrangian mechanics, named after Joseph Louis Lagrange, is a formulation of classical physics that is often more convenient to use than Newtonian Mechanics.

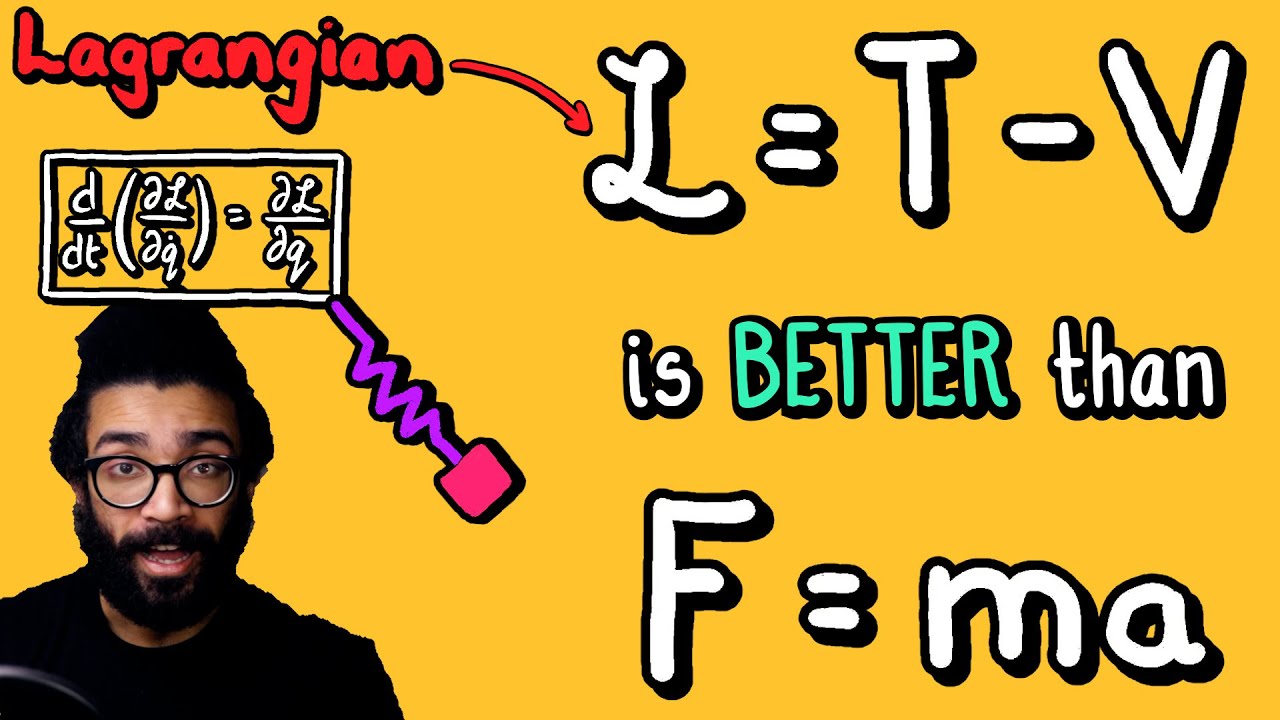
The first concept worth knowing about is that a quantity called the "Lagrangian" is defined as L = T - V where T is the kinetic energy of the system we happen to be studying, and V is the potential energy. In this video, we see how to find the Lagrangian for a simple mass-spring system by considering the mass of the block, spring constant of the spring, and motion of the entire setup. We see how to write the kinetic energy (or more specifically the speed of the setup) in terms of the distance moved by the system.

Once we have found the Lagrangian for the system, we then see that the "big boi" equation of Lagrangian mechanics is the Euler-Lagrange Equation. This is a complicated (looking) equation that allows us to substitute in the Lagrangian, and churn out an equation of motion for the system. Now the Euler Lagrange Equation (or EL Equation) is consistent with classical Newtonian mechanics - something I'd like to show in a future video. But the important thing is that there is some calculus (normal derivatives and partial derivatives) to be done, after which we will find an equation of motion.

Now for the mass-spring system, the equation of motion could have easily been found by considering the forces acting on the system (in this case the force exerted by the spring), and saying that the sum of all the forces was equal to the net force, ma. This is essentially the same as applying Newton's Second Law to our system. And in this case, using forces is MUCH simpler. So why did we go the long way and use Lagrangian mechanics?

Well, in many other scenarios, working with forces can be very complicated and fiddly. Working with energies, which is what Lagrangian mechanics does, can be much easier. Additionally, the EL Equation is very well suited to working with multiple coordinates. For example, if an object displays motion in multiple directions, such as x, y, and z, and there are different forces acting in each direction, then with Newtonian mechanics we would have to resolve all the forces in each direction. With Lagrangian mechanics, we simply find the Lagrangian and find an equation for each coordinate - x, y, and z.

That just about covers the very basics of Lagrangian mechanics, but it's worth mentioning that it goes much deeper. For example, Noether's Theorem, which talks about the fundamental link between symmetry and conservation laws (conservation of energy, conservation of momentum, etc) is based on the EL equation. It is a very interesting look at some deep universal concepts, and it's based on Lagrangian mechanics!

Thank you all so much for your wonderful support as always! Please check out my socials here:
Instagram - @parthvlogs