Using inverse properties to solve an exponential equation

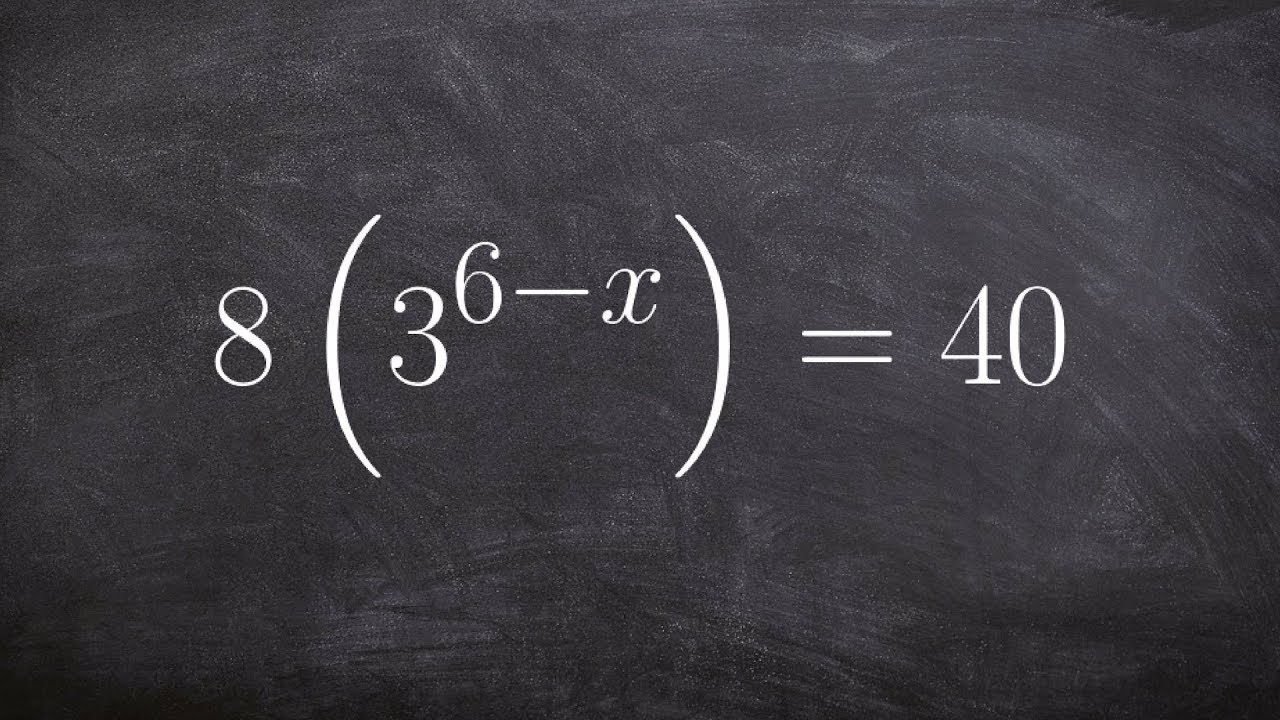
preview_player
Показать описание
👉 Learn how to solve exponential equations. An exponential equation is an equation in which a variable occurs as an exponent. To solve an exponential equation, we isolate the exponential part of the equation. Then we take the log of both sides. Note that the base of the log should correspond to the base of the exponential part of the equation, so that the log cancels out the exponential function, leaving out only the exponent.

Organized Videos:
✅Solve Exponential Equations
✅Solve Exponential Equations | Learn About
✅Solve Exponential Equations with a Calculator
✅Solve Exponential Equations with Fractions
✅Solve Exponential Equations | Easy
✅Solve Exponential Equations with e
✅Solve Exponential Equations with Logarithms
✅Solve Exponential Equations without a Calculator

Connect with me:

#brianmclogan #exponential
  1. Brian McLogan
  2. solve exponential equations
  3. solve exponential
  4. exponential equatios
  5. how to solve exponential equations
  6. fractions
Рекомендации по теме
Using inverse properties 0:06:47

Using inverse properties to solve an exponential equation

Using inverse properties 0:01:36

Using inverse properties to solve a logarithmic equation

Solving a logarithmic 0:02:00

Solving a logarithmic equation by using inverse properties

[Linear Algebra] Solving 0:11:28

[Linear Algebra] Solving with Inverses and Inverse Properties

How to Solve 0:03:26

How to Solve Algebra Equations Using Inverse Operations : Measurements & Other Math Calculations

Inverse Properties for 0:04:46

Inverse Properties for Logarithms

Inverse Property of 0:02:55

Inverse Property of Addition | Additive Inverse | Math with Mr. J

How To Find 0:11:36

How To Find The Inverse of a Function

Properties Of Numbers 0:04:08

Properties Of Numbers - Inverse Property / Maths Arithmetic

Learn How to 0:02:19

Learn How to Use Inverse Properties to Teach How to Solve a Radical Equation Root

Inverse Matrices and 0:12:00

Inverse Matrices and Their Properties

Overview of log 0:06:06

Overview of log properties - Inverse properties

How to Use 0:01:53

How to Use Inverse Operations to Isolate a Variable

Inverse Function 0:01:00

Inverse Function

Using inverse trig 0:04:06

Using inverse trig properties to solve word problems - Math help

Solving Simple Equations 0:19:50

Solving Simple Equations Using Inverse Operations | 8.EE.C.7 💗

Inverse of Logarithmic 0:13:02

Inverse of Logarithmic Functions - PreCalculus

Use inverse operation 0:02:39

Use inverse operation to solve exponential equation without one to one property

Inverse Matrices Solving 0:02:24

Inverse Matrices Solving Systems of Equations

Using inverse operations 0:03:55

Using inverse operations to help us solve exponential equations

Learn how to 0:02:03

Learn how to solve a multi step equation by using inverse operations

Introduction to Inverse 0:12:16

Introduction to Inverse Functions

How To Find 0:14:41

How To Find The Inverse of Exponential Functions

Inverse Operations Multiplication 0:06:17

Inverse Operations Multiplication And Division Year 3 | The Maths Guy

Комментарии
Автор

Uploaded 4 years ago. Helped me in 2017. Goodness thaanks

mayattv
Автор

@3:46 seconds into your video: So just to be clear, you used log_3 because the base in the problem is 3. If it had been 2^6-x, you would have used Log_2, correct?

christinagarcia