filmov
tv
Intro Stat, Lec 14B, Normal Approx to Binomial, Poisson RVs, Confidence Intervals for Pop Mean

Показать описание
(0:00) Review Normal approximation to a Binomial distribution problem from the end of Lecture 14A.
(3:32) Word of warning about whether a binomial distribution is the best model for the on-time shipping example.
(4:30) Poisson random variables (highlight the similarities and differences with binomial RVs).
(5:46) Poisson probability formula.
(6:00) Example: compute P(X = 3) when mu = mean = 1.2.
(8:25) Using =POISSON(k,mean,cumulative) on a spreadsheet.
(9:15) Compute the probability distribution on a spreadsheet.
(11:43) Compute the mean as a summation on the spreadsheet and confirm it equals the stated value of the mean mu.
(12:29) Special formulas for the mean and standard deviation that only work for Poisson RVs.
(14:00) Other slides with graphs to look at and think about. Approximate probabilities on two graphs for a Poisson distribution with mu = 1.2.
(15:21) Confidence intervals to estimate a population mean when we assume (unrealistically) that the population standard deviation is known. We are starting Chapter 6. The most important chapter: it contains the fundamentals of inferential statistics.
(19:23) The logic of confidence intervals (using the Central Limit Theorem).
(22:38) A bit of algebra to restate the probability for a 95% confidence interval.
(24:45) For more accuracy, we will use 1.96 instead of 2 as the 95% confidence interval multiplier in this chapter. Show the formula for the confidence interval in both "plus or minus" notation and in (open) "interval notation".
(25:50) Numerical example to illustrate the connection between the different notations.
(27:25) Encouragement to write answers with both notations to increase understanding. Ultimately, the calculations are not too hard, but the concept is challenging to understand.
#normalapproximation #poissondistribution #confidenceinterval
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
 0:28:00
0:28:00
 0:00:31
0:00:31
 0:33:26
0:33:26
 0:06:35
0:06:35
 0:15:20
0:15:20
 0:25:28
0:25:28
 0:31:48
0:31:48
 0:17:40
0:17:40
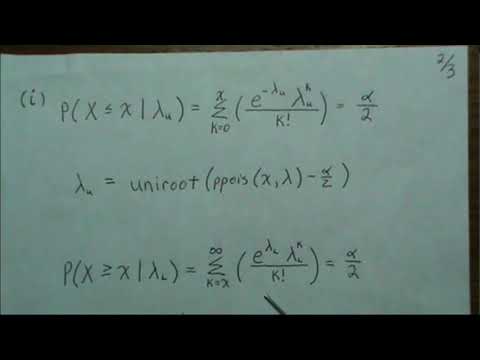 0:13:18
0:13:18
 0:05:47
0:05:47
 0:18:57
0:18:57
 0:00:41
0:00:41
 0:09:36
0:09:36
 0:48:46
0:48:46
 0:25:33
0:25:33
 0:19:42
0:19:42
 0:21:02
0:21:02
 0:59:01
0:59:01
 0:07:24
0:07:24
 0:08:34
0:08:34
 0:05:07
0:05:07
 0:10:53
0:10:53
 0:45:07
0:45:07
 0:04:56
0:04:56