Knot (mathematics) | Wikipedia audio article

This is an audio version of the Wikipedia Article:


00:00:39 1 Formal definition
00:01:18 1.1 Projection
00:01:38 2 Types of knots
00:02:17 2.1 Tame vs. wild knots
00:02:36 2.2 Framed knot
00:03:16 2.3 Knot complement
00:03:35 2.4 JSJ decomposition
00:04:14 3 Applications to Graph Theory
00:04:54 3.1 Medial graph
00:05:33 3.2 Linkless and knotless embedding
00:06:12 4 Generalization
00:06:51 5 See also
00:07:30 6 Notes
00:07:50 7 References
00:08:29 8 External links
00:09:09 Generalization
00:09:48 S1 and M
00:10:27 0. n − j is called the codimension of the knot. An interesting aspect of Haefliger's work is that the isotopy classes of embeddings of S j in Sn form a group, with group operation given by the connect sum, provided the co-dimension is greater than two. Haefliger based his work on Stephen Smale's h-cobordism theorem. One of Smale's theorems is that when one deals with knots in co-dimension greater than two, even inequivalent knots have diffeomorphic complements. This gives the subject a different flavour than co-dimension 2 knot theory. If one allows topological or PL-isotopies, Christopher Zeeman proved that spheres do not knot when the co-dimension is greater than 2. See a generalization to manifolds.
00:11:06 See also



Listening is a more natural way of learning, when compared to reading. Written language only began at around 3200 BC, but spoken language has existed long ago.

Learning by listening is a great way to:
- increases imagination and understanding
- improves your listening skills
- improves your own spoken accent
- learn while on the move
- reduce eye strain

Now learn the vast amount of general knowledge available on Wikipedia through audio (audio article). You could even learn subconsciously by playing the audio while you are sleeping! If you are planning to listen a lot, you could try using a bone conduction headphone, or a standard speaker instead of an earphone.

Listen on Google Assistant through Extra Audio:
Other Wikipedia audio articles at:
Upload your own Wikipedia articles through:
Speaking Rate: 0.8050302084346294
Voice name: en-US-Wavenet-E


"I cannot teach anybody anything, I can only make them think."
- Socrates


SUMMARY
=======
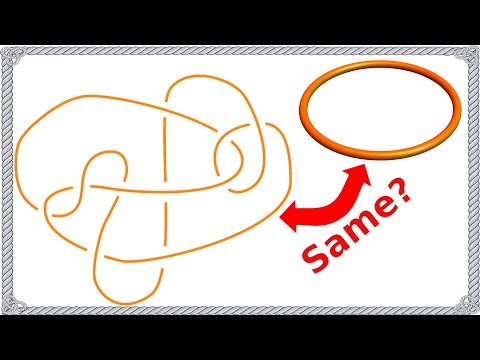
In mathematics, a knot is an embedding of a circle S1 in 3-dimensional Euclidean space, R3 (also known as E3), considered up to continuous deformations (isotopies). A crucial difference between the standard mathematical and conventional notions of a knot is that mathematical knots are closed—there are no ends to tie or untie on a mathematical knot. Physical properties such as friction and thickness also do not apply, although there are mathematical definitions of a knot that take such properties into account. The term knot is also applied to embeddings of S j in Sn, especially in the case j = n − 2. The branch of mathematics that studies knots is known as knot theory, and has many simple relations to graph theory.