filmov
tv
Derivatives the Easy Way in Calculus

Показать описание
In calculus, a derivative measures the rate at which a function changes. It provides a formula for the slope of a curve at any given point, representing instantaneous rate of change. Calculated as the limit of the average rate of change as the interval approaches zero, derivatives offer insights into functions' behavior, critical points, and concavity. They play a pivotal role in optimization, physics, and understanding dynamic processes. The process of differentiation involves finding the derivative, crucial for solving problems related to motion, growth, and optimization, making it a foundational concept in calculus with widespread applications in various fields.
Derivatives the Easy Way in Calculus
Derivatives Explained in One Minute
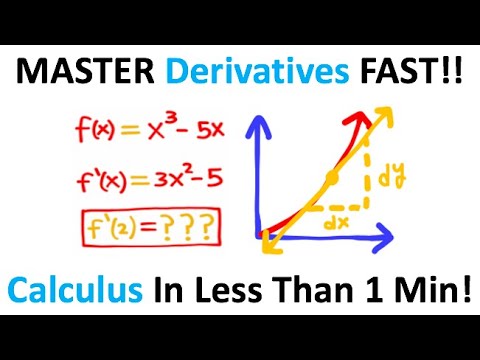
MASTER Derivatives In Less Than A Minute!!
Calculus 1 - Derivatives
Easy Way to Remember Derivatives of Trigonometry Ratios #shorts | How to Remember Derivatives Easily
Derivatives for Beginners - Basic Introduction
Derivative as a concept | Derivatives introduction | AP Calculus AB | Khan Academy
Understand Calculus in 35 Minutes
a curious case of a densely discontinuous derivative
Learn Derivatives Fast | Ultimate Beginner's Guide To Derivatives
Derivatives... How? (NancyPi)
Why is calculus so ... EASY ?
What are Derivatives? Derivatives Kya Hote Hai? Simple Explanation in Hindi #TrueInvesting
What is Jacobian? | The right way of thinking derivatives and integrals
Understanding the Basics of Derivatives in Calculus
100 calculus derivatives (ultimate derivative tutorial)
Differentiation | Derivatives (General Method)
Learn Derivatives in Calculus
Derivatives l Formulae & Basic Sums | HSC (12th) Science, Commerce & Arts
Derivative Trick for Quotient Rule (Derivatives Calculus)
How To Remember The Derivatives Of Trig Functions
Derivatives Market For Beginners | Edelweiss Wealth Management
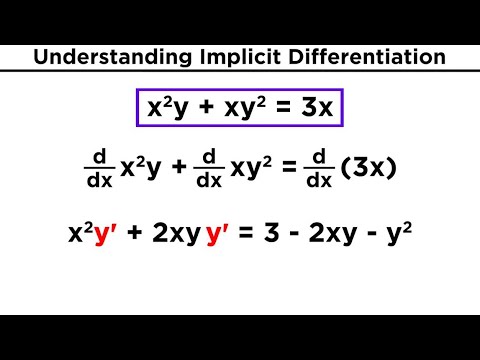
Implicit Differentiation
Definition of the Derivative
Комментарии
 0:00:59
0:00:59
 0:01:30
0:01:30
 0:00:58
0:00:58
 0:52:51
0:52:51
 0:00:50
0:00:50
 0:58:04
0:58:04
 0:07:16
0:07:16
 0:36:22
0:36:22
 0:11:08
0:11:08
 0:23:58
0:23:58
 0:14:30
0:14:30
 0:38:32
0:38:32
 0:03:34
0:03:34
 0:27:14
0:27:14
 0:01:00
0:01:00
 6:38:13
6:38:13
 0:13:33
0:13:33
 0:00:59
0:00:59
 0:11:20
0:11:20
 0:05:18
0:05:18
 0:05:45
0:05:45
 0:06:01
0:06:01
 0:11:45
0:11:45
 0:23:31
0:23:31