filmov
tv
Using Abel's Test to Prove a Fourier Sine Series Converges

Показать описание
We use Abel's Test for infinite series to prove that a particular Fourier Sine series converges. The terms sin(kx) have bounded partial sums away from the origin and 2\pi and the terms 1/k monotonically decrease to zero. These conditions allow us to prove that the infinite series converges.
#mikethemathematician, #mikedabkowski, #profdabkowski
#mikethemathematician, #mikedabkowski, #profdabkowski
 0:06:41
0:06:41
 0:17:15
0:17:15
 0:07:28
0:07:28
 0:00:09
0:00:09
 0:01:09
0:01:09
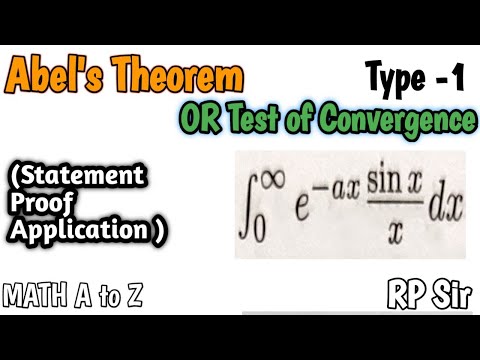 0:11:45
0:11:45
 0:10:25
0:10:25
 0:00:06
0:00:06
 0:39:12
0:39:12
 0:01:39
0:01:39
 0:08:18
0:08:18
 0:03:52
0:03:52
 0:32:27
0:32:27
 0:00:15
0:00:15
 0:00:13
0:00:13
 0:17:36
0:17:36
 0:17:03
0:17:03
 0:07:02
0:07:02
 0:15:41
0:15:41
 0:10:15
0:10:15
 0:08:04
0:08:04
 0:19:30
0:19:30
 0:04:13
0:04:13
 0:10:45
0:10:45