filmov
tv
AQA A-Level Further Maths D9-01 Inequalities: Introducing Rational Function Inequalities

Показать описание
AQA A-Level Further Maths D9-01 Inequalities: Introducing Rational Function Inequalities
AQA A-Level Further Maths D9-02 Inequalities: Rational Function Example 1
AQA Further Pure 1 (FP1) 10.07 Solving an Inequality
AQA A-Level Further Maths D10-01 Modulus: Introducing y = |f(x)|
AQA A-Level Further Maths E9-01 Limits: Limit of x^k e^(-x) as x tends to ∞
AQA A-Level Further Maths D9-05 Inequalities: A More Complicated Example
AQA A-Level Further Maths D16-01 Transformations: Sketch y^2 = 4x - 12
AQA A-Level Further Maths D8-01 Polynomial Inequalities: Introducing Cubic Inequalities
A-Level Further Maths E1-06 Improper Integrals: Integrating 1/√x from 0 to 1
A-Level Further Maths E1-10 Improper Integrals: Integrating 1/(x-1)^5 from 2 to ∞
AQA A-Level Further Maths D9-04 Inequalities: Rational Function Example 3
AQA A-Level Further Maths D9-03 Inequalities: Rational Function Example 2
AQA A-Level Further Maths D12-12 Rational Functions: Sketching y = (1-5x)/(5x-13) & y = 2x-1
AQA A-Level Further Maths D12-06 Rational Functions: Sketching y = (4-5x)/(2x+1)
AQA A-Level Further Maths D12-02 Rational Functions: Sketching y = (x+1)/(x-1)
AQA A-Level Further Maths C10-02 Eigenvalues and Eigenvectors: Formalising Notation & Method
[A Level Further Math] Rational Functions and Graphs | Part 2 | Further Pure Mathematics 1
AQA A-Level Further Maths D13-01 Rational Functions: How to Sketch y=(ax^2+bx+c)/(dx^2+ex+f)
AQA A-Level Further Maths D13-17 Rational Functions: Sketching y = (4x^2-5)/(3x+1)
AQA A-Level Further Maths D16-10 Transformations: Sketch (x-1)^2 - y^2 = 1
A-Level Further Maths E1-02 Improper Integrals: Integrating x^2 from 1 to ∞
AQA A-Level Further Maths D13-10 Rational Functions: Sketching y = 1/(x^2+4x-45)
AQA A-Level Further Maths D12-10 Rational Functions: Sketching y = (x+1)/(x+2) & y = x+3
AQA A-Level Further Maths D10-02 Modulus: Solving an Equation Example 1
Комментарии
 0:08:51
0:08:51
 0:04:32
0:04:32
 0:04:11
0:04:11
 0:04:28
0:04:28
 0:08:13
0:08:13
 0:13:50
0:13:50
 0:02:32
0:02:32
 0:05:07
0:05:07
 0:01:37
0:01:37
 0:03:24
0:03:24
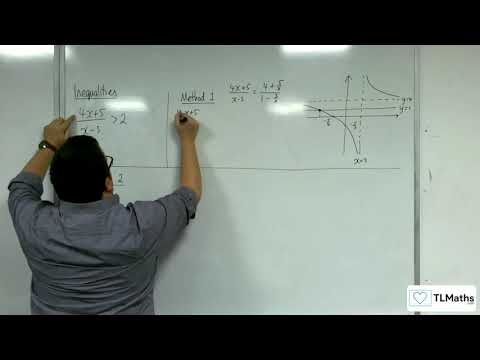 0:04:22
0:04:22
 0:05:05
0:05:05
 0:04:49
0:04:49
 0:02:01
0:02:01
 0:02:30
0:02:30
 0:12:35
0:12:35
 0:16:38
0:16:38
 0:17:28
0:17:28
 0:05:14
0:05:14
 0:03:59
0:03:59
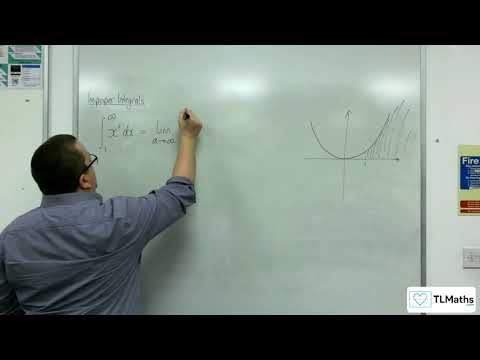 0:05:20
0:05:20
 0:03:06
0:03:06
 0:04:25
0:04:25
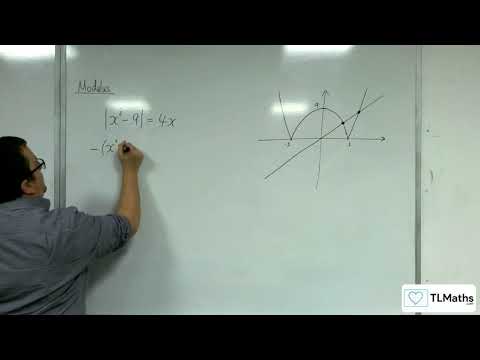 0:05:45
0:05:45