Linear growth of the quantum circuit complexity of random quantum circuits

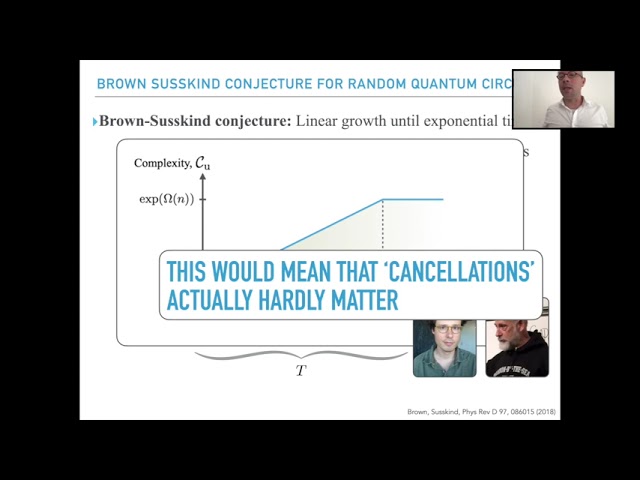
This is a rerecorded brief talk on the linear growth of quantum circuit complexity in random quantum circuits held at "Quantum Complexity: Theory and Application" in Dagstuhl on June 30, 2021, a fun workshop help partially on-site and partially with an off-site audience.

[AritraSarkar_YT]

~17mins... Brown-Susskind conjecture is kinda Poincare recurrence time in Hilbert phase space?
~last slide... why worry about Nielsen cost when Brown-Susskind poses a refinement of it (with a term involving the Quantum Kolmogorov Compleity as a correspondence with the potential energy)?
~last slide... given that Entanglement is an alternative to world-making relation like space-time distance metrics, a growth rate associate to it might help in quantum gravity.

excellent presentation by the way! Happy to see that you picked the right 'Really Big Questions'.. ;)